Aren't these equations identical? What is the difference in the aforementioned "time-harmonic version"?

Question

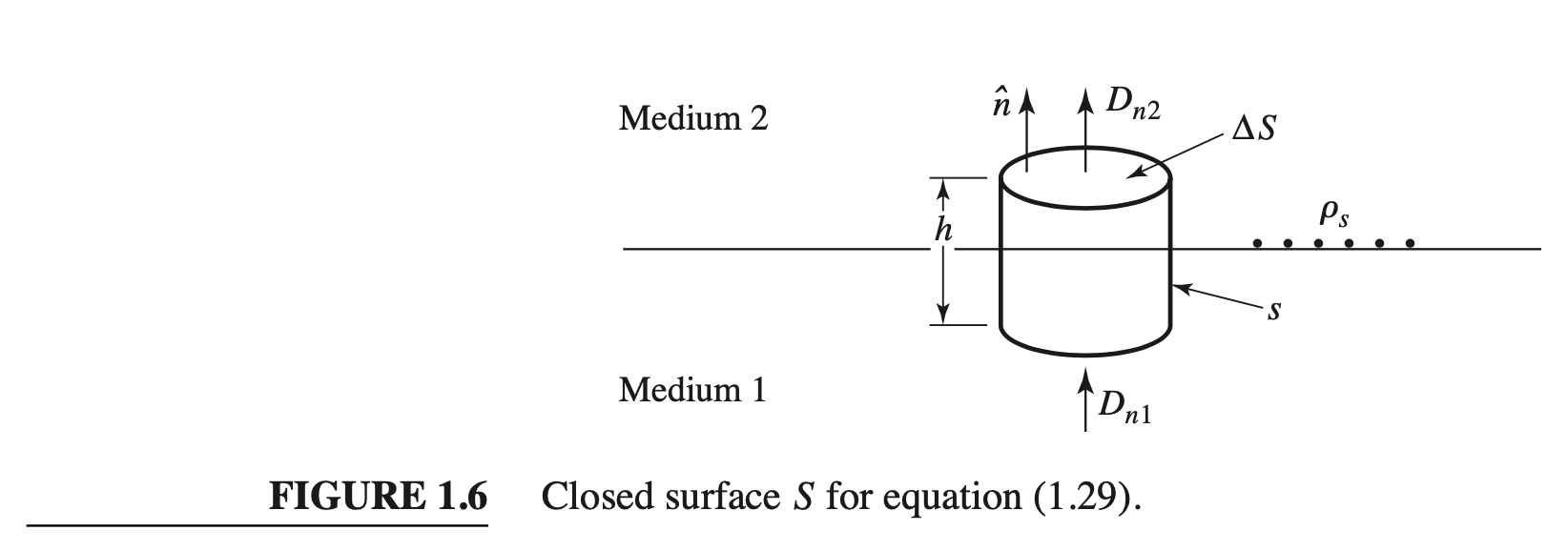
I am currently studying the textbook Microwave Engineering, fourth edition, by David Pozar. Section Fields at a General Material Interface of chapter 1.3 FIELDS IN MEDIA AND BOUNDARY CONDITIONS says the following:

The time-harmonic version of (1.4), where $S$ is the closed "pillbox"-shaped surface shown in Figure 1.6, can be written as $$\oint_S \bar{D} \cdot d \bar{s} = \int_V \rho \ dv. \tag{1.29}$$

(1.4) is written as follows:

$$\oint_S \bar{D} \cdot d \bar{s} = \int_V \rho \ dv = Q \tag{1.4}$$

$\bar{D}$ is the electric flux density, in coulombs per meter squared (Coul/$\text{m}^2$), $\rho$ is the electric charge density, in coulombs per meter cubed (Coul/$\text{m}^3$), and $Q$ is the total charge contained in the closed volume $V$.

So aren't (1.29) and (1.4) identical? What is the difference in the aforementioned "time-harmonic version"?

Answer 1

If you look carefully, Eq. 1.4 has a calligraphic symbol $\bar{\mathcal{D}}$, while Eq. 1.29 has a regular $\bar{D}$. They are related by factoring out a harmonic time dependence $\bar{\mathcal{D}}=\Re{[e^{j\omega t} \bar{D}]}$ which in the book is called "phasor notation". This is mostly useful when assuming the time dependence of the fields is purely harmonic with other properties being in a steady state. Thus they call it "time harmonic version".