What is this difference in the notation for the electric flux density in this closed surface example?

Question

I am currently studying the textbook Microwave Engineering, fourth edition, by David Pozar. Section Fields at a General Material Interface of chapter 1.3 FIELDS IN MEDIA AND BOUNDARY CONDITIONS says the following:

Fields at a General Material Interface
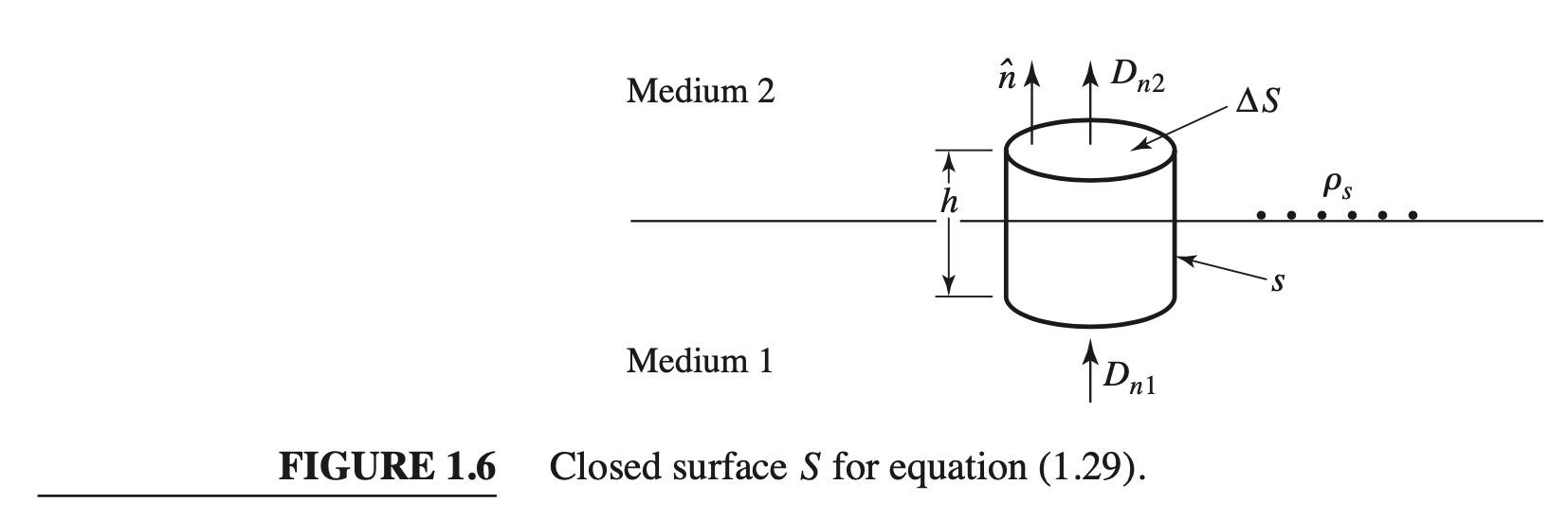
Consider a plane interface between two media, as shown in Figure 1.5. Maxwell's equations in integral form can be used to deduce conditions involving the normal and tangential fields at this interface. The time-harmonic version of (1.4), where $S$ is the closed "pillbox"-shaped surface shown in Figure 1.6, can be written as $$\oint_S \bar{D} \cdot d\bar{s} = \int_V \rho \ dv. \tag{1.29}$$ In the limit as $h \to 0$, the contribution of $D_\text{tan}$ through the sidewalls goes to zero, so (1.29) reduces to $$\Delta SD_{2n} - \Delta SD_{1n} = \Delta S \rho_s,$$ or $$D_{2n} - D_{1n} = \rho_s, \tag{1.30}$$ where $\rho_s$ is the surface charge density on the interface.

$\bar{D}$ is the electric flux density, in coulombs per meter squared (Coul/$\text{m}^2$).

Notice that, in Figure 1.5 and Figure 1.6, we are given $D_{n1}$ and $D_{n2}$, but, in equations (1.30), we are given $D_{1n}$ and $D_{2n}$. Why is there this difference and what does it mean?

Answer 1

It looks to me like this was a mistake by the author or publisher of the textbook. The $n$ in the subscript refers to the normal component of the field, and $1$ or $2$ refers to which material the field is in. Either order--$n1$ or $1n$--means the same thing, but the editor should have made sure the same notation was used throughout.