0
$\begingroup$

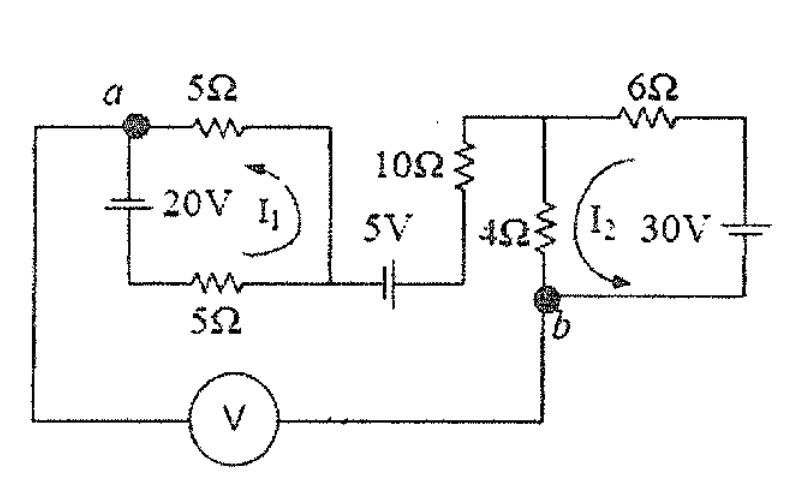
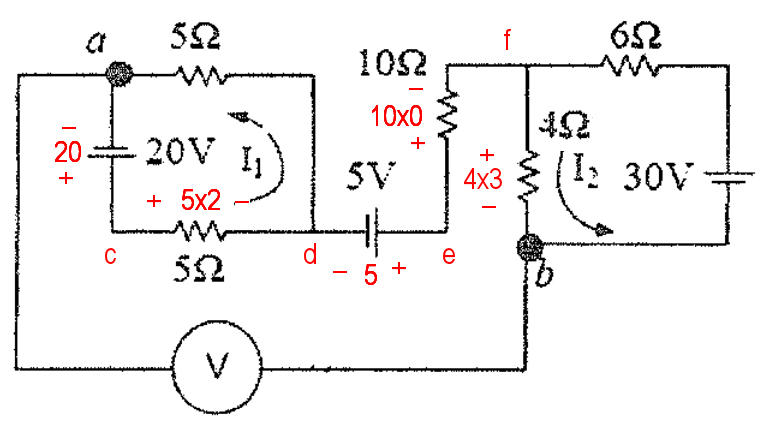
what will be the potential difference between point a and b?

I have been trying to solve this problem for a long time but couldn't. It has been suggested to solve this problem considering the voltmeter to be ideal so no current flows through the 10ohm resistor. If that is the case then $I_2 =3A $ and $I_1=2A$ and potential at the junction between 10ohm resistor wire and right side loop is 15V. If we solve that way then we get a non zero potential at point b. Is that even possible that there is a non negative potential at a point of the circuit which is directly connected to the negative terminal of the cell and there is no resistor in between as well as the wires are resistanceless?

It will be appreciated highly if someone clears this confusion of mine.

enter image description here

Improve this question
$\endgroup$
7
  • $\begingroup$ Voltmeters in schematics are generally assumed to be ideal (and it does not really matter in this case since the resistance of a voltmeter is generally in the MOhms while we're looking at way lower ohic values here). This looks an awful lot like homework assignement. Is it? Than please show the effort you've already put into solving this (like redrawing the schematic so it is easier to read). Hint: Put "b" at the bottom and "a" at the top and make all components vertical. $\endgroup$
    – kruemi
    Commented Feb 8, 2022 at 6:57
  • $\begingroup$ You can in principle choose any point in a circuit and say that point is 0 volts. If you choose the most negative point then all your voltages will be positive. But wouldn't $I_2=30/10=3A$? $\endgroup$
    – Peter
    Commented Feb 8, 2022 at 6:58
  • $\begingroup$ And "negative potential" and "positive potential" is always dependent on what you assume to be zero. A voltmeter always shows the difference between to points, so sure, b can have a negative potential towards whatever point you assume is zero. $\endgroup$
    – kruemi
    Commented Feb 8, 2022 at 7:02
  • $\begingroup$ @Peter I corrected it now. Thanks for pointing out that $\endgroup$
    – MSKB
    Commented Feb 8, 2022 at 7:32
  • 1
    $\begingroup$ You can’t talk about the potential at point b unless some point in the circuit is assigned a potential of zero $\endgroup$
    – Bob D
    Commented Feb 8, 2022 at 8:21

2 Answers 2

Reset to default
2
$\begingroup$

You should not talk about the potential of a node unless you compare with the potential of another node in the circuit.

I will use the notation $V_{\rm ab}$ as meaning the potential of node $b$ relative to node $a$.

The current through a resistor always flows from a region of higher potential to a region of lower potential.

I have added some labels to your circuit.

enter image description here

In your circuit $V_{\rm ab}=V_{\rm ac}+V_{\rm cd}+V_{\rm de}+V_{\rm ef}+V_{\rm fb}$ which is equivalent to starting a walk at node $a$ and passing through nodes $c,\,d,\,e,\,f,$ and arriving at node $b$ whilst added the potential differences between nodes together.

$V_{\rm ac} = +20$
$V_{\rm cd} = -5 \times 2 = -10$ - remember that the current through a resistor flows from a node at higher potential to a node at lower potential.
$V_{\rm de} = +5$
$V_{\rm ef} = 10 \times 0 = 0$
$V_{\rm ac} = -4\times 3 = -12$

Which gives $V_{\rm ab}= +20 +(-10)+5+(-0)+(-12)=+3\,\rm V$ ie the potential of node $b$ is $3\,\rm V$ higher than the potential of node $a$.

Improve this answer
$\endgroup$
1
  • $\begingroup$ previously I had a misconception about that. Thanks a lot $\endgroup$
    – MSKB
    Commented Feb 8, 2022 at 9:15
1
$\begingroup$

So basically we have 3 circuits in series. The one with I1 where we have 20V on 2 similar resistors. So from point "a" to the connection we have a 10V voltage differential (the "output" of this part is a + 10V). Than we have to 5V source and the 10 Ohm resistor. Forget the 10 Ohm, we have no current flowing trough it. So another 5. We're now 15V below "a" at this point. In the circuit with I2 we have a 30V source (attention, reversed direction!) and a 6 Ohm and a 4 Ohm resistor. We only care about the voltage drop on the 4 Ohm Resistor which is $30V * 4 Ohm / 6 Ohm$ so we're getting 12V on that one. But point b is 12V below the input of that part of the cicuit. So we have from a to b $10V + 5V - 12V = 3V$. So b is 3V higher than a.

No issue there.

Improve this answer
$\endgroup$
2
  • $\begingroup$ if we just focus on the rightmost loop, then potential drop across 6ohm was supposed to be 18V and so potential at that terminal should be 30V-18V=12V then we have 15V at the junction. As current cannot flow back to positive terminal of 30V source due to higher potential than 15V, So it will flow towards negative terminal then we have a 12Vvoltage drop across 4ohm. which means we are remaining with 3V at b. as far as I know potental at point b should be zero as a portion of the electrons goes to a reference potential region which is 0V and there are no resistance to drop some potential. $\endgroup$
    – MSKB
    Commented Feb 8, 2022 at 7:31
  • $\begingroup$ I am confused at potential at point b being 3V. $\endgroup$
    – MSKB
    Commented Feb 8, 2022 at 7:32

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.