0
$\begingroup$

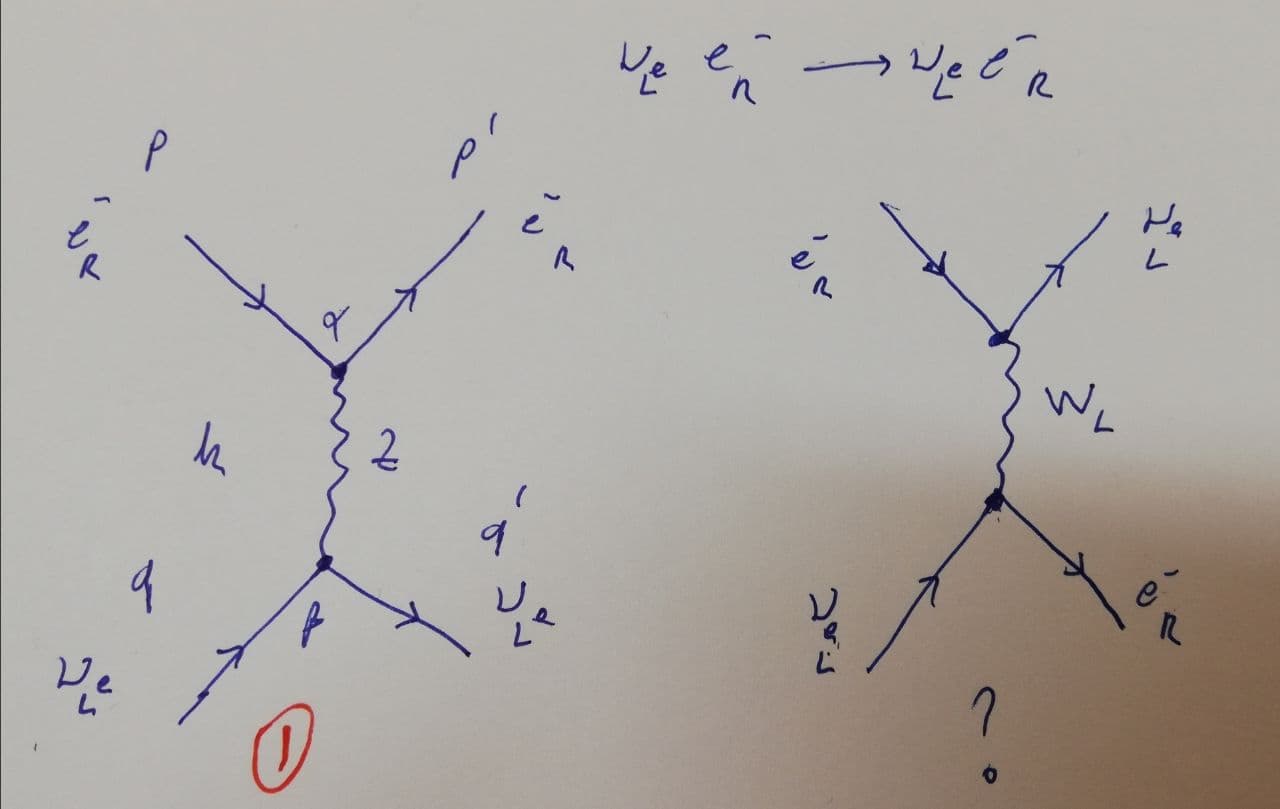
enter image description here This is a question about the Feynman diagrams: imagine I want to calculate the following process:

$$\nu_{e, L} + e^-_R \rightarrow \nu_{e, L} + e^-_R$$

  • I can have a t-channel diagram with $e^-_R e^-_R$ and $\nu_{e, L} \nu_{e, L}$ currents with an intermediate Z boson.

  • However I seem to have a process with $e^-_R \nu_{e, L}$ and $\nu_{e, L} e^-_R $ current terms with an intermediate W boson.

I am just not sure about the second diagram described above because it seems like handedness through the current terms in the diagram is not conserved.

My question simply is that does the handedness have to be conserved in the current terms of Feynman diagrams? Can the second process exist?

Edit To clarify what I am asking, a diagram has been added.

Improve this question
$\endgroup$
3
  • $\begingroup$ The R electron does not couple to the W--it is not part of the charged weak current. But it does couple to the Z, which "sees" both chiralities of leptons because of its peculiar "cockeyed" chiral structure. $\endgroup$
    – Cosmas Zachos
    Commented Jan 15, 2022 at 21:27
  • $\begingroup$ Could you please elaborate why R electron does not couple to W? (Is it something more general like no right handed fermion couples to W?) $\endgroup$
    – Monopole
    Commented Jan 15, 2022 at 21:31
  • $\begingroup$ Wouldn't such a coupling between different chiral particles and any gauge boson vanish, since the chiral projectors kill the term? I.e $\overline{\psi}_R\gamma^\mu\psi_L = \overline{\psi}P_L\gamma^\mu P_L\psi = \overline{\psi}P_LP_R\gamma^\mu\psi = 0$ ? $\endgroup$
    – QuantonPhysics
    Commented Nov 14 at 17:09

1 Answer 1

Reset to default
2
$\begingroup$

Indeed, all vector boson couplings preserve chirality. Moreover,

  • No R fermion and no L antifermion couples to the W.

  • By contrast, even though no neutral R fermion couples to the Z, charged fermions get an exemption: both L and R couple to the Z, because of the latter's "cockeyed" chiral structure. (Feynman's term, not mine.)

These are summarized in eqn. (10.2) of the PDG.

So your first diagram exists, but the second does not.

(Bonus fact: Accidentally, because $\sin^2 \theta_W \approx 0.22$, it turns out the vector coupling of the charged leptons is negligible, $g_V\approx -0.06$, so these couple to Z almost purely axially: the Z loves R electrons as much as the L ones.)

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.