Whenever I see a 2D drawing of dispersion occurring when light travels through a solid prism, I see the rays get bent downwards on entry and downwards on exit again. For example here: https://www.wikiwand.com/en/Dispersion_(optics)
To my understanding of optics when entering a medium with a higher optical density, the ray should get bent towards the normal of the surface, rotated CW and CCW when entering one with a lower IOR. However, the drawings suggest that it gets bent in the same direction upon entry and exit.
$\begingroup$
$\endgroup$
0
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
2
The normals in consideration for the incident and emergent rays are different.
For simplicity, take a monochromatic beam of light incident on a prism, as shown in this figure:
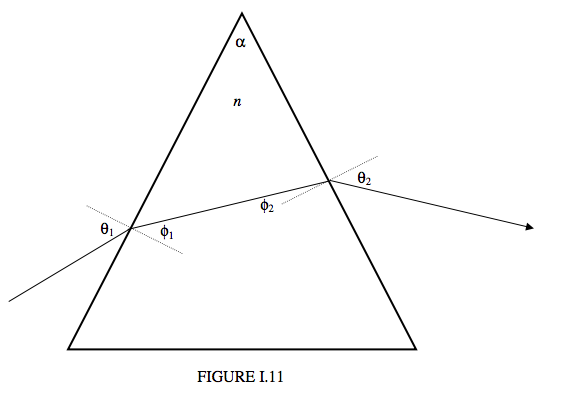
- When light is incident on a medium with a higher index of refraction ($n$), it bends towards the normal.
- When light is incident on a medium with a lower $n$ it bends away from the normal.
In reference to this figure, the incident ray should bend towards the normal, which would mean a clockwise rotation ($\phi_1 < \theta_1$)
And the ray within the prism would bend away from the new normal at the new interface, corresponding to another clockwise rotation. ($\phi_2 < \theta_2$)
For a beam of light, dispersion will cause different wavelengths of light to bend in different angles, but they will all bend in the same sense.
Hope this helps.
-
4$\begingroup$ The first sentence says it all, thanks for the well formated answer, mystery solved. $\endgroup$ Commented Dec 21, 2021 at 17:39
-
3$\begingroup$ There's a similar phenomenon with lenses: both sides bend the light, and they do it the same way because the slopes are reversed. $\endgroup$ Commented Dec 22, 2021 at 6:59
$\begingroup$
$\endgroup$
4
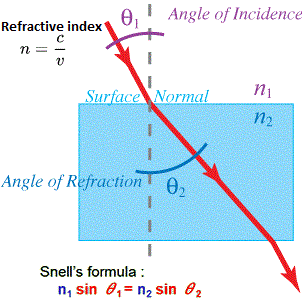
This is how refraction of light in a medium works.
The phase velocity $v$ of light changes transitioning from one medium to a different density medium according to its refraction index $n$ and the refraction angle to the incident is dictated by Snell's law:
Base image source: https://www.detailingwiki.org/detailing-miscellaneous/what-is-refractive-index/attachment/snellslaw1/
The light then exiting the medium and returning to the initial medium regains whatever phase velocity $v$ it had in this medium and therefore also to its initial incident angle.
If the boundary entry and exit surfaces of the medium are not parallel to each other, like the prism case, you have to algebraically add their difference to the exit angle. Snell's law does not change.
-
3$\begingroup$ I upvoted this answer bc. it's correct, but the other one is more detailed. $\endgroup$ Commented Dec 21, 2021 at 17:42
-
$\begingroup$ The blue medium in the middle has a lower index of refraction than the material outside (i.e. $n_2 < n_1; \theta_2 > \theta_1$) quite an unusual example and not a good one for the question which is (likely) about a glass prism in air. It's opposite of any of the other illustrations in either link. I'm curious, where is it from, here perhaps? $\endgroup$– uhohCommented Dec 24, 2021 at 0:06
-
1$\begingroup$ @uhoh Yes this is true. However, the mechanism and conclusions remain the same with the only difference that a denser $n_{2}$ medium would bend light westward of the $n_{1}$ direction shown instead of eastward as shown in the above illustration. $\endgroup$ Commented Feb 2, 2022 at 16:03
-
$\begingroup$ Okay, thanks for the reply. I have a hunch that the original drawing was actually drawn in error with the darker material having substantially lower index, but you are right, it certainly doesn't make any difference to your answer. +1 $\endgroup$– uhohCommented Feb 2, 2022 at 19:52