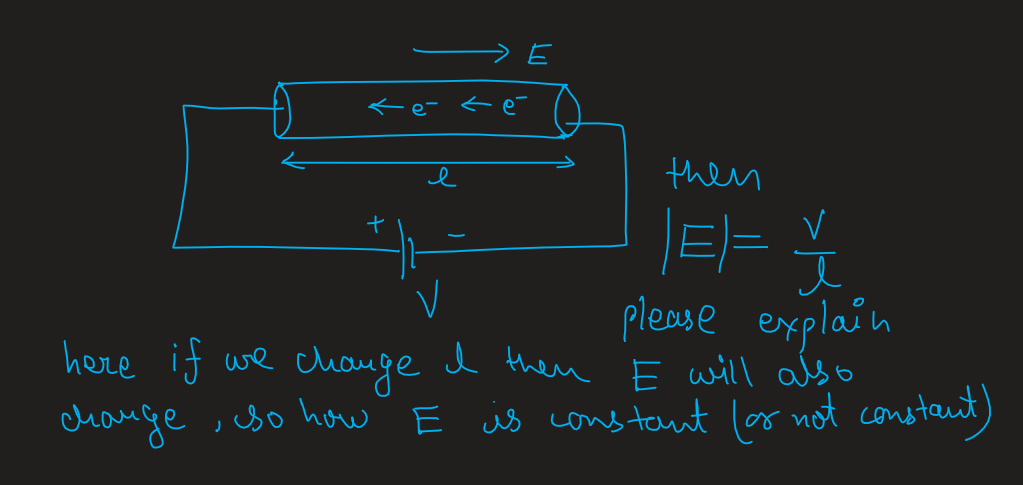For a uniform (constant) electric field, we have the relation $E = - \Delta V/\Delta r$. Now, if the electric field provided by a battery is constant over a constant potential difference and if we calculate the field between two points on a wire taking the same value of $\Delta V$ (as of battery), the electric field will increase as we reduce the distance between the points on the wire, which contradicts the field being constant throughout the wire? Please explain.