1
$\begingroup$

I've run a lot of laser diffraction experiments with nothing more than a quality laser pen, some rasor blades, a human hair and a small hole in a Christmas card as demonstrations for laymen but I'm kind of bored with these now.

I'd really like to emulate X-ray crystallography diffraction, in a backyard lab setting but found it hard to get (Google) some information on that.

One possibility is to construct a macroscopic $\text{NaCl}$-style lattice from copper single filaments, with a $d$ of about $0.1\,\mathrm{mm}$. That would of course not be easy to pull off but not impossible either, IMO.

I do have another idea, which I want to keep 'under wraps' for now because I'll be test driving it shortly.

Searching P.SE for 'laser diffraction' I came across one intriguing comment claiming $\text{XRD}$-style laser diffraction may be possible with synthetic Opal. Googling then threw up large amounts of links to applications of laser diffraction in the field of particle characterisations (something I'm not interested in for now).

And then there's a paper titled Linear and Nonlinear Optics of Synthetic Opal by M. V. Vasnetsov, V. Yu. Bazhenov, V. V. Ponevchinsky, which throws a little light on the structure of (a) synthetic Opal: 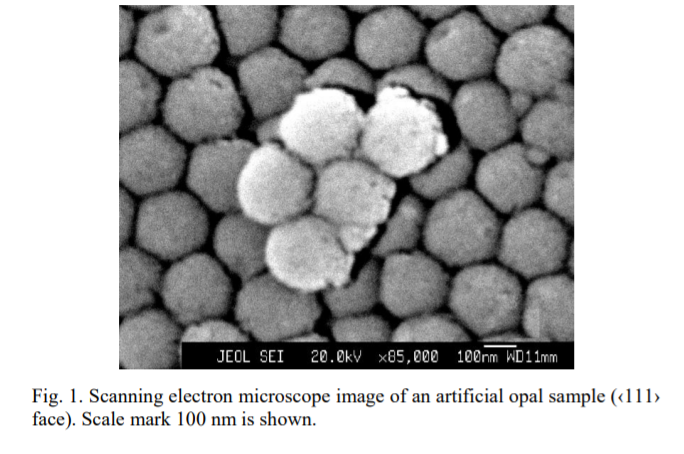the structure of one synthetic Opal

But with a lattice $d \approx 100\,\mathrm{nm}$ it isn't clear how such a material could exhibit 'laser $\text{XRD}$'.

Commercial synthetic Opals are not expensive and readily available but the samples I've seen are all either non-transparent or transparent like glass, so it's hard to see how they could produce the effect I'm looking for.

A beautiful (commercial) sample of synthetic Opal (about $8\,\mathrm{g}$):

Opal

The fascinating coulours of Opals are attributed to diffraction.

Does anyone here have experience/information on laser $\text{XRD}$ with Opal or anyother substrates?

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ You should take a look at a book called "Atlas of Optical Transforms" by Harburn, Taylor and Welberry. $\endgroup$
    – Xcheckr
    Commented Nov 24, 2021 at 16:39
  • 1
    $\begingroup$ Perhaps use a printer to print small black dots on a transparency and stack layers of small pieces of that to mimic a crystal structure, e.g., NaCl. Just a thought. $\endgroup$
    – Ed V
    Commented Nov 24, 2021 at 16:57
  • $\begingroup$ @EdV Yup: like that idea! $\endgroup$
    – Gert
    Commented Nov 24, 2021 at 17:22

1 Answer 1

Reset to default
2
$\begingroup$

I cannot give you information about Opals but only last week I came across a rainbow coloured Happy Birthday banner as shown below.

enter image description here

I flattened one corner with a couple of microscope slides and a paper clamp.

enter image description here

Using a couple of holders (as I do not a third hand to take the photograph I was able to get a 2-D diffractions pattern from which I was able to evaluate the "crossed" grating line spacing.

enter image description here

Improve this answer
$\endgroup$
11
  • $\begingroup$ Hey, that's wonderful! So you shone the laser (a pointer pen?) straight (perpendicularly) on the material? What gave you the idea? $\endgroup$
    – Gert
    Commented Nov 24, 2021 at 17:25
  • 1
    $\begingroup$ I wondered about the colouration of the banner which was characteristic of a diffraction/interference pattern. Shifting colours as the banner or my eyes moved. $\endgroup$
    – Farcher
    Commented Nov 24, 2021 at 18:26
  • $\begingroup$ Yes, of course. For that reason Opal should also be useable for 'laser $\text{XRF}$' because its fleeting colours are due also to diffraction. Time to buy! $\endgroup$
    – Gert
    Commented Nov 24, 2021 at 18:56
  • $\begingroup$ I've also bought a similar Happy Birthday banner with those interesting 'ecanescent' colours, so time to experiment. $\endgroup$
    – Gert
    Commented Nov 24, 2021 at 18:59
  • $\begingroup$ What laser did you use? With my $532\,\mathrm{nm}$ green laser I'm getting very variable results but nothing as definite as your result. Interestingly my red $650\,\mathrm{nm}$ laser shows $zero$ diffraction, indicating the "$d$" must be smaller than that value. $\endgroup$
    – Gert
    Commented Nov 26, 2021 at 15:52

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.