Bragg diffraction and lattice planes

Question

Crystalline substances show, for certain sharply defined wavelength and incident directions, very sharp peaks of scattered X-ray radiation.

From the illustration below we see that we get constructive interference when the path-length difference is a multiple of the wavelength $\lambda$.

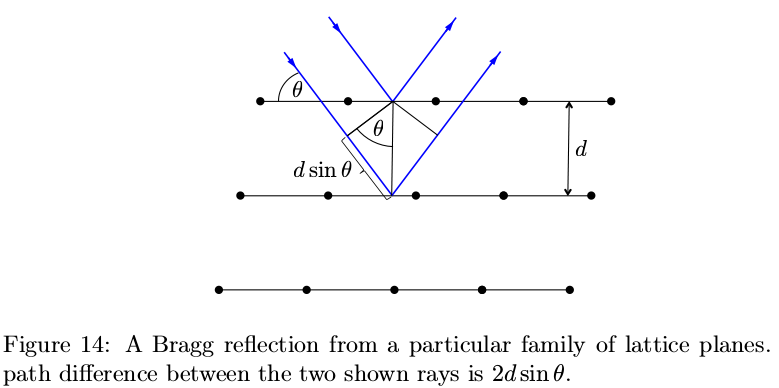
In real crystalline materials we have a large amount of closely packed lattice planes. This large amount accounts for the sharp peaks for certain $\theta$. I do not understand how this follows from the Bragg reflection formula $$ n\lambda = 2d \sin \theta , $$ since $d$ is not constant anymore. I understand the model for two lattice planes as in the illustration.

Is it true that $d$ can only take on values of the seperation of lattice planes, so $d$ is defined to be the seperation of points in the reciprocal lattice, or in others words, is $d$ constrained to be the absolute values of some reciprocal lattice vector?

How does the Bragg condition account for very sharp peaks when we let $d$ run through all such absolute values?

Answer 1

The d is not separation between points in reciprocal lattice. Actually, they do not even have the same units. d is the separation between lattice planes, as you said. What is related to reciprocal lattice vectors is the change (before and after scattering) in the wave vector of light: change in k = reciprocal lattice vector, which is the Laue condition that is equivalent to Bragg condition. See e.g. the book by Ashcroft and Mermin.

Answer 2

I think that the most appropriate way to think about the Bragg formula is in terms of a diffraction grating. In a diffraction grating one obtains sharp peaks because there are many slits with distance $d$ between them. The derivation of the intensity maximum for the diffraction grating case is similar to the Bragg case. To obtain a diffraction grating maximum, i.e. constructive interference, the path-length difference between each of the slits, $d\sin\theta$, must be an integer number of wavelengths, $n\lambda$.

Similarly, for the Bragg case, one must now have that the path-length difference between each of the lattice planes, $2d\sin\theta$ (because the light reflects into and out of the material), must be an integer number of wavelengths, $n\lambda$. This is the condition for constructive interference, and hence one gets sharp maxima just like in the diffraction grating case.

Hence, one may in some sense think of a perfect crystal as a diffraction grating for X-rays.

Answer 3

$d$ is constrained to be integer multiples of any ($hkl$) planar separation. The use of Miller Indices really helps realise how many planes there can be to reflect off, especially when it comes to more complex crystal structures. An alternate representation of Braggs law is $n \lambda = 2d_{hkl}\sin\theta$ to explicitly show which crystal plane is satisfying the Bragg condition. You can also define it in terms of the reciprocal space, where the Bragg condition will be satisfied if the change in the wavevector from scattering is equal to a reciprocal lattice vector associated with the crystal structure of the material, $\Delta \mathbf{k} = \mathbf{G}$.

Answer 4

The d in that formula is the distance between lattice planes, not points. Basically, one ray (the top one) bounces off the top "plane". The other ray (the bottom one) passes the first plane and "bounces" off the second plane. It then comes out and meets the first ray at some far away point.

Question: Since they travelled different distances, they have different phases. In other words, they will interfere. For this to happen, the "extra" distance the bottom ray traveled must be equal to a multiple of $ \lambda $ so that constructive interference occurs. What is the formula?

You can use some simple trig to show that the extra distance traveled by the bottom ray is $ 2dsin(\theta) $, and you want this to be a multiple of $ \lambda$, the wavelength of the light.

So: $ 2dsin(\theta) = n\lambda$ is the condition for constructive interference.