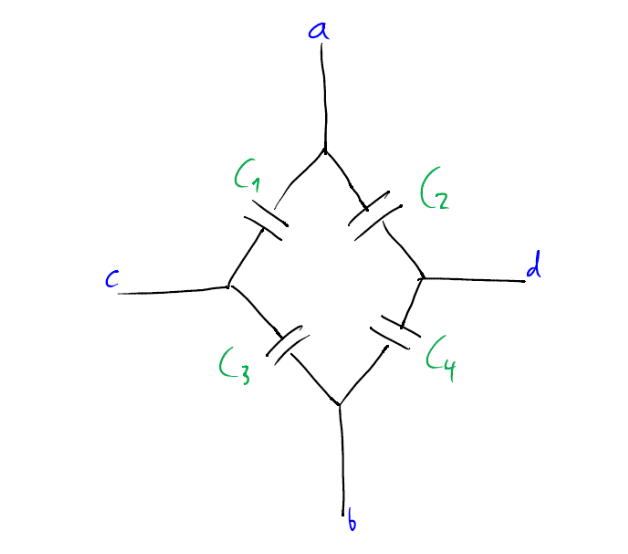
There is a rhombus with four different capacitors. There's a voltage between the point $a$ and the point $b$, but there is no voltage between the point $c$ and the point $d$. Apparently, assuming that there is no excess of charges in the circuit, the capacitors on the left side have the same charge, and the same holds for the right side. I have trouble understanding why the charges should be equal on each side.
3 Answers
It is true, only if the capacitors have capacitance values that are symmetric ( $C_1 = C_2$ and $C_3 = C_4$ ). Else is not true. You can do the computation using the formula of a series of capacitors $1/C_{tot} = 1/C_1 + 1/C_2 +...+ 1/C_n$ (here $n = 2$). You can compute the charge by the well known relationship $Q = C \times V$.
You should have trouble with it. It isn't true. For example, suppose $$C_3 = 2C_1$$ $$C_2 = 10C_1$$ $$C_4 = 20 C_1$$
You will find that
$$V_{ac} = V_{ad}$$
But
$$Q_{right} = 10 Q_{left}$$
In the above circuit $C_1$ and $C_3$ are in series and also parallel to series capacitors: $C_3$ and $C_4$. There is a voltage potential between $a$ and $b$, but none between $c$ and $d$. Capacitors in series have the same charge, and the charge on the left and right sides is the same.
For the series capacitors:
$$left \ hand \ side \ \ \frac{1}{C_{s1}} = \frac{1}{C_1} + \frac{1}{C_3} = \frac{C_3 + C_1}{C_1 C_3}$$
$$right \ hand \ side \ \ \frac{1}{C_{s2}} = \frac{1}{C_2} + \frac{1}{C_4} = \frac{C_4 + C_2}{C_2 C_4}$$
We add the capacitance on left and right sides that are in parallel. I.e. capacitance is added, because the charges are added but the voltage is the same:
$$C_p = C_{s1} + C_{s2}$$
Therefore, for the whole system:
$$Q_{tot} = C \times V = (C_{s1} + C_{s2}) \times V $$
But the charges on the left and right hand sides are the same, therefore, $C_{s1}$ and $C_{s2}$ must be the same. So, $C_1 = C_2$ and $C_3 = C_4$, or even, $C_1 = C_2 = C_3 = C_4$.
I.e. $Q_{rightside} = C_{s1} V = Q_{leftside} = C_{s2} V $