How does friction act on vehicles driving on the 'Well of Death'/'Motordrome'?

Question

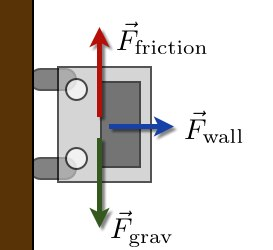
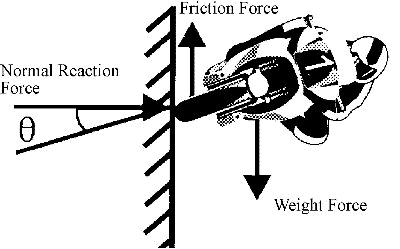
Since the body is moving in a circular path, I understand that the normal reaction from the wall provides the required centripetal force. I also get that the driver has to lean in order to counteract the rotating forces caused because the forces of gravity, friction and normal are in different planes. What I don't get is the action of friction. I read that when the driver goes faster, the upward friction force increases, counteracting gravity. How does that happen? In my understanding, when the driver goes faster, the required centripetal force increases.

My questions:

1. When the driver goes faster, does the normal reaction from the wall increase to provide the extra centripetal force? If he goes slower, does it decrease?

2. If yes,

   a. How?

   b. Is that increase in normal reaction responsible for increasing friction?

   c. Does the normal reaction keep increasing when velocity increases? Meaning, if the person keeps accelerating, will the normal reaction keep increasing to provide the centripetal force?

   d. If the person goes too slow, does he fall because normal reaction is too weak to provide enough friction?

3. If no, how exactly does it work?

Answer 1

1. Yes
2. This explanation is based on your first picture.

Since the body is moving in a circular path, I understand that the normal reaction from the wall provides the required centripetal force.

Yes, it's true. It is given by $\vec{F}_{\text{wall}}=\frac{mv^2}{r}$ . $v$ is the speed and $r$ is the radius of the circular path. Since $m$ and $r$ are constants, $\vec{F}_{\text{wall}}\propto v^2$. Thus when $v$ increases, $\vec{F}_{\text{wall}}$ also increases.

For second part, let's say $N=\vec{F}_{\text{wall}}$. You may know $(F_s)_{\text{max}}=\mu N$. Thus $(F_s)_{\text{max}}\propto N$. So when $N$ increases maximum friction force also increases. But unless $mg=\text{max}\ F_{\text{fric}}$ only static friction acts. So $F_{\text{friction}}$ changes according to $F_{\text{grav}}$ to keep net vertical force zero. So the vehicle is vertically balanced. Thus, it will not fall.

So, there is a required $F_{\text{friction}}$ to balance vehicle vertically and it depends on $N$ and $N$ depends on $v$.

I leave it to you to conclude what will happen if the vehicle moves too slowly. Though it is obvious within my answer.

Answer 2

When he moves faster, a larger acceleration is needed to turn his motion - that acceleration is what we call the centripetal acceleration:

$$a_c=\frac{v^2}r.$$

Such larger centripetal acceleration is causes by a larger centripetal force which is the normal force in this case. Newton's 2nd law in the horizontal direction tells us that:

$$\sum F=ma\quad\Leftrightarrow\quad n=ma_c.$$

So, to move faster, $a_c$ must increase which requires $n$ to increase. With the normal force increasing, so does the possible maximum static friction force:

$$f_s\leq \mu_s n.$$

A certain amount of static friction is required to avoid him falling since the static friction must balance out gravity.

The driver thus must move fast enough, $v$, for the centripetal acceleration to be large enough, $a_c$, for the normal force to be large enough, $n$, for the maximum static friction to be allowed large enough, $f_s$, to balance out gravity. Otherwise he will fall. This is why you can't do the motordrome motion at low speeds.