Why do the inner tyres of a vehicle rise when taking a turn at high speeds?

Question

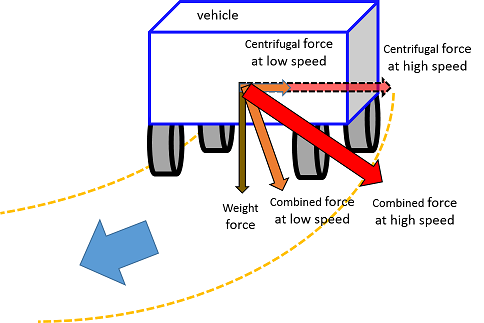
When a person cycles at high speed on a turn, his body bends inwards, to raise the horizontal component of the normal reaction to provide the centripetal force for the turn. The more the speed is, more is the centripetal force needed and hence more is the bending.

Now if in place of the cycle, we keep a bus, then similarly bus needs more centripetal force and hence the normal component should rise by bending the bus inwards. But if we consider common experience, bus bends outwards, raising the inner tyres. Does this not lead to normal reaction being applied away from the centre of the circle? Why does this happen?

Answer 1

When you go around a corner on a bike your body (and bike) leans toward the inside of the bend, to avoid being thrown over by centrifugal force (inertia).

When a bus goes around a corner, it cannot lean into the bend, as there is no rider forcing it to do so. As a result, centrifugal force pushes its centre of gravity towards the outside of the turn. Hence the bus will lean towards the outside. If it leans too far, it will fall over - exactly the same thing your bike would do if you didn't lean into the bend.

Answer 2

If the center of gravity of the bus is higher than the ground level (how could it not be?), then there is a net torque on the bus because centrifugal force acts outward from the center of the bend's curvature at the height of the center of gravity, while friction acts inward toward the center of the bend's curvature at the height of the wheels' contact with the ground.

If you were to attach a cable to the center of gravity of the bus and pull in a horizontal direction (at right angles to the long axis of the bus), the forces would be about the same as in the above case. Pull hard enough and the bus will tip over. The lower the center of gravity, the harder the cable will need to pull.

A bicycle banks into the curve because the rider forces it to do so. At the ideal banking angle, the gravitational torque due to the horizontal offset of the center of mass relative to the contact points of the wheels on the ground is exactly balanced by torque due to centrifugal force and the vertical offset of the center of mass relative to the contact points on the ground.

Answer 3

There is a rule: an object (set on the ground) will not topple as long as its weight force points to the ground within its supports. In other words, the bus does not topple so long as the imaginary arrow of the combined weight force (gravity force plus centrifugal force) ‘contacts’ the ground between the left/right or indeed forward/rear vehicle wheel pairs (see diagram below).

As the vehicle speeds up, centrifugal force increases with the square of the linear velocity. We go from the combined weight force contacting ground half way between the wheels (no centrifugal force), to movement of the ground contact position of combined weight force towards the outer wheels.

As the ground contact position moves beyond the outer wheels, the inner wheels are no longer supporting the vehicle weight, and thus the vehicle will generally tip over.