10
$\begingroup$

When a car's engine injects fuel into the cylinder chambers, the reaction creates a force that generates rotational momentum to the shaft and over the transmission, it translates that power to the wheels, right?

But, something's bothering me, how does the car actually move, is it dependent on the force of friction? Wheels have a contact with the road in one exact point and when the drive gets to rotate them, a force is exerted on the road in the direction of movement and there is friction that is counteracting it.

Is the key to get a car properly moving to have the drive force equal or less than the force of friction to keep that point locked to the ground and use the rolling of the wheel for translational displacement ie. moving forward? If it's bigger than the force of friction, that would cause the wheels to spin in place?

Am I completely off or am I getting something right? If there's a knowledgeable person on this topic, I'd greatly appreciate some insight, perhaps even a bigger response with some basic vehicle physics.

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Hi Scienation, and welcome to Physics Stack Exchange! We already have a few questions about the role of friction in driving that you might find useful. Also, I edited your post to make the title in the form of a question, which is the recommended way to post on this site (since it is a Q&A site). $\endgroup$
    – David Z
    Commented Dec 17, 2011 at 5:40
  • 2
    $\begingroup$ It's not right to say that "Wheels have a contact with the road in one exact point". I think you're imagining that the wheel is perfectly round and the ground is perfectly flat. In that case there would be a line of contact. In fact the tyres are soft (and the ground is slightly soft too), and they get squashed together under the weight of the car, so there is some area in contact. Even a trains wheels have some small area in contact with the track, not just a point. $\endgroup$
    – bdsl
    Commented Nov 16, 2013 at 20:31

4 Answers 4

Reset to default
6
$\begingroup$

Friction does help in movement of a wheel aka rolling motion.

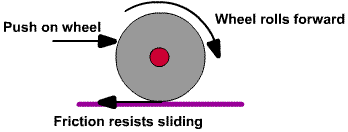
enter image description here

See the image, the point touching the surface actually stays at rest which causes the rest of the wheel to move forward. This is caused due to friction. Now, if you don't have friction, nothing would be there to stop the wheel from spinning around its axis.

Improve this answer
$\endgroup$
2
  • 7
    $\begingroup$ Please be careful to note in the image that the horizontal arrow pointing to the left (the one on top of the purple line) is the force made by the wheel pushing backward on the street, not the Friction force itself. I say this because the word 'Friction' is just below it so it might appear you are suggesting that. The Friction force has opposite direction to that (ie would be an arrow pointing to the right) in this example. $\endgroup$
    – user3723667
    Commented Oct 19, 2015 at 22:55
  • $\begingroup$ Friction (like every other interaction else) is a force-pair. The wheel pushes the road backwards, and the road pushes the wheel forwards. $\endgroup$
    – bdsl
    Commented Jan 17, 2020 at 20:45
4
$\begingroup$

Yes , friction is the driving force for the car to run, consider simple example where you put grease on road, is the car able to move properly..? no..because friction becomes very less..hope you got the point...

Improve this answer
$\endgroup$
1
1
$\begingroup$

Yes I think it is propulsion due to rolling, also the tires put a reaction on the road. The engine spins the wheels through transmission as you push the gas pedal and the engine accelerates, then you shift to higher gear and spin the wheels faster with less engine acceleration as you go to higher speed.

Hope that answers the question.

J

Improve this answer
$\endgroup$
1
$\begingroup$

First and foremost, here is the free body diagram of the wheel. The wheel is moving to the left and the friction is holding it back in the opposite direction. The $c$ here is damping from the bearing of the wheel, but I am not going to explain that further because we are focusing on the friction between the wheel and the ground.

Wheel Free Body Diagram

From the free body diagram, the friction is only written as $F_t$, mainly because there are two types of frictional force, and they both play a role here. The static frictional force $f_s$ and the kinetic frictional force $f_k$ can be written as

$$f_s = \mu_s N \\ f_k = \mu_k N $$

where $\mu_s$ & $\mu_k$ are static and kinetic friction coefficient respectively and $N$ is the normal force.

Now, if we try to move a block of wood from a stationary position by applying a force to it, the friction force is not constant. It follows the diagram below

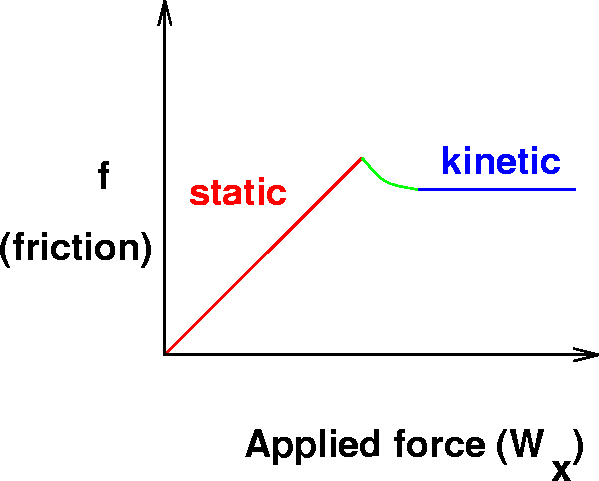
Static and Kinetic Friction
(source: ucsc.edu)

So, we start with not enough force to move the wooden block, what we feel is actually the static frictional force holding back our applied force. Without this, then the block would move with just a tiny amount of force (marked red). Next, if we apply more force, the friction holding it back will increase until a threshold point (marked green); this is at the exact moment when the block is just about to move. Then, as we apply even greater force, we will eventually move the block and the friction would remain constant even if we keep applying more force (marked blue).

Thus, in a car, we can imagine the scene as something similar to that block of wood. When moving, the engine rotates the wheel with just enough torque so that the wheel can "grip" the road using the static frictional force. Does that mean the car doesn't move? No, because the bottom of the wheel is held back by friction, therefore there is no motion relative to the two surfaces. Also, because the torque generated by the engine, it still wants to rotate. Since the bottom part doesn't move, it moves forward

On the other hand, if more torque is applied (or the surface is slippery, thus reducing the static frictional coefficient) then the wheel would slip or spin in place (e.g. when going through mud). Or simply put

Wheel friction

I hope this answers your question. There are a lot of resources on the internet on this topic. This is just a summary based on the available information.

Improve this answer
$\endgroup$
1
  • $\begingroup$ It is my understanding that in the FBD the friction should actually point to the left. What should point to the right is the force representing the push made by the wheel to the ground. $\endgroup$
    – user3723667
    Commented Oct 19, 2015 at 23:07

Not the answer you're looking for? Browse other questions tagged or ask your own question.