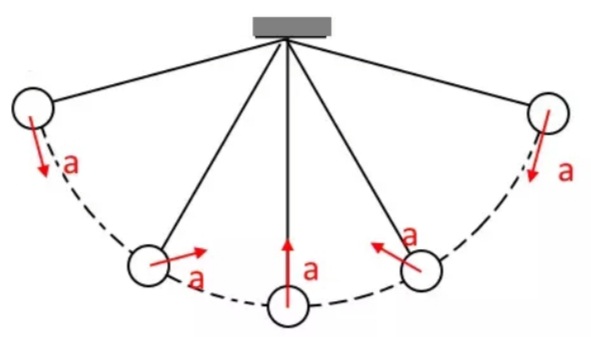
The following diagram shows the direction of the acceleration of a pendulum at different states.
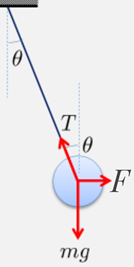
 Also, consider the below diagram. It shows the state before the pendulum starts simple harmonic motion.
Also, consider the below diagram. It shows the state before the pendulum starts simple harmonic motion.
In this situation, a horizontal force is exerted on the bob in addition to the tension by the string and its weight and also it is in rest. According to the theorem of the parallelogram of forces, the resultant of $T$ and $mg$ acts horizontally along the opposite direction of $F$. Just after $F$ is removed, the net force on the bob should act along the horizontal axis. According to $\vec F=m\vec a$, the initial acceleration should be along the horizontal axis. But this idea contrasts with the first diagram mentioned above.
In my opinion, if the bob moves a little bit ahead horizontally, the string will loosen and there will be no tension. So it will gain a downward acceleration caused by its weight. When it tries to go down the string tightens again and tension arises. So the direction of the net force is changed again with the direction of acceleration. This is unnoticeable in comparison with time. But I am still not sure about this. Please help me for a better solution.