0
$\begingroup$

i was solving some question based on harmonic oscillations and a question popped up:

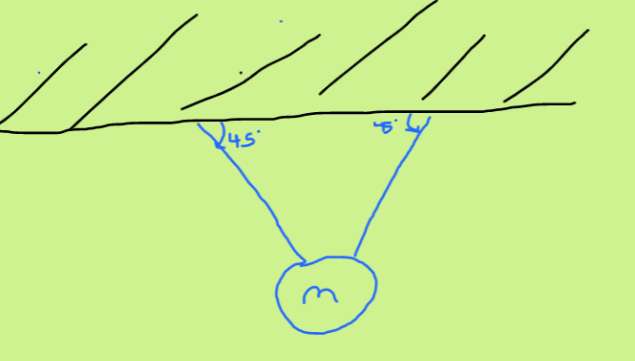
enter image description here

If the angle between the the wires and the surface is 45

and the mass of the bob is $m$ calculate the time period of the pendulum when displaced slightly in the horizontal direction(small oscillations)\

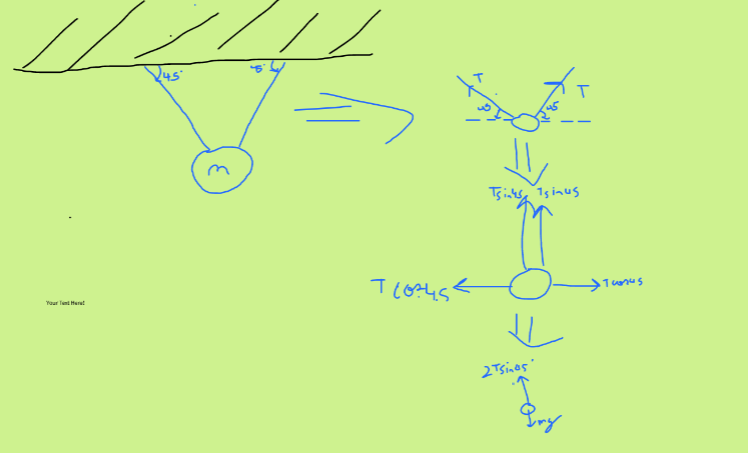
so, if u break the tension vectors acting on the bob as follows:

enter image description here

therefore, $mg=2Tsin45$

now if we see the pendulum from the left side such that only one wire faces u it looks planar and

enter image description here

since $theta$ is very small $sintheta= theta$ let the length of one string be $l$ $x/l=theta$ restoring force =Mgsin(theta)=mg*theta

ma=-mg*theta .......... T=2pi $(\frac{\sqrt l}{\sqrt g})$

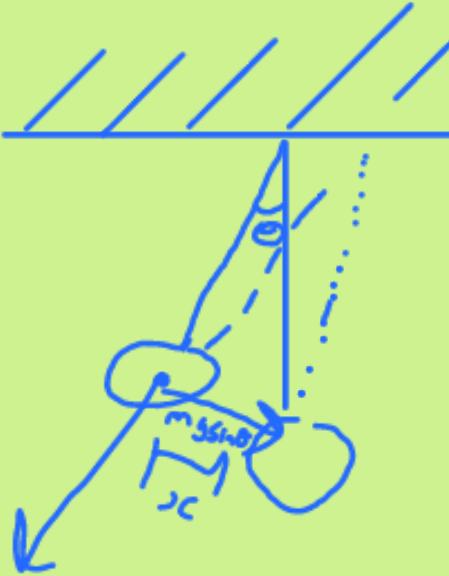
but it turns out u need to use something called the effective length of a pendulum which is given by :(

L_effective=L/${\sqrt 2}$

can some one explain why am I supposed to find the effective length and what is wrong with my aproach??\

(please excuse my bad drawing, I had to draw with my mouse:/)

Improve this question
$\endgroup$
1
  • $\begingroup$ You can't use statics when the bob is moving. This is demonstrated by the standard problem of a rod suspended by two wires at its end, and when one wire is cut the other wire only supports $1/4$ of the total weight, down from $1/2$ of the total weight in the static case. $\endgroup$
    – John Alexiou
    Commented Sep 11, 2023 at 16:36

1 Answer 1

Reset to default
0
$\begingroup$

You must consider the distance between the fulcrum and the oscillating mass. If both wires are of length $l$ and the angle between each wire and the horizontal is $\frac{\pi}{4}$ then the distance between the fulcrum and the oscillating mass is $d=l\sin\frac{\pi}{4}=\frac{l}{\sqrt{2}}$.

Improve this answer
$\endgroup$
9
  • $\begingroup$ why is that so? $\endgroup$
    – user287374
    Commented May 6, 2021 at 10:38
  • $\begingroup$ Which part of my response are you asking about? $\endgroup$
    – Michael Riberdy
    Commented May 6, 2021 at 10:39
  • $\begingroup$ "You must consider the distance between the fulcrum and the oscillating mass" $\endgroup$
    – user287374
    Commented May 6, 2021 at 12:12
  • $\begingroup$ "If both wires are of length l and the angle between each wire and the horizontal is π4 then the distance between the fulcrum and the oscillating mass is d=lsinπ4=l2√." $\endgroup$
    – user287374
    Commented May 6, 2021 at 12:12
  • $\begingroup$ The normal length of a pendulum is the distance between the mass and the fulcrum. The question becomes: why would that change in this case? The second point is some trig based on your diagram. Drop a perpendicular from the vertex at the 90° angle to the hypotenuse. The length of that perpendicular is $lsin(45°)$ if $l$ is the length of the legs. That is the distance in question. $\endgroup$
    – Michael Riberdy
    Commented May 6, 2021 at 12:31

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.