Can you intuitively explain the decreasing time-period of oscillation with increasing pendulum length in some cases?

Question

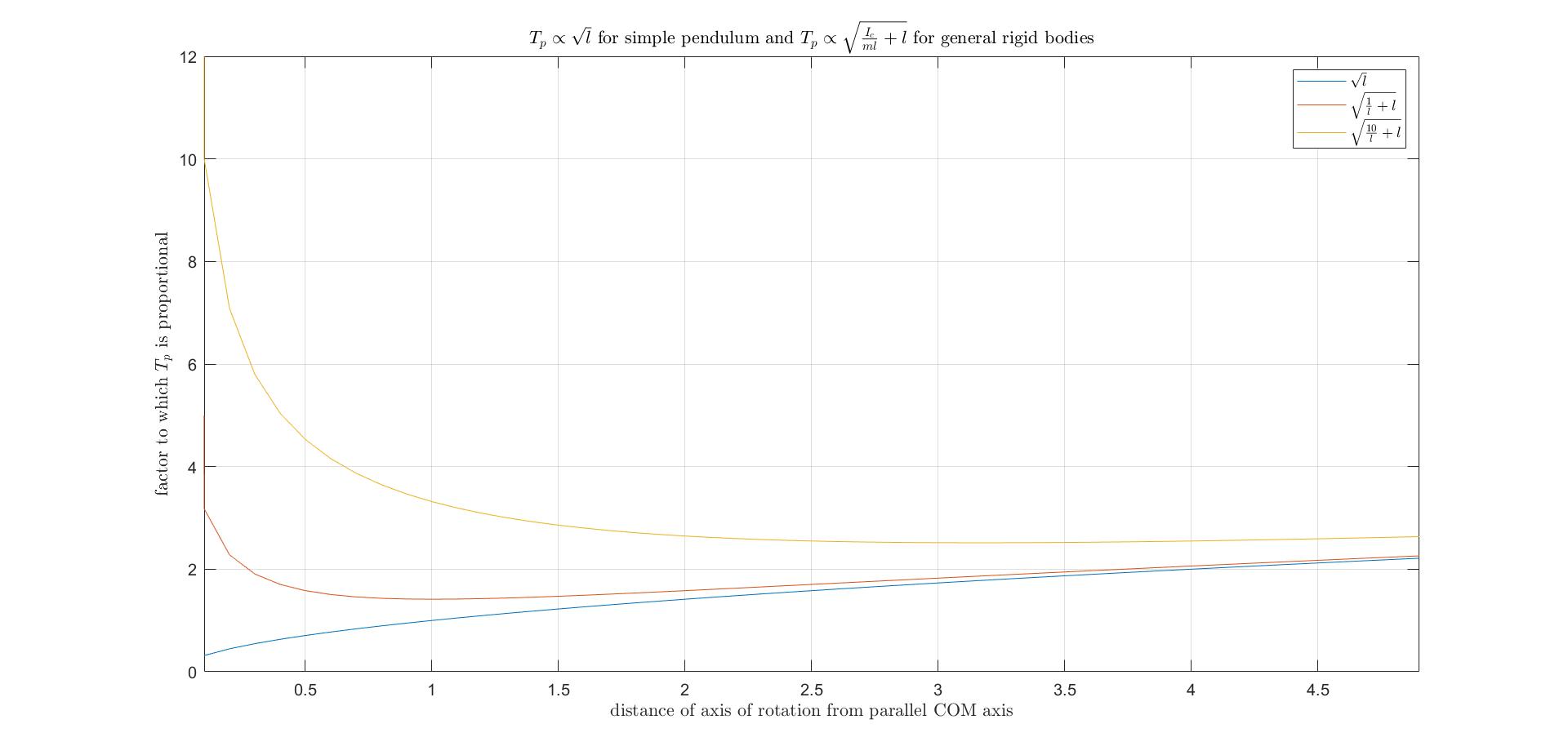
Consider a rigid body suspended about an axis of rotation which, in general does not pass through it's center of mass (COM) and has a moment of inertia (MOI) $0 < I_{axis}$ about that axis. Let $I_C$ denote the moment of inertial of the object about an axis parallel to the one mentioned before and passing through the COM. The parallel axis theorem implies that $I_{axis} = I_C + ml^2$ where $0 \leq l$ is the distance between the two axes. From the rotational dynamics of the object $I_{axis} \ddot{\theta} = -mgl\theta$, where $0 \leq \theta \rightarrow 0$ is the (small) angular displacement of the object, we can fine the time period of oscillation as $T = \frac{2\pi}{\sqrt{\frac{mgl}{I_{axis}}}}$ so that $T \propto \sqrt{\frac{I_{axis}}{ml}}$.

In case of the simple pendulum, $I_C \approx 0$ so that $I_{axis} = ml^2$, and thus $T \propto \sqrt{l}$ leading to the usual conclusion (matching our intuitive physical understanding) that the time-period of small oscillations increases as the length $l$ increases. However, in the case of general rigid bodies, i.e. not point-masses, the algebra results in $T \propto \sqrt{\frac{I_C}{ml} + l}$. From the plot one can see that this expression explains the apparently counter-intuitive observation that for general rigid bodies (i.e. not point-masses), for small $l$, the time-period of small oscillations reduce as the length $l$ increases, in a mathematical sense.

In the case of a simple pendulum, it is physically intuitive that the time-period should increase with the increase in $l$ (distance traveled over an oscillation is increasing linearly with $l$, while the motivating force remains roughly of the same magnitude regardless of $l$). In the same sense what is the intuitive physical explanation of this apparently counter-intuitive behavior?

Answer 1

Consider a pendulum that consists of 2 points of mass $m$ connected by a massless rigid rod of length $h$. Suspend it at the center of mass. It doesn't oscillate.

Suspend it a small distance, $\delta x$, above the center of mass. Turn it 90 degrees. It oscillates slowly. The moment of intertial, $I$, is almost the same as if it was suspended at the center. The torque is $\tau = mg \delta x$. The angular acceleration is $\alpha = \tau / I$.

Suspend it $2 \delta x$ above the center of mass. $I$ is still almost the same. $\tau$ has doubled, and so has $\alpha$.

Consider other properties of the two cases.

• The distance the center of mass travels in a half oscillation has doubled from $\pi \delta x$ to $\pi 2\delta x$.
• The potential energy decrease when rotated upright has doubled from $mg \delta x$ to $mg 2\delta x$. So has the maximum rotational kinetic energy.
• The maximum angular velocity, $\omega$ has quadrupled.

These ratios hold at each angle during the half oscillations of each case. $\omega$ is quadrupled over a trajectory that is twice as long. The period has halved.

Answer 2

In the case of a simple pendulum, it is physically intuitive that the time-period should increase with the increase in l (distance traveled over an oscillation is increasing linearly with l). In the same sense what is the intuitive physical explanation of this apparently counter-intuitive behavior?

In my opinion, the behaviour of a simple pendulum is not so intuitive. I guess if a common visitor of the Paris Pantheon is asked about the period of the Foucault's pendulum, it would be no surprise if some answers put the mass as a variable for example, and miss the role of the length.

But, we can have an "educated" intuition of the rigid body oscillations: as the moment of inertia is $\alpha mr^2$, where $\alpha$ is some constant, increasing $l$ means decreasing the length parameter ($\frac{r^2}{l}$) inside the square root. The period is proportional to the square root of the "length" so to speak, if l is small compared to the other term. And the mass cancels.

So its behavior is intuitive in this meaning.