0
$\begingroup$

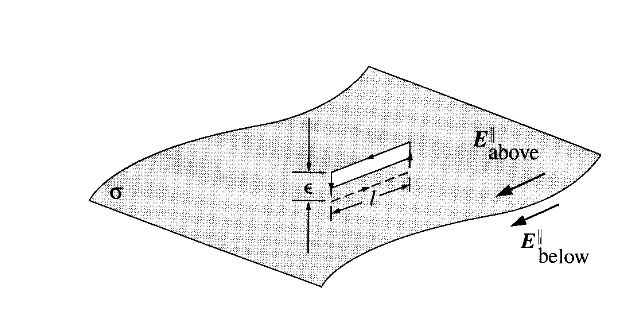
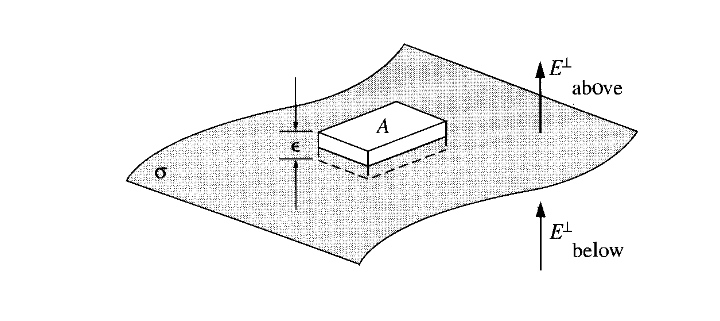
In the deduction of boundary conditions of electric field we take a surface and do calculations mathematically.enter image description here

enter image description here

enter image description here

Why do we take the direction of $E$ perpendicular and parallel in same direction (meaning the arrow in respective cases as shown in fig is parallel in direction ) across two surface? How can the deduction based on this assertion be used as a general one. (Meaning why not take the antiparallel case also)? What will happen if the direction are arbitrary.? Deriving the Electrostatic boundary conditions This link question's last part is related to mine, i just want to know why this specific orientation is taken as it won't be a general one?

Improve this question
$\endgroup$
3
  • $\begingroup$ The fields in those figures are meant to show components of a general field, not the total field itself. The consequences of a general field is found by combining the consequences of the two figures. $\endgroup$
    – garyp
    Commented Jun 2, 2021 at 11:47
  • $\begingroup$ Yes you are right but why the general components are taken parallel and not antiparallel .? If they are antiparallel then continuity of field will alter. So i want to know why they are parallel (general components) $\endgroup$
    – User 1
    Commented Jun 2, 2021 at 12:03
  • $\begingroup$ Are they taken as parallel due to continuity of Electric field lines? $\endgroup$
    – User 1
    Commented Jun 2, 2021 at 13:05

1 Answer 1

Reset to default
1
$\begingroup$

Its important to understand the Physics rarely depends on the choice / direction of coordinates that you take. Coordinates are a way for humans to comprehend and quantify things.

It doesn't matter which way $E^{above}$ and $E^{below}$ are directed. What matters is once you fix a direction for each vector you should change it mid way between calculations. You will always get the right direction provided you do the calculations right. As a matter of fact, in that particular figure, if the thin sheet is charged, then you will eventually see that the perpendicular components will turn out to be opposite.

What the figures shows is just a convention of vectors being directed along the positive axes.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thank you @TheImperfectCrazy. $\endgroup$
    – User 1
    Commented Aug 27, 2021 at 18:09

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.