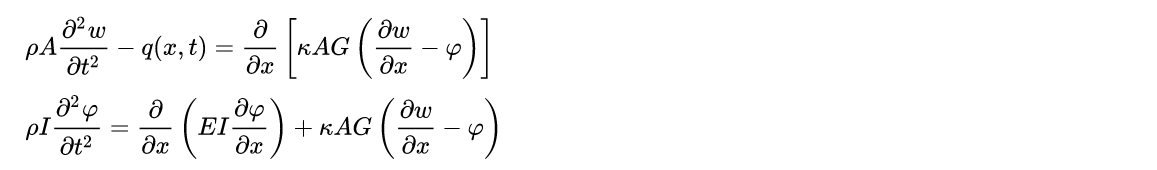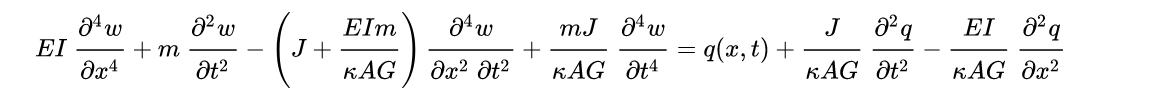0
$\begingroup$

I am trying to learn how to model a Timoshenko beam which is described here:

https://en.wikipedia.org/wiki/Timoshenko%E2%80%93Ehrenfest_beam_theory

There are a few things I can't understand but the main one is where they try to create a combined equation of motion. They state:

The Timoshenko beam theory, allowing for vibrations, may be described with the coupled linear partial differential equations:

enter image description here

For a linear elastic, isotropic, homogeneous beam of constant cross-section these two equations can be combined to give:

enter image description here enter image description here

where the dependent variables are $w ( x , t )$, the transverse displacement, and $φ ( x , t )$, the angular displacement. Note that unlike the Euler–Bernoulli theory, the angular deflection is another variable and not approximated by the slope of the deflection.

But I don't understand - how were these two equations "combined" to get the third?

It's also funny because they say $φ(x,t)$ cannot be approximated by $\frac{∂w}{∂x}$ but I am still getting the impression they still substituted this in their "combined" equation since all the $φ$ terms went away.

Can anyone explain how this works?

Improve this question
$\endgroup$
2
  • $\begingroup$ Did you try clicking on the helpful "Derivation of combined Timoshenko beam equation" box directly below that last equation on the Wikipedia page? $\endgroup$
    – Michael Seifert
    Commented May 15, 2021 at 21:14
  • $\begingroup$ Argh. Thanks Mike. I didn't even see that. :) $\endgroup$
    – mike
    Commented May 15, 2021 at 22:05

1 Answer 1

Reset to default
4
$\begingroup$

This sort of derivation is done by differentiating the coupled equations and then substituting the results back into the original, undifferentiated equations to eliminate the undesired variables.

In this case, if I were asked to reproduce the last equation, I would:

  • Isolate $q$ from the first equation.
  • Differentiate this equation twice to find expressions for $\partial^2 q/\partial t^2$ and $\partial^2 q/\partial x^2$ in terms of the derivatives of $w$ and $\phi$.
  • Write down the right-hand side of the desired equation in terms of the derivatives of $w$ and $\phi$.
  • Cancel out as many terms as I can and hope that I don't make a sign error somewhere.

Note that for a homogenous and isotropic beam, all of the beam properties are constant with respect to $x$, which simplifies the differentiation somewhat.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thanks Mike. That makes sense in the context of seeing the derivation wiki box. They have eliminated $ϕ$ because then everything can easily be solved based on displacements/derivatives (eg. in a finite difference model). Then the only remaining question I have is: If you were solving this in a finite difference model per time sample & x-increment, what would you put in for "$q$" and it's derivatives? They give $EI\frac{d^4w}{dx^4}=q-\frac{EI}{kAG}\frac{d^2q}{dx^2}$ so maybe use that to solve $q$ and it's derivatives backwards/recursively from the prior few samples' data? Thanks. $\endgroup$
    – mike
    Commented May 15, 2021 at 22:19
  • $\begingroup$ Or is $q$ just meant to be "any externally added force"? I see they use a $q(x)$ here with that intention as well for the similar Euler beam: en.wikipedia.org/wiki/Euler%E2%80%93Bernoulli_beam_theory $\endgroup$
    – mike
    Commented May 15, 2021 at 22:31
  • $\begingroup$ Yes, $q(x,t)$ is the external load per unit length as a function of position and time. It's meant to be something that you know, i.e., I apply such-and-such amount of force distributed in such-and-such a way over such-and-such a time period and see how the beam responds. If the beam has no external forces on it (other than at the ends, where the boundary conditions are enforced), then $q(x,t) = 0$. $\endgroup$
    – Michael Seifert
    Commented May 17, 2021 at 17:14

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.