1
$\begingroup$

In an exam question about photons scattering off protons it showed a resonance graph and asked why there was a lack of structure for energies much higher than the resonance peak. It alluded that the answer had something to do with the fact that at these energies the photons scatter of the constituent quarks rather than the proton as a whole.

Does that mean that, since quarks are elementary particles, there are no resonant states that can be formed from a photon interacting directly with a quark?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
2
$\begingroup$

Quarks are most peculiar elementary particles: they cannot be asymptotic states, and be observed in isolation outside hadrons.

So, no, a photon cannot excite them to some type of resonant hadronic bound state. How could it?

(A photon could, and often does, knock them out of the hadron, but they drag the requisite gluons and quarks out with them to generate their own color-singlet hadron cocoon.)

Improve this answer
$\endgroup$
12
  • 1
    $\begingroup$ Yes, all resonances are basically hadrons (color singlets!)--it's just that they decay too quickly, but that's a quantitative, not qualitative, distinction. $\endgroup$
    – Cosmas Zachos
    Commented May 6, 2021 at 13:44
  • 1
    $\begingroup$ Yes you may say that. Your two options aren't really that different, though. Angular motion is a type of rearrangement. If the net spin of the hadron is different, we call it something else, and not an excited state. A good review of the quark model details the differing wavefunctions, etc... $\endgroup$
    – Cosmas Zachos
    Commented May 7, 2021 at 15:46
  • 1
    $\begingroup$ You are thinking about resonant dibaryons? These are very, very rare. You might study the PDG for those... $\endgroup$
    – Cosmas Zachos
    Commented May 7, 2021 at 16:14
  • 1
    $\begingroup$ Yes, essentially, for baryon-baryon scattering. You have no such problem for baryon-meson scattering, or meson-meson resonances. $\endgroup$
    – Cosmas Zachos
    Commented May 7, 2021 at 16:28
  • 1
    $\begingroup$ Sorry, you want an open tutorial on baryon resonances, and you might try separate questions on each tangent. Dibaryons never crop up in photon-proton scattering. $\endgroup$
    – Cosmas Zachos
    Commented May 7, 2021 at 16:48
1
$\begingroup$

Most hard photons scatter off partons, breaking up the nucleon (per Cosmas Zachos's answer). There is an amplitude for exclusive processes (including a resonance), but it falls rapidly with energy according to "the constituent counting rules". Here you count the point particles in the initial and final states: $n$.

The scattering cross section then scales:

$$ \frac{d\sigma}{dt} \propto \frac 1 {s^{n-2}} $$

so any resonance production will be too tiny to see against the DIS background.

Here's some TJNAF data showing the behavior in $\gamma +D \rightarrow n+p $ (this reaction has a kinematic region with no background):

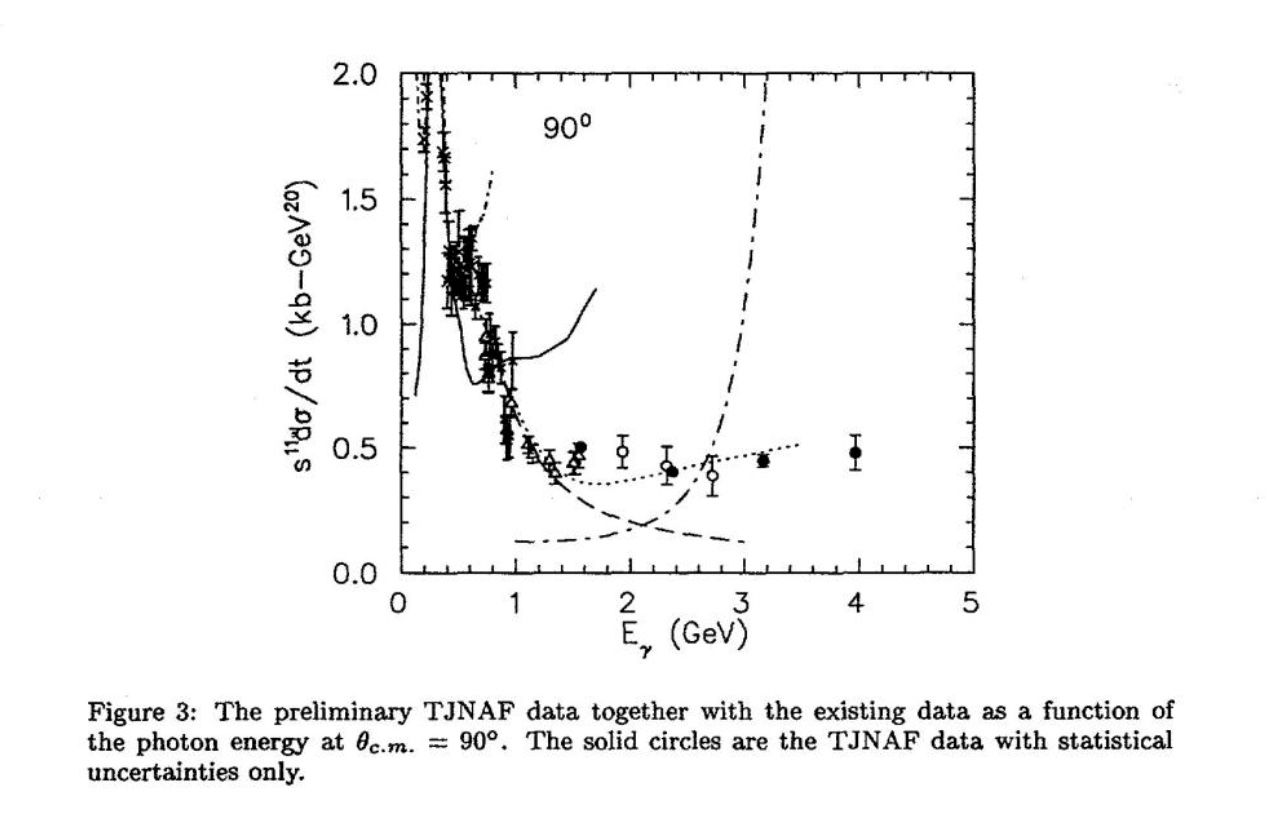
enter image description here

The onset of scaling occurs around $E_{\gamma}=1.5\,$ GeV, where the photon wavelength:

$$ \lambda =\frac{\hbar c}{E_{\gamma}} = \frac{0.2\,{\rm GeV\cdot fm}}{1.5\,{\rm GeV}}=0.13\,{\rm fm}$$

is an order of magnitude smaller than the proton, and the energy is 700 times the binding energy of the deuteron.

If you consider all the reacting hadrons to be Lorentz flattened and time frozen non-interacting disks of quarks, then the constituent counting follow from simple geometric considerations.

Improve this answer
$\endgroup$
2

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.