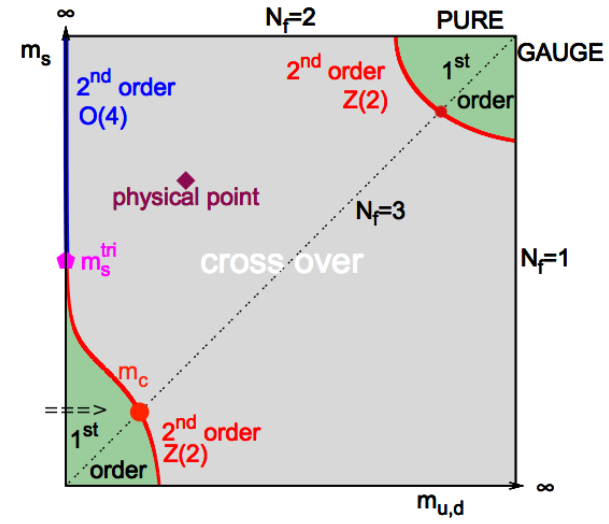
Overall, such a Columbia plot tells you what kind of phase transition (between the confined, hadronic phase and the deconfined quark-gluon plasma phase) can be observed for specific values of the quark masses. For intermediate quark masses, a crossover is generally observed while regions characterized by first-order phase transitions are found in the limit of heavy as well as light quarks. These regions are separated from the crossover regime by critical lines of $Z(2)$ second-order phase transitions. It should be noted that the behavior for small quark masses is subject to current research and that the variant you posted here is not the only possibility.
The quark masses are given as variables as one can study arbitrary masses in lattice QCD calculations. Particularly, it is generally easier to work with heavier and/or degenerate masses from a computational point of view. Besides that, it is also of theoretical interest how QCD behaves in certain (unphysical) regimes.
The diamond labeled 'physical point' corresponds to the quark masses found in nature and is the only point in the Columbia plot which is directly related to the QCD phase diagram. It corresponds to the point at $\mu=0$ at which the phase transition occurs ($T_c\approx 150\,$MeV). The fact that a crossover is observed is often represented by a dashed line in the phase diagram.
There also exist three-dimensional variants of the Columbia plot in which the baryochemical potential is added as a variable. These explore the type of the QCD phase transition away from the $\mu=0$ axis. These calculations are, however, more complicated due to the appearance of a so-called sign problem.