Lattice QCD Link Variables Meaning of $\mu$ and $\nu$

Question

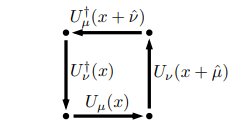
I'm currently coding a lattice QCD project and ran into an issue with my understanding.

A link variable connecting two points could be in the $\mu$ or $\nu$ direction, for example, $U_\mu(x)$ goes from bottom left to bottom right on the diagram and $U_\nu(x)$ goes bottom left to top left, but what do these directions actually mean? Are both of these directions somehow along the same 1-D axis $x$? Or is $\mu$ is in the direction of the specified axis and $\nu$ is in the direction of time? (Both of these ideas do not work since you cannot go in two perpendicular directions on a 1-D line and $\mu$ and $\nu$ both apply to link variables with time; $U_\mu(t)$ and $U_\nu(t)$ exist)

It would also be great to know what 2-D slice of the 4-D lattice the diagram represents and the relative coordinates of the sites shown.

Diagram taken from https://fse.studenttheses.ub.rug.nl/20342/1/BRP_Thesis_Piter_Annema.pdf page 12

Answer 1

In this context $x$ labels a four-vector $x=(x_0, x_1, x_2, x_3)$ in euclidean spacetime and $\mu$ and $\nu$ label arbitrary spacetime directions (with $\hat{\mu}$ and $\hat{\nu}$ labeling the unit-vectors in those directions). In that sense the diagram you are referring to is representative of any 2-D slice out of the 4-D lattice.