How do I find the unit vector for the tangent? [closed]

Question

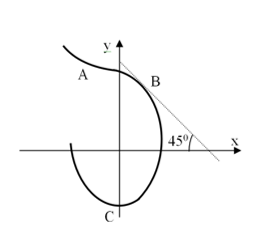
A body moves in the track from A to B to C and so on. The magnitude of the velocity in any given moment is $|\vec V|$=$t^3$$[m/s]$. The body arrives to point B at $t$$=$$2$$[s]$. The line in the draw is tangent to the track at point B and makes a $45^\circ$ with the negative x axis.
a. Calculate the tangential vector at point B.
b. At point C which is at the bottom of the track, the magnitude of the acceleration is $|\vec a|=78.103[m/s^2]$. The body arrives to this point at $t=5[s]$. Calculate $\vec a_y$
Attempt: a. I found the magnitude of the acceleration $a$ but i'm clueless on how to continue.
as for b, im not sure at all. I could really use any help or guidance.

Answer 1

These are Hints.

---

For (a) : The tangent at point $B$ makes an angle of $45^o$ with negative x-axis. The unit vector (towards the tangent at this point) is given by $$\hat{v}=\cos\theta\hat{i}+\sin\theta\hat{j}$$

where $\theta$ is angle from x-axis( can be computed from the angle that is given). The direction of velocity vector is tangent to the curve (so it's same as the unit vector computed). The magnitude of the velocity vector can found from $|\mathbf{v}|=t^3$ at $t=2$ sec.

---

For (b) : The general velocity vector can be written as $$\mathbf{v}=|\mathbf{v}|(\cos\theta\hat{i}+\sin\theta\hat{j})=t^3(\cos\theta\hat{i}+\sin\theta\hat{j})$$ where $\theta=\theta(t)$.

Now If you differentiate the following you get the acceleration as $$\mathbf{a}=\frac{d\mathbf{v}}{dt}$$

At point $B$ the slope is zero and thus $\theta=\pi$. Put this when you have calculated acc. Now what you will get will be in term of $\theta'(t)$. This can be found from the fact that the magnitude of acceleration at this point is given. Onece you find out accl, so the $a_y$.

Answer 2

Decompose acceleration in tangential and normal directions. Only the tangential acceleration contributes to a change in the magnitude of the particle's velocity.