1
$\begingroup$

Take the (111) surface of a fcc lattice:

fcc(111)

If we have a wave incident on the surface moving in a direction with a projection parallel to the orange line, then in principle we should observe diffraction in the scattering plane, since there is a periodicity in the surface in this direction.

However, what we would find is that we do not observe a 1st order, though there is a second order peak. Mathematically this is a consequence of a smaller periodicity in the directions indicated by the red and green lines.

Physically though it would be nice to understand the absence of 1st order peak in terms of destructive interference. Do you see a way to explain these absences in terms of a cancellation of scattered waves?

Improve this question
$\endgroup$
5
  • $\begingroup$ I've added an answer, let me know if it's helpful or if it needs clarification. Can you add some details as to why you say the 2nd order peak is not present, and exactly what you mean by 2nd order? A side-view drawing would be helpful. Thanks! $\endgroup$
    – uhoh
    Commented Jul 11, 2021 at 7:02
  • 1
    $\begingroup$ It's been awhile since I wrote this, and looking back I am now finding that we should expect 2nd order diffraction for a scattering plane intersecting the orange line. By 2nd order I mean that the path length difference between trajectories scattering off of neighboring rows (two neighbors would be, e.g., the beginning and end of the orange line) is two lambda. $\endgroup$
    – creillyucla
    Commented Jul 12, 2021 at 21:43
  • 1
    $\begingroup$ Indeed, in G Boato et al 1976 J. Phys. F: Met. Phys. 6 L237, they observe diffraction of molecular hydrogen at 38.5 degrees for an incident angle of 18.5 degrees. For the Ag(111) surface along the orange direction we have a periodicity of 500pm, so that the scattering has a path length difference of 152pm. Their H2 beam was estimated to have a de Broglie wavelength of 75.2 pm, which is almost exactly one half the path length difference, which implies second order diffraction. $\endgroup$
    – creillyucla
    Commented Jul 12, 2021 at 22:04
  • 1
    $\begingroup$ Your explanation in terms of cancellation of wave scattering from equivalent rows is exactly right. Another way of putting it would be to say that the projection of the scattered wave onto another plane wave travelling in the scattering plane should be unchanged by a translation of the surface by a green or red vector. This is however equivalent to a simultaneous translation of the ingoing and outgoing plane waves, which are invariant under the lateral component of the green/red translation. There will however be phase shifts due to the vertical component, which is half the orange vector. $\endgroup$
    – creillyucla
    Commented Jul 12, 2021 at 22:12
  • $\begingroup$ Thank you for your feedback! I'm now stuck on this Straightforward formalism to get four sets of 2D hexagonal lattice vectors of fcc(111) planes that I can also cite? and speaking of molecular scattering from surfaces, there's ]Particles in a box simulation of vacuum system; how to treat scattering from walls?](physics.stackexchange.com/q/539969/83380) which was probably closed for not being easy to answer. $\endgroup$
    – uhoh
    Commented Jul 13, 2021 at 1:09

1 Answer 1

Reset to default
1
$\begingroup$

I happen to have a slide about something similar, so I'll adjust it and paste below.

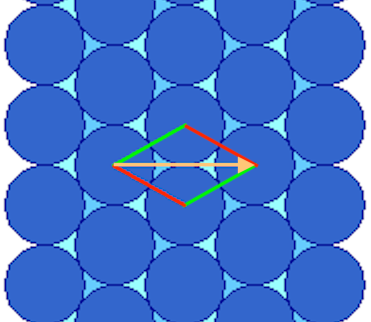
If the red and green lines are the (acute) 2D lattice vectors for the 2D hexagonal lattice on the fcc(111) face, we can call those directions [1, 0] and [0, 1]. Then "orange" direction could be called [1, 1]. As far as I can tell from the two images below, in the 3D lattice those directions could be called either [1, -1, 0] & [-1, 0 1] or [1, -1, 0] & [1, 0 -1].

Either way, add them together to get the orange arrow and it's [0, -1, 1] or [2, -1, -1] and the rules for allowed diffraction are all odd or all even and the orange line does not satisfy that

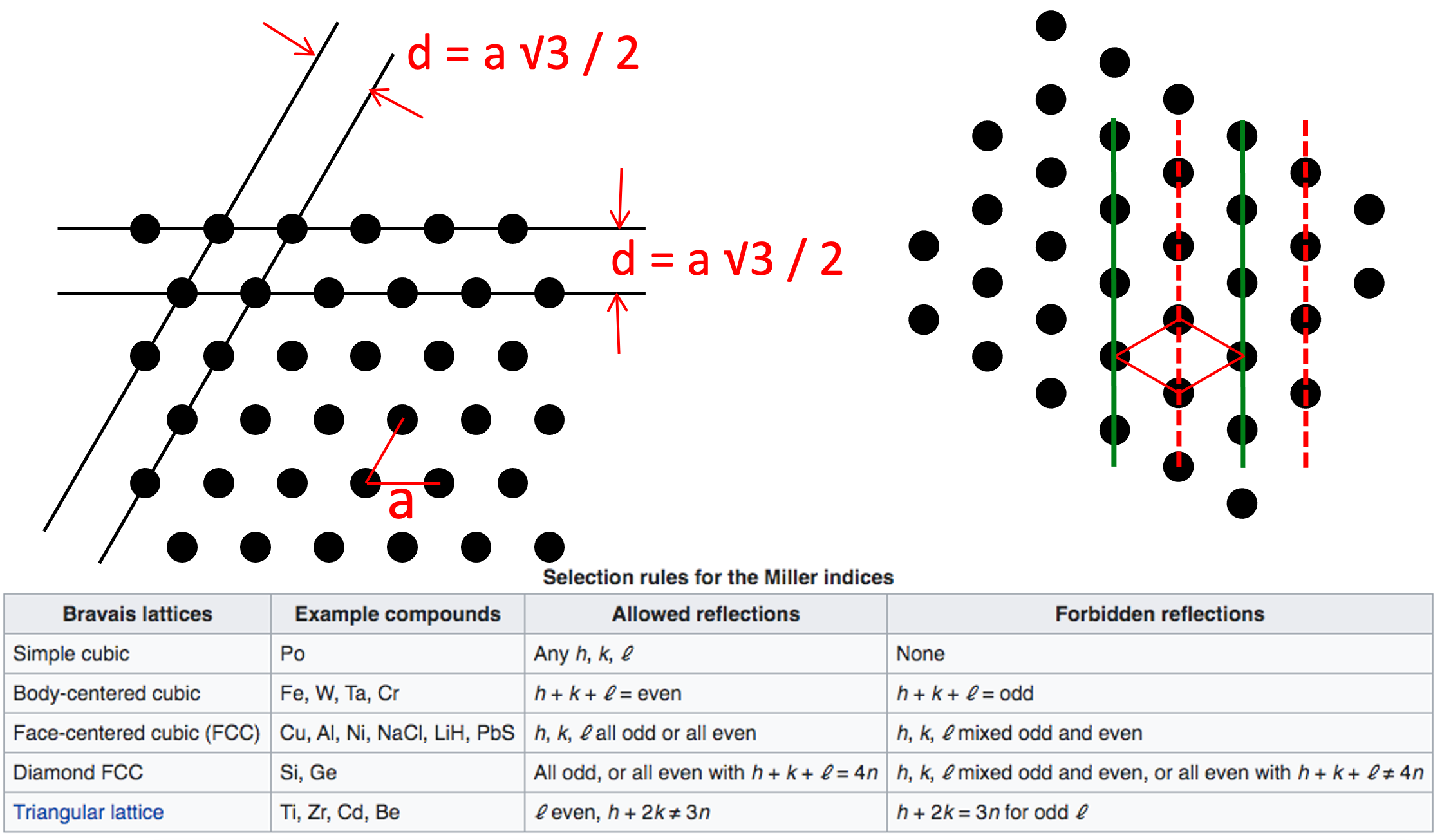
Before the intuitive answer, let's look at the selection rules for diffraction We can trust them while we learn to understand them.

Bravais lattices           Allowed reflections           Forbidden reflections

Simple cubic               Any h, k, ℓ                   None

Body-centered cubic (BCC)  h + k + ℓ = even              h + k + ℓ = odd

Face-centered cubic (FCC)  h, k, ℓ all odd or all even   h, k, ℓ mixed odd and even

Diamond FCC                All odd, or all even with     h, k, ℓ mixed odd and even 
                           h + k + ℓ = 4n                or all even with h + k + ℓ ≠ 4n

Triangular lattice         ℓ even, h + 2k ≠ 3n           h + 2k = 3n for odd ℓ

For the orange-arrow case in your question let's look again:

OP's diagram with bigger arrows

Now examine my old slide:

For your plane wave with a projected period on the surface equal to the [1, 1] direction, we can see that it passes TWO rows of equally spaced atoms. The reason that the selection rule says this diffraction is forbidden is that diffraction from the alternating rows of atoms cancel each other; they are 180 degrees out of phase.

my old slide

Trying to get the correct [hkl] notation for directions of the hexagonal lattice on the fcc(111) surface. These seem to :

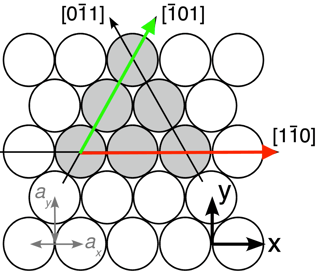
from Diffusive Atomistic Dynamics of Edge Dislocations in Two Dimensions from Reconstruction of steps on the Cu(111) surface induced by sulfur

Sources left: Diffusive Atomistic Dynamics of Edge Dislocations in Two Dimensions, right: Reconstruction of steps on the Cu(111) surface induced by sulfur

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.