Why is the central maximum white when white light passes through a diffraction grating?

Question

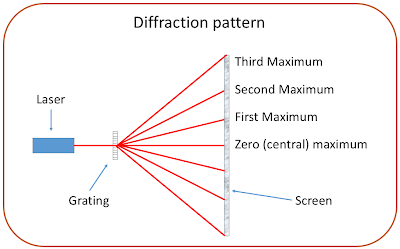
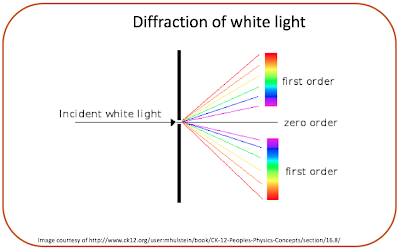
I can't see how path difference and central maximum (where path difference is 0) work when there are more than 2 wave sources.

When a beam of white light passing through a diffraction grating, it is obvious that the central maximum is a white fringe, while other bright fringes are arranged in spectra (due to the composition of white light).

However, I can't understand how the central maximum can be like this. When there are more than 2 circular wave sources, there will be no points where the path difference is 0 for all the waves. Therefore, there should be no path where the antinodal lines from all coloured lights overlap to produce a white fringe. Can someone please point out what is wrong with my reasoning above?

Answer 1

I am not sure if I am using the same geometry as you. This is my best guess.

Here are a couple images from https://sites.google.com/a/perthgrammar.co.uk/physics/courses/higher/particles-and-waves/35-interference-and-diffraction/353-diffraction-of-waves

This is using a laser with a single wavelength. The important thing is it has a maximum in the center.

Here is a similar image with many lasers with different colors. The higher order maxima do not occur at the same place, leading to rainbows. But they all have a central maximun at the same place. All those colors add to white.

Answer 2

The reason is that all colors are in phase there. The so called zero order maxima concide and their sum produces white light.

Answer 3

We are using a far field approximation. Each groove in the diffraction grating acts a source of circular waves and it's true that the distances from each groove to the central maximum are different. But once you get far enough away from the grating these differences in path length become much less than the wavelength of light. So while light from each groove isn't travelling exactly the same distance to the central maxima and thus won't arrive exactly in phase it's close enough to produce constructive interference.