How do subatomic particles have mass, velocity, spin etc if they are waves? [duplicate]

Question

I don't think I understand the concept of sub-atomic particles very well. How can an electron or any sub-atomic particle have mass and spin if they are waves?

Answer 1

This is because of the dual nature of quantum mechanical objects, which furnish them with the properties of waves when observed in one experimental context and as particles when observed in another.

For example, an electron that is being shot down the beam tube of a linear accelerator along with millions of its pals can be conveniently visualized as a speeding bullet which is going to bounce off a target proton, thereby allowing us to determine its shape and size.

Quantum mechanics says that at the same time, the electron bullets (which exhibit mass, charge and spin) with which we are machine-gunning the proton have a wavelength that shrinks as the energy of those bullets is increased, and if we increase the energy of the electrons enough, their wavelength becomes much smaller than the diameter of a proton and they start resolving the presence of the quarks inside the proton when they smack into it.

Those quarks are invisible to us when the wavelength of the electrons in the beam is larger than the diameter of the proton, in which case the scattering pattern tells us that the proton is instead a tiny sphere with a well-defined diameter.

Answer 2

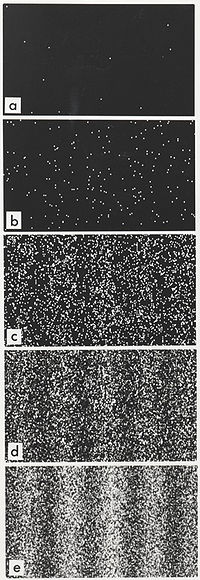
The experiment "scattering electrons one at a time from a given double slit" can give a clear distinction of what "waves" mean in elementary particles .

From a to e accumulation of electrons over time.

The experiment shows that the footprint on the screen of an individual electron scattering through a slit is a dot, a classical particle footprint within the accuracies of recording of the screen.

As time goes on more and more dots appear , that seem random.

Then, lo, a pattern starts to appear, an interference pattern characteristic of waves!!

At frame e one can calculate a wavevelegth for the interferering wave, BUT, it is not the electron that is waving, each individual electron leaves a solid footprint of a point. It is the probability of seeing an electron at (x,y)on the screen that is wavelike.

This is in accordance with the theory of Quantum Mechanics, which fits the probability of interaction of elementary particles, with solutions of the quantum mechanical wave equation.

The particles are point particles with mass and charge and spin etc, but their probability of interacting with each other obeys wave equations.

Answer 3

Talking about the 'dual nature' of quantum mechanical objects can confuse newcomers.

Quantum systems are not either particle or waves. They are in fact neither because both concepts are classical in nature. This, of course, is well known.

Less well known (to paraphrase Adami) - quantum systems (e.g. an electron) don't take on the 'coat' of a wave or a particle when it is being observed. They remain "neither". Rather, they appear to an experimenter the way you choose you want to see them, when you interrogate a quantum state with classical devices.

Nevertheless, if used in a clever manner, these classical devices can enable you to learn something about quantum physics.

Answer 4

How can an electron or any sub-atomic particle have mass and spin if they are waves?

Electrons behavior

Ask yourself in which cases electrons are treated as waves. I see two main cases. The first starts with a wave equation to calculate right the emission spectra of a hydrogen atom. The second is the distribution of electrons behind edges.

In both cases how you observe the phenomenon?

For the Schrödinger equation - called in Germany in the time of its invention Wellenmechanik (wave mechanics) -, the known spectra was used with boundary conditions to get the known spectra. More than this, this wave equation was influenced at least from Bohrs imagination of revolving around a nucleus electrons. To rescue this imagination against the argument, that a revolving electron has to lose energy, the wavelike revolution was introduces.

Please note that I call the wave mechanics an invention. In the mentione Wikipedia article are the next notes from Feynman and Schrödinger.

Where did we get that (equation) from? Nowhere. It is not possible to derive it from anything you know. It came out of the mind of Schrödinger.

Nearly every result [a quantum theorist] pronounces is about the probability of this or that or that ... happening—with usually a great many alternatives. The idea that they be not alternatives but all really happen simultaneously seems lunatic to him, just impossible.

For the distribution behind egdes, called interference pattern, you see what Anna published in her answer. Electrons appear as dots on the measuring instrument. What happens near the slits is unobservable, because the influence of additional fields - to measure the particles - destroy the path the electrons are moving.

Photons behavior

Photons indeed have a wave characteristics. They have an oscillating electric field and they have an oscillating magnetic field. In the interaction with subatomic particles they interact with these particles with their fields. The outcome in some cases are oscillating phenomenas. For example, a radio wave with synchronized and aligned photons is able to go through a wall because of the induction of phonons in the material.

Fazit

For some phenomena it is a good way to use wave equations. But this does not mean that the involved subatomic particles are waves. Only their interaction is describable as resonant to each other.

Answer 5

The particles are not "waves". They are particles - small, minute objects of a size at least much below what we can measure (whether they are true points or not is unknown, and impossible to prove empirically because all we can truly honestly say with any finite measurement is that it is "smaller than the error of the measurement") - at least, that is how the theory works.

You see, there is no experiment where you ever see a single electron (say) being a "wave" or some sort of extended object. Whatever you do to it, it always looks like a particle. The "wave" behavior only appears when you take a whole bunch of electrons and send them through the right devices and let them accumulate as a statistical aggregate, such as the famous "double slit" experiment. It's an aggregate effect. The wave pattern is built up by the individual particles, which will always look "particle-like".

The "weirdness" is because of the following: just as I described it, that isn't necessarily impossible with classical particles, either - a mass of interacting classical particles could indeed develop some kind of undulations within itself (think about a swarm of birds, for example) and thus produce the wave patterns. What gets funky is because you can send these particles one at a time, and still, in aggregate, it will build the wave pattern.

The trick, then, is not in the shape or "nature" of the particles as material objects, but in the propagation between source and destination. That is the trick. If you need even more argument in favor of this understanding, we can also send molecules - objects that clearly have finite extent and structure as objects, that even can be observed as such with suitable special extremely sensitive microscopes, and have them build a wave pattern as well. Clearly the molecule can't somehow be pulling apart or something like that to pass through the slits or it would be destroyed, and not arrive intact. The structure has to be preserved (or at least it's reasonable to say it is so) throughout the whole propagation since we can intercept them at any point without change. The propagation is non-classical.

So how do we describe the non-classical propagation? The answer is that we have to take a big step to say that the physical parameters of the particle, or the molecule or other object - are "fuzzy": we replace ordinary real-number quantities with probability distributions, which (at least in this author's opinion) are most sensibly understood as a subjective quantity, so do not directly belong to the particle but rather belong to a modeled (in the theory) information-collecting agent. We have to look through the agent's "eyes" to describe the process. The probabilities stand for reduced information (see entropy). When the agent acquires new information from the particle, then we update its probability distributions accordingly - similar to how if you've seen the weather man talking about a forecast for 50% probability of rain tomorrow, then tomorrow comes and it didn't rain, that is "updated" to 0%, likewise if we have a probability distribution for a certain quantity, say that it's 25% likely to be "1", 50% likely to be "2", and 25% likely to be "3", and we get a "3", then this becomes 0% likely to be "1", 0% likely to be "2", and 100% likely to be "3".

These probabilities are what make up the famous wave function, of which really there isn't just one, but many wave functions for each possible measurable parameter of the system (in the case of a particle, these are position, momentum, and any ). And this wave function can be extrapolated with the Schrodinger equation, and the extrapolation will develop a pattern like a wave.

But what is important to note about this is this wave is not a description of the shape of the object. The wave function $\psi_x(P)$ does not belong to "shape". It belongs to the position parameter (in this case) of the particle. The wave function bears the relation to a quantum particle that is the same as the coordinate vector $\mathbf{r}$, or better a geometric point $P$, bears to a classical particle. $\mathbf{r}$ is not the shape of a classical object, so neither is $\psi_x(P)$ for a quantum object. Thus it is wrong to look at the wave pattern formed in $\psi_x$ and say the particle is a wave. The electron is still a particle (as far as we can tell) - to say otherwise is as wrong as saying that because classical $\mathbf{r}$ can be drawn like a 3 meter long "arrow" from the origin, your tennis ball must be some 3 meters long object and not a small round fuzzy one. Rather, what both of these describe is the relationship of the particle to space, not its structure, and what happens in quantum mechanics is that this becomes complicated.

And this doesn't just apply to the position - it applies to the velocity (or momentum), as you say, as well. Velocity is fuzzy; that's "why" (in one sense) the probability distribution for position spreads out. In fact, you can describe a badly-informed classical-mechanical observer in a similar way, using probability distributions, and they do the same thing, but in quantum mechanics, this lack of determinacy is fundamental: the Universe just doesn't contain as much information, in some sense, when it comes to pinning the parameters of its objects.

So how do they have mass and spin and all that? They "have" them just as classical particles do. Only these attributes now become these weird, fuzzy, low-resolution quantities that our best description also requires us to factor in the actions of the observing agent and to describe transactionally therewith, and this fuzziness of the attributes is what is responsible for giving the particles the capability to statistically build up wave patterns in situations where classical mechanics would not allow that (and many other interesting phenomena).