Is the relativistic mass of a photon non-zero?

Question

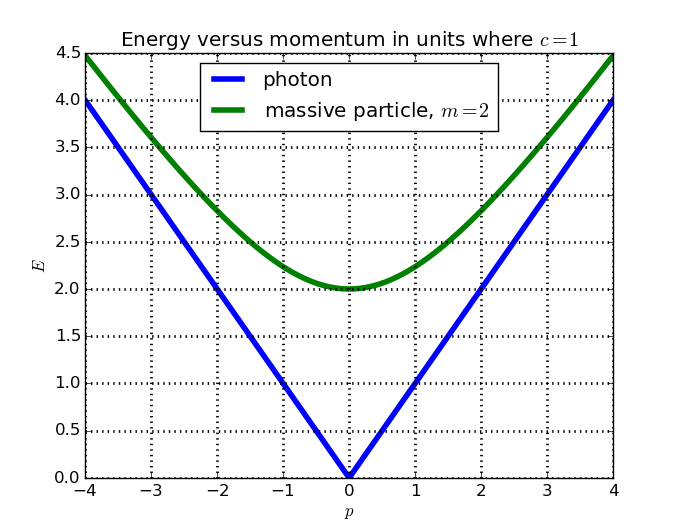
A photon has rest $m=0$, but it is never at rest, so it has no rest mass, then the relativistic mass is according to me non-zero because:

1. $E = \text{work}=m \times \text{acceleration} \times \text{displacement}$, if $m=0$ then $E=0$, which it is not
2. Light is trapped in a black hole
3. In the de-Broglie equation, $\lambda=h / (mc)$, if $m=0$, the expression is undefined, alternatively I can find the mass of a photon applying the wavelenghth of light in lamda
4. Light has momentum and energy, which is I think impossible with a zero mass.

I dont have a very vast knowledge in physics, please tell me where in each of this 4 cases I am wrong.

Answer 1

1. $E = m \times \text{acceleration} \times \text{displacement}$ is not the whole story even for normal matter once you get into special relativity. The full formula is $$E^2 = p^2 c^2 + m^2 c^4 \tag{1}$$ where $m$ is the rest mass, $p$ is the momentum, and $c$ is the speed of light. Photons have $m=0$ but $p \neq 0$.

2. I'm not sure why this matters. In general relativity we have bending of space-time so even though light travels on "null paths"$^{[a]}$ when those paths themselves are bent the light goes in a bent trajectory.

3. The deBroglie equation you've written is a semi-classical equation only applicable to massive particles. It't not even really correct for massive particles though, so don't take it too seriously.

4. Momentum is not just $p = m v$. One way to think about it is to invert Eq. (1) getting $$pc = \sqrt{E^2 - m^2 c^4}\,. \tag{2}$$ Therefore a photon with energy $E = \hbar \omega$ has momentum $p = \hbar \omega / c$. You can regard the energy of a photon $E = \hbar \omega$ as the fundamental starting point, and then Eq. (2) as another fundamental relation coming from the structure of special relativity. The fact that photons have $m=0$ really means that it is possible to create one with arbitrarily low amounts of energy. With massive particles like electrons creating one with zero momentum costs energy $E = m c^2$ as per Eq. (1). In other words, to create a stationary electron out of the vacuum costs $m c^2$ in energy. For a photon, you can create one with any amount of energy $E=\hbar \omega$ as long as the frequency $\omega$ is low enough. This idea is illustrated in the figure where we show the energy required to create a photon or a particle of mass $m=2$ with specified momentum $p$.

$[a]$: This is just jargon here but it refers to the fact that light moves on special "as fast as possible" paths.