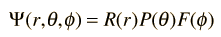Is it true every sub-atomic particle can have a mathematical representation as a wave? Regarding every theory about sub-atomic particles can all these theories be 'revised' in a way so they only talk about waves interacting and make no mention of the particle view of the theories ?
$\begingroup$
$\endgroup$
2
-
$\begingroup$ en.wikipedia.org/wiki/Wave_function $\endgroup$– lemonCommented Apr 17, 2015 at 0:09
-
$\begingroup$ See heisenberg uncertainty principles also $\endgroup$– user6760Commented Apr 17, 2015 at 1:13
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
9
Yeah, they can have a representation as a wave. What you have to realize is that we have absolutely no intuition about the world at particle scales. Here, in the macroscopic world, we easily distinguish thing as particles or waves. For example, sound and the motion of a string are clearly waves to us, while a basketball and a car are clearly particles. Non the less, when we go to particle scales, we found out that there is no sense in even trying to ask whether something is a wave or a particles because we found that it behaves as both! That's why the term particles-wave duality is nothing but a way of humans to say that they don't have a clue about what these things are and that's ok! We can't expect to have it since we don't have a day to day experience with these things.
Historically, as Dirac explains in his book Principles of Quantum Mechanics, the function $\psi$ which represents the state of a particle is called the wave function. Non the less, it resemble a wave only in certain physical configurations. Say, if you measure a particle's position with great precision, you will find the wave function looks more like a big spike than a wave. So, to precise, you can indeed describe every particle with a wave function (which sometimes has nothing to do with a wave) mathematically, but this doesn't mean every particles is a wave (nor is it a particle as classically described).
-
$\begingroup$ Why though do most theories about 'particle physics' I've heard of talk about mainly 'particles' and how they interact? Is it because quantum mechanics 'say's' these sub-atomic 'things' must be in discrete packages and therefore like particles? $\endgroup$– 201044Commented Apr 20, 2015 at 17:02
-
$\begingroup$ The usage of the word particle is just convention. It distinguishes between entities with different properties (charge, mass, spin, etc....) but has nothing to do with what the thing actually looks like. $\endgroup$ Commented Apr 20, 2015 at 19:59
-
$\begingroup$ That's very interesting. Do all physics authors regard it as just convention? Like an undefined term in Logic. It certainly seems as though some authors view particles as 'particles' ; almost like a bias towards something with a type of 'solidity' and not a wave.. $\endgroup$– 201044Commented Apr 22, 2015 at 14:19
-
$\begingroup$ For example a theory describing how an atom when 'given' energy and splits into smaller atoms plus energy; could such an event be described entirely using 'wave representations'? $\endgroup$– 201044Commented Apr 22, 2015 at 14:23
-
1$\begingroup$ Yeap, photons have discrete energies $\endgroup$ Commented Apr 27, 2015 at 12:54
$\begingroup$
$\endgroup$
Just for a completeness from an experimentalist's pov:
Is it true every sub-atomic particle can have a mathematical representation as a wave?
Experiments have determined the fundamental particles that define the standard model of particle physics. These are mathematically represented as point particles, with the mass in the table and the other quantum numbers shown, and enter in the Lagrangian with these values.
So there exists definitely a "particle" representation.
Regarding every theory about sub-atomic particles can all these theories be 'revised' in a way so they only talk about waves interacting and make no mention of the particle view of the theories ?
From the Lagrangian one generates the quantum mechanical equations that describe the kinematics and energy momentum balances of these elementary particles. These equations are wave equations, in that their solutions are sinusoidal similar to the solutions of classical waves. The simplest equation is the Schrodinger equation. A basic postulate of quantum mechanics is that the function
which is a complex function, when squared with its complex conjugate, gives the probability of finding the "particle" at (r,theta, phi) or (x,y,z,t) in general. So it is the probability that has the wave characteristics, which means to see the wave one must accumulate a lot of data for the same boundary conditions, as in the double slit single particle at a time experiment.
Thus yes, in a sense, everything needing a quantum mechanical framework, i.e. small dimensions and in general where the Heisenberg Uncertainty is important, can be described with the wave equations of quantum mechanics.
When coming to particles like nuclei which are made up of protons and neutrons, and which protons and neutrons are composites of the elementary quarks, etc. , approximations of potentials are used to solve for problems at hand, like the shell model.
Physics also displays a continuity between classical representations as particles, and the quantum mechanical entities which are "particles" hitting a screen and "waves" in the accumulation. For some problems the "particle" identification is adequate, as in fitting the tracks of charged "particles" in a magnetic field to get their energy and momentum, as is done routinely in high energy physics experiments. In others the wave identification is crucial, as in predicting interactions and crossections for the experiments.
In the comments to the other answer you ask:
Could the Universe and all the countless events and processes going on be thought of as 'energy and vibrations' and discrete 'bundles' or packages of waveforms of energy with no mention of particles anywhere? In other words one can interpret and describe any event as interactions of 'energy and vibration' with no mention of particles or any 'physical' traits.
It would be very very complicated and unnecessary, in a similar way that "temperature" is a useful macroscopic number important to our life: what use would it be if we had to measure all the particles in the air whose average kinetic energy squared builds up the temperature, instead of just reading a thermometer? So theoretically everything could be calculated with quantum mechanical wave equations and their solutions, but it would be very stupid to do so.
