As Fehértói-Nagy Lili pointed out, the observed diffraction pattern is a convolution between two functions:
- the diffraction pattern of a single aperture (e.g. rectangular or circular) and
- a function which accounts for the fact that we have two of these apertures.
Let's try to understand and visualise this effect. However, since the diffraction patterns of these apertures are complicates and in 2D, we will use simpler functions for the illustration: So let's suppose the single aperture is described by a "triangular" function (blue), and the fact that we have to apertures is describes by the "step" function (red):
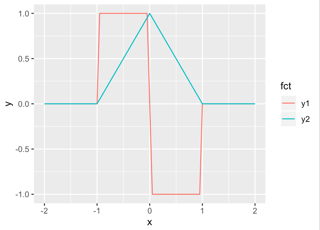
(Just to make sure: There is no connection between these functions and the actual function. These functions are merely used for illustration.)
The effect of the convolution is to slide the blue triangle across the red step function. For each point we multiply these functions and calculate the area underneath this product. So let's do that:
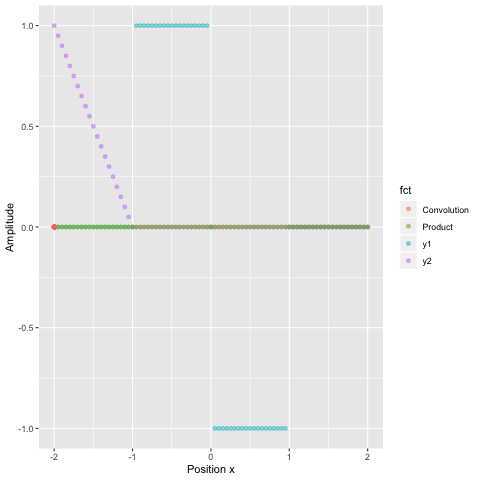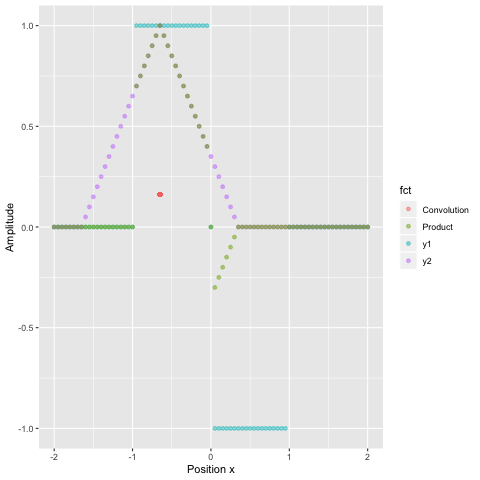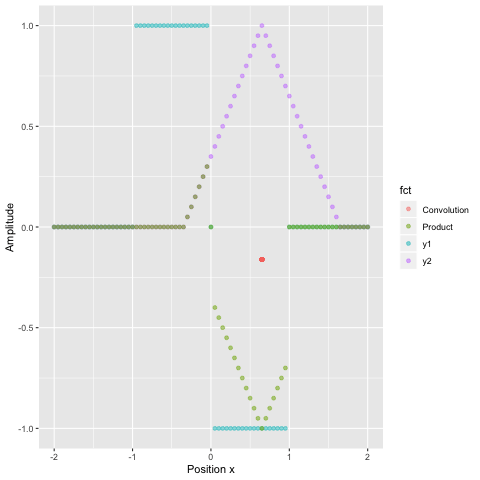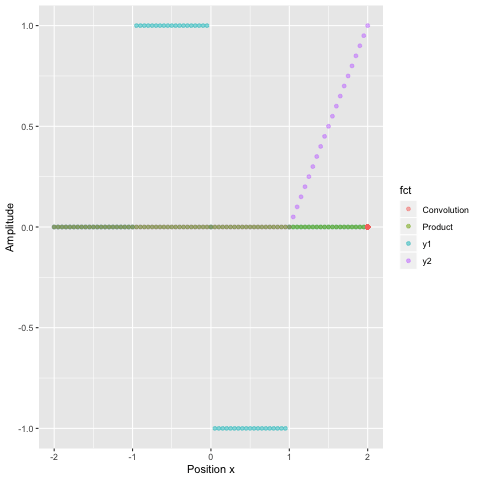
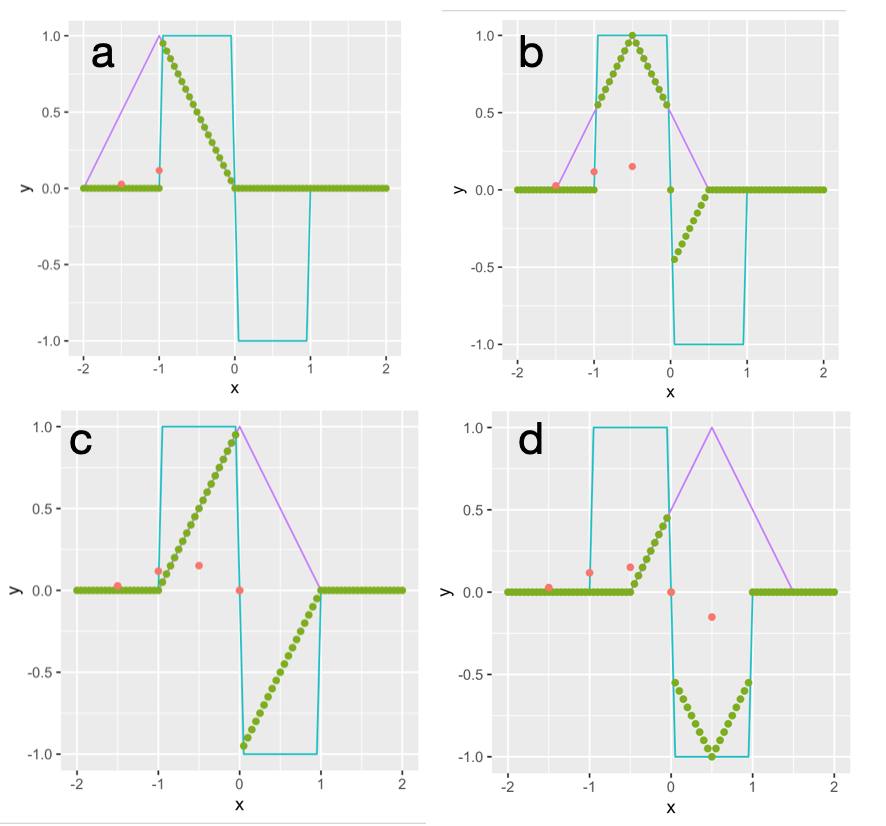
In green I plot the product. The red dot indicates the area underneath the product curve (I use the average of all the green curves in the pictures). I keep the red for previous positions of the triangle in the image, so that we can see a trend.
These images show that the triangular function acts as a weighted average of the "step" function. The triangular shape smoothes the steps and generates a fuzzy / blurred result. However, the important point (regarding your question) is that if we do not take a triangular but a different function for "smoothing" we obtain a different result. This is exactly why the double slit experiment for a rectangular and circular aperture generate different diffraction patterns.



