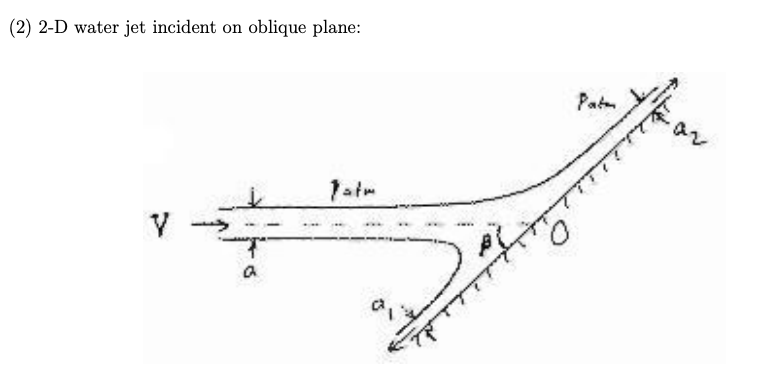
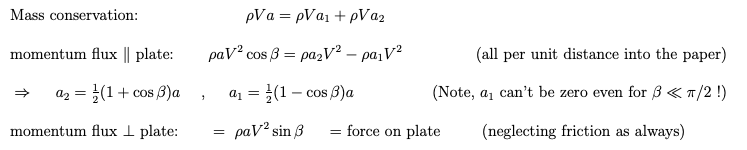We have a 2-D water jet on oblique plane. We are ignoring gravity and want to calculate the force on the plane. The explanation I have been given goes as follows. We assume inviscid flow. Image is attached.
Using Bernoulli's theorem on the free surface streamline: $$\frac{1}{2}V^2+\frac{p_{atm}}{\rho}=\frac{1}{2}U^2+\frac{p_{atm}}{\rho}$$ and so far enough along, we must have the flow velocity to be constant. We also have conservation of mass implying that $$Va=Va_1+Va_2$$ These two parts I understand. We then find the momentum flux parallel and perpendicular to the plate: parallel component of: $$\int_S \rho \underline{u}(\underline{u}.\underline{n})+p\underline{u} dS=0$$ where the other terms in the momentum equation disappear since we have steady flow and are neglecting gravity. We then say this is equal to $$\rho aV^2\cos(\beta)=\rho a_2V^2+\rho a_1V^2$$ We do a similar argument to find the perpendicular component of the momentum equation.
I am not sure about this part. Are we not neglecting the term from $$\int_S p\underline{u} dS$$ when finding the parallel component, if so why?
Here is the full derivation for reference: