If two bulbs are connected in parallel circuit with a variable resistor attached to the one of the branches and the resistance is decreased, what will be the effect on the brightness of the both bulbs? I don't understand how the brightness of the other bulb (the one with no resistor) is unaffected?
$\begingroup$
$\endgroup$
2
-
$\begingroup$ The brininess of a bulb should depend on the electrical power $P = U\cdot I$. If you change the resistor in one branch you affect the current in the other branch. So maybe I misunderstand your set-up. Could you add a drawing? $\endgroup$– NotMeCommented Feb 3, 2020 at 22:52
-
$\begingroup$ @Semoi, it's not necessarily true that changing the resistance one branch affects the current in the other branch, $\endgroup$– Alfred CentauriCommented Feb 4, 2020 at 1:37
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
It seems your circuit looks something like the following:
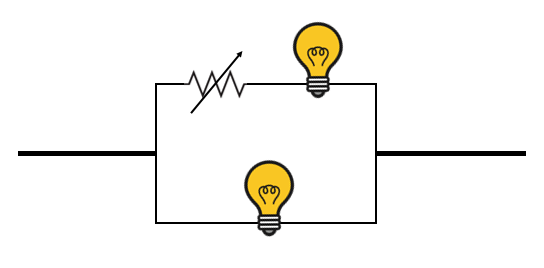
When the terminals of the above arrangement are maintained at a constant potential difference by some means (for example an ideal battery i.e., with zero internal resistance), the brightness of the bulb at the lower branch is independent of the value of resistance of the variable resistor at the upper branch. But, increasing or decreasing the resistance of the variable resistor affects the brightness of the bulb in the upper branch. When you increase the resistance, the current flowing through the upper branch reduces and hence the brightness of the bulb reduces. When the resistance is decreased, the current in the upper branch increases and so the upper bulb glows brighter.
I think this is the case you're looking for.
But, when the terminals are connected to a battery of significant internal resistance, then the potential difference across the terminals of the above arrangement vary which I hope you could work out with Ohms law or Kirchhoff's law or anything else as needed. Here brightness of both bulbs vary which depends on the amount of current which flows though each of them.
I'd recommend you to try the experiment yourselves in a laboratory or in an online simulator like this one.
If you still have any doubts, please ask in the comments.