I am currently studying the Wikipedia article for diffraction grating, and am having difficulty understanding some of the information in the theory of operation section of the article.
An idealised grating is made up of a set of slits of spacing $d$, that must be wider than the wavelength of interest to cause diffraction. Assuming a plane wave of monochromatic light of wavelength $\lambda$ with normal incidence (perpendicular to the grating), each slit in the grating acts as a quasi point-source from which light propagates in all directions (although this is typically limited to a hemisphere). After light interacts with the grating, the diffracted light is composed of the sum of interfering wave components emanating from each slit in the grating. At any given point in space through which diffracted light may pass, the path length to each slit in the grating varies. Since path length varies, generally, so do the phases of the waves at that point from each of the slits. Thus, they add or subtract from each other to create peaks and valleys through additive and destructive interference. When the path difference between the light from adjacent slits is equal to half the wavelength, $\dfrac{\lambda}{2}$, the waves are out of phase, and thus cancel each other to create points of minimum intensity. Similarly, when the path difference is $\lambda$, the phases add together and maxima occur. The maxima occur at angles $\theta_m$, which satisfy the relationship $d \sin(\theta_m) = |m|$, where $\theta_m$ is the angle between the diffracted ray and the grating's normal vector, and $d$ is the distance from the center of one slit to the center of the adjacent slit, and $m$ is an integer representing the propagation-mode of interest.
(By Vigneshdm1990 - Own work, CC BY-SA 4.0, https://commons.wikimedia.org/w/index.php?curid=58383485.)
In particular, I am having difficulty conceptually associating the type of interference explained in this excerpt with what I know about interference in waves.
My understanding is that the harmonic wavefunction is $\psi(x, t) = A \sin(kx - \omega t) = A \sin(\varphi)$, where $\varphi$ is the phase. Furthermore, my textbook, Optics, Fifth Edition, by Hecht, provides the following explanation and accompanying example of waves being "out-of-phase":
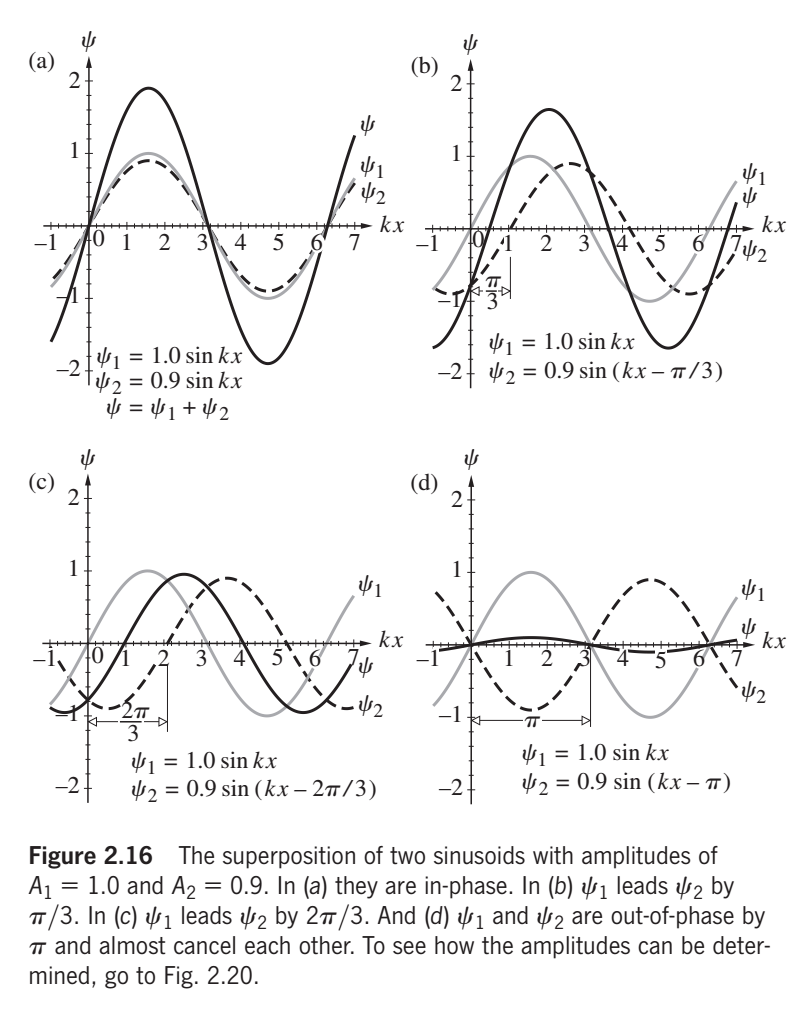
Developing the illustration a bit further, Fig. 2.16 shows how the resultant arising from the superposition of two nearly equal-amplitude waves depends on the phase-angle difference between them. In Fig. 2.16a the two constituent waves have the same phase; that is, their phase-angle difference is zero, and they are said to be in-phase; they rise and fall in-step, reinforcing each other. The composite wave, which then has a substantial amplitude, is sinusoidal with the same frequency and wavelength as the component waves (p. 293). Following the sequence of the drawings, we see that the resultant amplitude diminishes as the phase-angle difference increases until, in Fig. 2.16d, it almost vanishes when that difference equals $\pi$. The waves are then said to be $180^\circ$ out-of-phase. The fact that waves which are out-of-phase tend to diminish each other has given the name intereference to the whole phenomenon.
My questions concern this section:
When the path difference between the light from adjacent slits is equal to half the wavelength, $\dfrac{\lambda}{2}$, the waves are out of phase, and thus cancel each other to create points of minimum intensity. Similarly, when the path difference is $\lambda$, the phases add together and maxima occur.
What does the "path difference/length" have to do with the interference explained here? How does this relate to the interference that I learned from Hecht?
How does the path difference between the light from the adjacent slits being equal to half the wavelength mean that the waves are out of phase? Similarly, how does the path difference between the light from the adjacent slits being equal to the wavelength mean that the waves are out of phase? How does this relate to the interference that I learned from Hecht?
I would greatly appreciate it if people would please take the time to clarify these points of confusion.