4
$\begingroup$

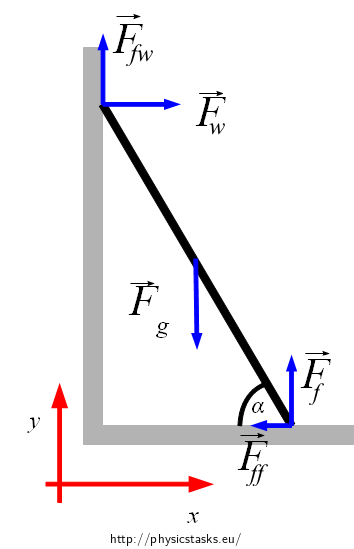
enter image description here

There is only one force acting on the ladder which is its weight and it acts vertically downwards. Then why does the normal contact force from the vertical wall act horizontally on the ladder? There must be a horizontal force acting on the wall to exert a horizontal force on the ladder. What causes the horizontal force on the wall and what is it called?

Improve this question
$\endgroup$
1
  • $\begingroup$ I have updated my answer to give you, what I think is, a more definitive explanation as to why there needs to be a horizontal reaction force at the wall. Hope it helps. $\endgroup$
    – Bob D
    Commented Dec 27, 2019 at 16:39

7 Answers 7

Reset to default
16
$\begingroup$

Think about how a ladder stands up in real life. Would the ladder stay in the orientation shown in your image if the ground were ice? No! The reason? Friction.

The friction force, represented by $\vec{F}_{ff}$ in the figure acts to prevent the ladder from sliding to the right.

There are actually 5 forces acting on this ladder:

  • $\vec{F}_g$: the gravitational force (aka the "weight" force), which pushes the ladder toward the ground
  • $\vec{F}_w$: The normal force of the wall on the ladder, which prevents the ladder from falling into the wall.
  • $\vec{F}_{fw}$: The friction force of the wall on the ladder, which prevents the ladder from sliding down the wall
  • $\vec{F}_f$: The normal force of the floor on the ladder, which prevents the ladder from falling through the ground.
  • $\vec{F}_{ff}$: The friction force of the floor on the ladder, which prevents the ladder from sliding to the right.
Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ I think your explanation in the first paragraph, while a correct intuitive explanation, does not explain why there is a need for a horizontal friction force when the only external forces acting on the ladder are vertical. I think that is what the OP is asking. $\endgroup$
    – Bob D
    Commented Dec 27, 2019 at 15:14
  • $\begingroup$ @BobD finally somebody who gets it ! Everyone taking it as granted without explaining it ! $\endgroup$
    – c.leblanc
    Commented Jun 20, 2023 at 21:35
4
$\begingroup$

There must be a horizontal force acting on the wall to exert a horizontal force on the ladder. What causes the horizontal force on the wall and what is it called?

Actually, there must be a horizontal force acting somewhere on the ladder to require an equal and opposite normal reaction force on the wall for equilibrium. That horizontal force acting on the ladder is the friction force at the base of the ladder. So what your question really boils down to is, why is there a friction force at the base of the ladder? @Bunji has given you an intuitive explanation. The following is in terms of the gravitational force acting on the ladder.

To answer that question note that any force can be resolved into mutually perpendicular components. Therefore $F_g$ can be resolved into two components, one acting down and parallel to the ladder, $F_{g}sinα$, and one perpendicular to the ladder, $F_{g}cosα$. At the base of the ladder, this force down and parallel to the ladder has a vertical downward component acting on the ground and a horizontal component acting on the ground to the right. Per Newton's third law these forces have equal and opposite reaction forces as shown in the free body diagram of the ladder at the base. One of those is the horizontal friction force acting to the left. For equilibrium you then need a horizontal reaction force on the wall for the sum of the horizontal forces on the ladder to be zero.

All of the above is intended only to explain the reason for a normal reaction force at the wall. Given that you now have 4 unknown reaction forces and one known force, $F_g$. So solve for the 4 unknowns, you need 4 equations. From here you should be able to identify the needed equations if you realize that the sum of the moments where the ladder contacts the ground and the wall have to be zero for equilibrium.

Hope this helps.

Improve this answer
$\endgroup$
3
$\begingroup$

I feel like there is something missing in this diagram, which is torque. In reality, there is a torque on the ladder, due to gravity, which causes it to want to rotate counterclockwise around the point where it touches the floor. This torque is "responsible" in some sense for the force of the top of the ladder against the wall (and the counterbalancing force of the foot of the ladder against the floor's friction.)

I don't see any torques in your free body diagram, although I do see an angle "alpha" at the base of the ladder, which is suggestive that maybe there should be some. If you haven't covered torque yet, this is not a great problem to try to work through.

Improve this answer
$\endgroup$
2
  • $\begingroup$ There is nothing missing in the diagram. The torque, or moment, that the gravitational force has about the points where the ladder contacts the ground and wall are taken into account when summing the moments about those points and setting them to zero for static equilibrium. The locations where the ladder contacts the wall and floor offer no moment reaction (like a hinge support on a simply supported beam has no moment reaction). $\endgroup$
    – Bob D
    Commented Dec 27, 2019 at 15:52
  • $\begingroup$ I think this answer is the only sound one, though it could be digged a bit further $\endgroup$
    – c.leblanc
    Commented Jun 20, 2023 at 21:39
1
$\begingroup$

Normal forces are always perpendicular to the direction of possible slipping since they do no work. Zero work means they must be perpendicular to any displacement or motion.

Since the ladder can slip downwards at the top, the only possible direction for the normal force is horizontally.

You can think of normal forces as enforcers of a specific constraint. In this case the ladder cannot interpenetrate through the wall. So a force that resist pushing into the wall must be perpendicular to the wall.

Improve this answer
$\endgroup$
0
$\begingroup$

Normal forces act perpendicular to the surface in contact. The force acting in the ladder is actually somewhere in between the horizontal and vertical forces shown. Those are just the components of the normal force.

Improve this answer
$\endgroup$
0
$\begingroup$
  1. The vertical force due to the weight creates a torque around the contact point between the ground and the ladder. At this point, nothing is generating a horizontal force. If the wall wasn't there, the ladder would simply rotate around the contact point (with or without friction).
  2. The wall prevents the ladder from rotating by applying a horizontal force (assuming there is no friction on the wall).
  3. Since there is a horizontal force, to achieve equilibrium, it must be countered by another force. The only possibility is the frictional force between the ground and the ladder. Therefore, if there is no friction there, the ladder will slide and fall.
Improve this answer
$\endgroup$
-1
$\begingroup$

Actually if you see the diagram , body is in rotational inertia, and rod is not perfectly vertical or horizontal, thing which work here is frictional force between rod and surfaces, friction always try to maintain the inertia, here the friction which acts is static friction.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ Hmmm.. I don't recall statics problems ever talking in terms of "rotational inertia" which is defined, as I understand it, as the measure of an object's resistance to change in rotation. The ladder is in static equilibrium and therefore does not, by definition. undergo rotation. If there is no rotation there is no resistance to a change in rotation. To prevent rotation, the sum of the moments anywhere on the ladder have to equal zero. $\endgroup$
    – Bob D
    Commented Dec 27, 2019 at 15:59

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.