0
$\begingroup$

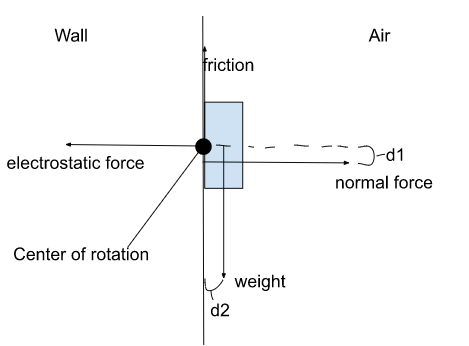
Say there's an object, a rectangular prism, that becomes electrically charged and then stuck on a wall using the electrostatic attraction between the object and the wall. The object and wall are pretty strong, rigid, and flat. The torque created with the vertical unalignment of the friction and weight forces means there's a torque created with a horizontal unalignment to counteract that (so the normal force acts mostly below where the electrostatic force is centered: Is the normal force greater below the center of mass than above?). This requires there to be some vertical length of contact between the object and wall, so I tried to find this length. Is my work right?

I know:
$F_N$=$F_E$
$F_g$=$F_F$
I take the midpoint (or line bc the scenario is 2D) of where the two surfaces are in contact to be the center for determining torque so I can balance them.

Let $d_1$ be the vertical distance between the center of the object and where the "main part" or "middle" of where the electrostatic force and normal force acts from.
Let $d_2$ be the horizontal distance between the middle of the object and where it meets the wall.

$T_1$=$T_2$
$d_1F_N$ = $d_2F_g$
$d_1F_N$ = $d_2F_F$
$d_1F_N$ = $d_2\mu$$F_N$
$d_1$ = $d_2\mu$

So the normal force is centered around a distance equal to the half of the horizontal length of the object times the coefficient of friction from below the vertical midpoint of where the object and wall are in contact (below the center of rotation I used). Therefore, the total vertical length of contact with the wall has to be more than twice that. It can't be that exactly, I believe, since half of the normal force has to be below where it is "centered."

Is this correct? Does this situation work how I am thinking it does? And if so, does this mean the strength of the electrostatic force has no effect? That doesn't seem right to me though, which makes me think I am doing something wrong.

enter image description here

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ I think your point of application of torque isn't really apt for this analysis, the object would more likely rotate about it's bottom most edge. Also without a drawing it's really unclear what all these distances are. $\endgroup$
    – Triatticus
    Commented Apr 13, 2023 at 18:06
  • $\begingroup$ Yea @Triatticus I just made a diagram. Why would the rotation be about it's bottom edge? $\endgroup$
    – Nathan Czopp
    Commented Apr 13, 2023 at 18:55
  • $\begingroup$ I suggest you remove the asterisks and write simply "$d_1F_N$" for example. The asterisks make it difficult (at least for me) to read. I was tempted to edit the question myself, but I thought that you might have a preference to have it as it is, and it is your question. $\endgroup$
    – garyp
    Commented Apr 15, 2023 at 12:35
  • $\begingroup$ Thanks @garyp I just removed them. $\endgroup$
    – Nathan Czopp
    Commented Apr 15, 2023 at 12:50

1 Answer 1

Reset to default
1
$\begingroup$

I think the point that you might be overlooking is that the $\mu F_N$ is the maximum friction force that the contact can support: the actual friction force can be less than that. $F_F=\mu F_N$ only when the object is on the verge of sliding downward.

There are two ways the object can fail to stay in equilibrium: it can either start sliding downward, or start rotating about its bottom left corner. The condition for the object to not slide is $$F_g=F_F\le\mu F_N=\mu F_E$$ $$F_E\ge\frac{F_g}{\mu}.$$ From torque balance, $$d_1 = \frac{d_2F_g}{F_E}=\frac{wF_g}{2F_E}$$ where $w=2d_2$ is the object width (assuming the object has uniform density). Since $d_1$ cannot be more than half of the object height $h$, the condition for the object to not rotate is $$\frac{wF_g}{2F_E}\le \frac{h}{2}$$ $$F_E\ge \frac{w}{h}F_g.$$ As you can see, the electrostatic force does matter: in fact static equilibrium imposes two different constraints on how small it can be, which can be summarized as

$$F_E\ge\max\left\{\frac{1}{\mu},\frac{w}{h}\right\}F_g.$$

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thanks this helps. You meant $w$=$d_2*2$ though right? And does this mean that the normal force acts mainly by the bottom edge of the object's contact with the wall (so it doesn't create torque)? $\endgroup$
    – Nathan Czopp
    Commented Apr 16, 2023 at 4:45
  • $\begingroup$ Yes, I meant $w=2d_2$, thanks. The effective location at which the normal force is applied (what you call $d_1$) depends on $w$, $F_g$ and $F_E$ (see the torque balance equation above). It acts on the corner ($d_1 = w/2$) only when the object is on the verge of "peeling off" from the surface and start rotating. Whether the torque due $F_N$ is zero depends on the point with respect to which you are calculating the torque. If you choose this point to be the middle of the contact surface, it is never zero. If it is the bottom left edge, the torque is zero only when the object is about to rotate. $\endgroup$
    – Puk
    Commented Apr 16, 2023 at 6:05

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.