TL;DR: On average, a lone atom (hydrogen or not) is truly nonpolar, producing no net electric field. This is not, however, necessarily true at any given instant, and the momentary generation of weak, transient electric fields by atoms (and nonpolar molecules) turns out to have very important consequences.
@EmilioPisanty's answer gets it right on a basic level - the negative charge of an atom (or molecule)'s electron cloud is distributed continuously over the whole electron cloud, rather than being concentrated in one (or more) discrete electron point charges.
It is also true that, averaged over time, the distribution of electric charge over the electron cloud of a lone atom1 is spherically symmetric, with no permanent net electric field being produced.
The electron cloud's charge distribution at a given instant, however, is not necessarily symmetric; there can be (and are) momentary clumps of more-strongly-charged electron cloud and of less-strongly-charged electron cloud.
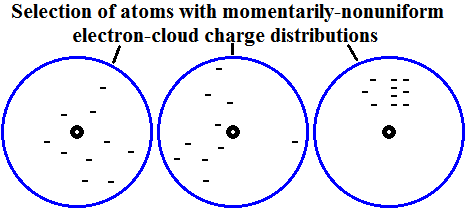
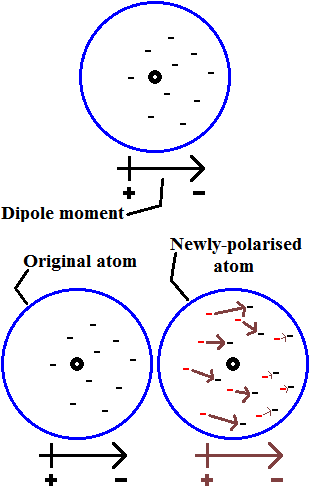
If an atom's electron cloud happens to momentarily have more of its negative charge on one side than on the other, this gives the atom a (weak, momentary) electric dipole moment, causing it to generate a (weak, momentary) electric field.
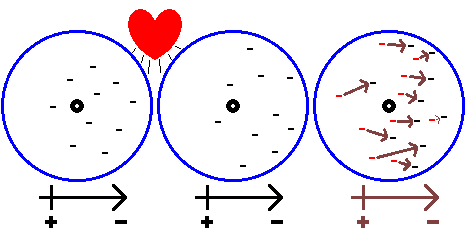
Although one might dismiss this as unimportant, it turns out to be anything but. If an atom with such a momentary dipole moment happens to come close to another atom in such a way that its positive or negative pole is pointing at (or nearly at) the second atom, it can then polarise the second atom.
We now have two polarised atoms, with dipole moments aligned head-to-tail. This creates an attraction, known as the London dispersion force, between said atoms; this is the weakest, but also the most ubiquitous, of the van der Waals forces mentioned by Emilio (and the only van der Waals force not requiring the participation of at least one polar molecule or ion).2 Additionally, as this atomic pairing-off does not cancel out the dipole moments of the two atoms, the pair can, in turn, polarise more atoms around them.
As atoms with more electrons - and, thus, more total charge - in their electron clouds are easier to polarise (especially when the polarising force is weak, like that from a random momentary atomic dipole in an adjacent atom), atoms (and molecules!) with more electrons exhibit stronger London dispersion forces, which is why things like iodine (a nonpolar diatomic molecule with lots of electrons, which is solid at room temperature) have higher freezing and boiling points than smaller nonpolar atoms and molecules, like helium (a nonpolar monatomic gas with only two electrons, which only liquifies when cooled to just above absolute zero).3
1: This train of thought also works for nonpolar molecules, which, likewise, generate no permanent net electric field, but these wouldn't be accurately described as "spherically symmetric".
2: The other two are the Coulomb/Keesom force between permanently-polar molecules (the strongest of the van der Waals forces, but also the least common, as it requires that both participants be molecules with permanent electric dipole moments) and the Debye force between a permanently-polar molecule (on the one hand) and an ordinarily-nonpolar atom or molecule polarised by the electric field of the permanently-polar molecule (on the other).
3: For nonpolar molecules with more than two atoms and containing atoms from at least two elements, an additional method of generating momentary charge separation comes into play; if the thermal flexions and stretchings and vibrations of the atoms in the molecule happen to put most of the atoms of element A on one side of the molecule, and most of the atoms of element B on the other side, this generates a momentary dipole moment even if the charge distributions within each of these atoms' electron clouds remain perfectly symmetrical. This is why (for instance), even though a neon atom and a methane molecule each have ten electrons, neon boils at 27.1 K at standard pressure, while methane's boiling point is a toasty 111.65 K.




