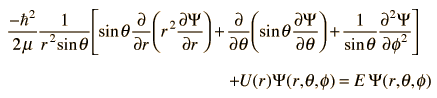During the approach of an electron and a proton into a hydrogen atom, are their electric fields unchanged? Or do they cancel each over out (more or less, only a weak electric dipole alive).
$\begingroup$
$\endgroup$
7
-
$\begingroup$ How and why would they change? Do you mean have experiments been done to measure them? $\endgroup$– user108787Commented Sep 24, 2016 at 18:12
-
1$\begingroup$ I think that the magnetic dipole moments of the electrons are responsible for the shells with 2, 8, 8, ... electrons and the Pauli exclusion principle (academia.edu/18391675/…). And that protons (together with neutrons) are able to be in a nucleus happens do to the cancelling out the electric fields of the involved electrons and protons (academia.edu/19657550/…). But in my question I simple want to know is the cancellation is taken in account. $\endgroup$– HolgerFiedlerCommented Sep 24, 2016 at 18:25
-
$\begingroup$ Congratulations on the papers, they read extremely well, Can you not include a reference to them in your post? If I had known about them, I would not have asked you the otherwise "simple" question. Are you allowed to put the abstracts in your post? $\endgroup$– user108787Commented Sep 24, 2016 at 18:47
-
1$\begingroup$ @CountTo10 The facts are "on the hand", but the conclusions are not mainstream, so due to the really high quality if physics.stackexchange I'm not sure that it would be good to do what you propose. $\endgroup$– HolgerFiedlerCommented Sep 24, 2016 at 18:51
-
$\begingroup$ The shell structure is well described with vanilla quantum mechanics, and the Pauli Exclusion principle is predicted by the Spin Statistics theorem which also explains why bosons are not subject to that limit. $\endgroup$– dmckee --- ex-moderator kittenCommented Sep 24, 2016 at 19:00
|
Show 2 more comments
1 Answer
$\begingroup$
$\endgroup$
2
The hydrogen atom is analytically solvable in the Schrödinger equation
U(r) is the potential and the solution of the equation exists for all r. If the energy is positive it is a free electron and a free proton, if it is negative it has to be in a quantized state.
-
$\begingroup$ U is the potential energy? $\endgroup$ Commented Sep 24, 2016 at 18:28
-
$\begingroup$ in the case of the simple electron-proton field it is the electric potential ~1/r users.aber.ac.uk/ruw/teach/237/hatom.php $\endgroup$– anna vCommented Sep 25, 2016 at 3:52