From Hecht optics 5th edition:
It says 'With an extended source, the symmetry of the setup requires that the interference pattern consists of a series of concentric circular bands centered on the perpendicular drawn from the eye to the film'
Why is that so? What does the 'extended' source do and why isn't it any other shape like an ellipse? Thank you.
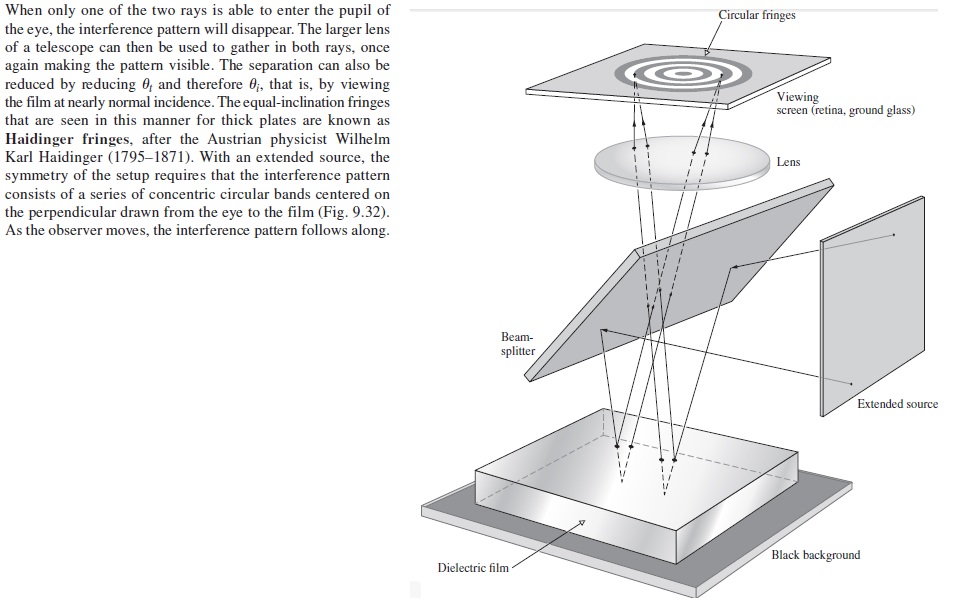
This type of interference is called division of amplitude as opposed to division of wavefront which is applicable to Young's slits.
If there was a point source $S$ then the ray diagram for the arrangement described in your question would look something like this and I have used the labels that Hecht uses.
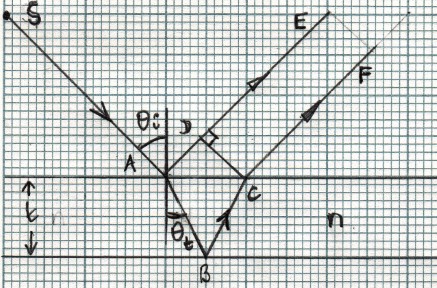
Ray $SA$ is reflected from the top surface at $A$ and also from the bottom surface at $B$ resulting in parallel reflected ray $AE$ and $CF$ which will superpose at infinity (or in the focal plane of a lens).
The optical path difference can be shown to be $2\,n\,t\,cos \theta_{\rm t} + \frac \lambda 2$ the last term being due to a $\pi$ phase change at one of the reflections.
If the optical path difference is equal to $m\,\lambda$, where $m$ is an integer, then the waves will arrive in phase - constructive interference.
Now imagine that there is a point source $S_2$ close to point source $S_1$ and a lens is used to focus the parallel rays in the focal plane of the lens at $Z$.
The condition for constructive interference is the same for light which start off from point source $S_1$ as it is for point source $S_2$ as the angle $\theta_{\rm t}$ is the same - say constructive interference.
Now consider another two point sources the mirror images about line $XX'$ on the right hand side.
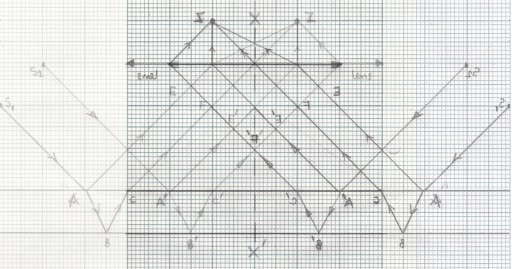
Those sources backward $S_1$ and backward $S_2$ will now produce constructive interference at backward $Z$.
Now consider a rotation of the diagram above about the line $XX'$ and you obtain the diagram from Hecht - a circular fringe.
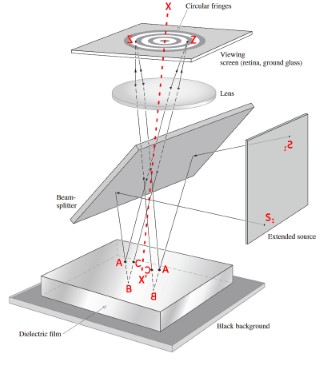
Each bright fringe is characterised by the angle $\theta_{\rm t}$ (and $\theta _{\rm i}$) being the same and so the fringes are called fringes of equal inclination. The range of angles $\theta_{\rm i}$ is provided for in this arrangement by using an extended source.
There is an arrangement which in principle is similar to this one with two reflecting surfaces (mirrors - one real and one virtual) separated by air and so there is not the added complication of refraction occurring.
It is the Michelson interferometer producing circular fringes.
It already tells you with the "symmetry of the setup".
If it were an ellipse then there would have to be an azimuthal asymetry in the setup, but there is not. You have circular rings because the path length difference is a function of r only.
The extended source provides plane wave illumination. As a lens acts as a Fourier transform, the source focuses to a point without any object to interfere with. Other sources would not, thus blurring out the effect you are trying to see.
