Understanding the notion of lose or win of angular momentum in accretion disk

Question

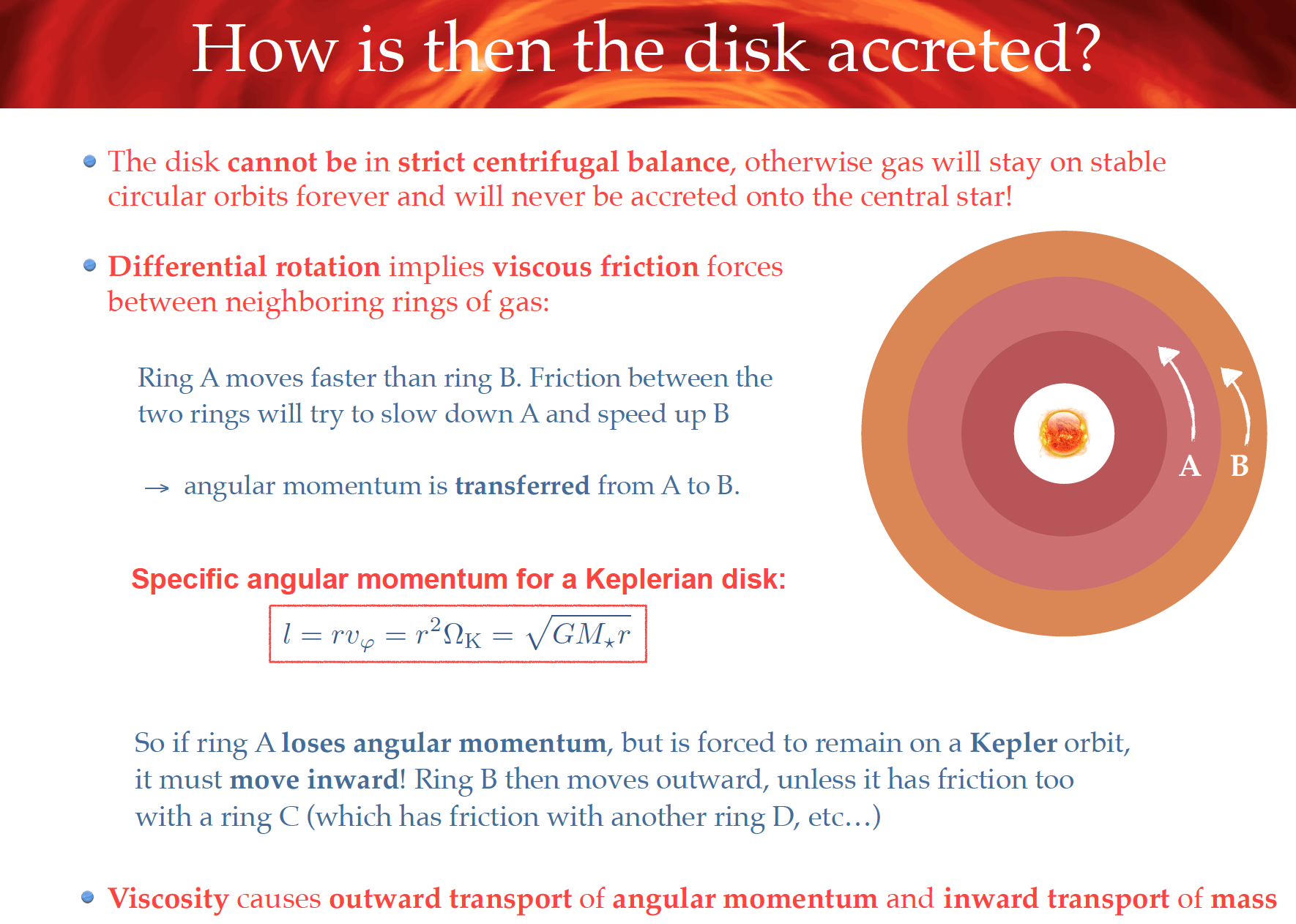
Here is below a slide of one of my lecture in planetology :

I understand well the fact that ring A will be slow down by friction with ring B since ring B is rotating slower and the inverse process (ring B will be speed up by ring A).

Question 1) But what does mean "but is forced to remain on a Kepler orbit" under the formula of angular momentum.

Question 2) Does it mean that if $v_{\phi}$ is lower , then it moves outwards ($r$ is increasing) and if $v_{\phi}$ is higher, it moves inwards ($r$ is decreasing) ?

But this is the contrary of what it is said ?

Question 3) When we say, "if ring A loses angular momentum", does it mean that "ring B wins "angular momentum" (I assume so the total conservation of angular omentum) ?

Question 4) If ring B wins angular momentum, that's why dust or gas goes outwards ($\Delta r > 0$ into $L=\sqrt{GM_{\star}r}$)?

UPDATE 1 : Concerning the fact that mass of ring A is falling at a lower orbit ($r<r_{A}$) is due to $v_{\phi}$ remains constant but angular maomentum $L$ is descreasing from its definition :

$L = m\,v_{\phi}r$

On the orher side, ring B wins angular momentum and so with also $v_{\phi}$ constant, mass of ring B tends to go at a higher orbit.

My mais issue is in both cases the notion of "$v_{\phi}$ remains constant" to explain the inner and outter motion of ring mass.

Regards

Answer 1

For Q1, the Keplerian orbit in the sentence you refer to is a hypothetical, not a consequence. If it loses angular momentum and if it remains in a Keplerian orbit then the orbit must have a smaller radius. This follows from the equation.

Q2. Note the the slide doesn't say that A slows down and B speeds up. It says that friction tries to slow down A and tries to speed up B. But if this process is done slowly and continuously, that doesn't happen. As the energy and angular momentum is pulled from A, the orbit lowers in such a way that the speed increases. At no time does A actually slow down.

Q3. I'd prefer the term "added" or "gained", but yes. Any angular momentum lost by A is gained by B. The sum remains constant.

Q4. Correct.

U1. Why do you ask about $v_\phi$ being constant? It's not. As the ring contracts, it speeds up. As it enlarges, it slows down.

I have difficulties with "tries to slow down A and tries to speed up B" and saying nothing of both occur : So how the lose or win of angular momentum can be acheived ?

Because speed isn't directly related to the angular momentum. There is an interaction (viscous friction in the slide), and this might initially cause a slight slowdown in the particles. But the orbit of the particles changes as well and ends up speeding them up (by more than the friction slowed them down).

The inner ring loses angular momentum and gains orbital speed at the same time.

You indicated that Q4 was correct : can we say the same thing for inward motion of mass : if ring A loses angular momentum, there will be an inward motion of mass from ring A to a lower orbit ?

Yes. We're assuming the ring has a particular mass that is roughly constant. So the only change in $\sqrt{GMr}$ is the radius (which is decreasing).

Answer 2

1. An object with an angular momentum $L$ with respect to the central object tends to follow a circular orbit with a Keplerian angular frequency $\Omega$ such that the gravitational attraction is balanced by the radially directed centrifugal force. If an object loses a part of its angular momentum, and is hence too slow for its current orbit. It will move to a similar orbit of a lower radius.

2. This premise is valid (by conservation of angular momentum) when the body stays in the same orbit, like when the earth moves around in an elliptical orbit around the sun. This is not applicable here since the "ring" moves to a different Keplerian orbit (because it lost its angular momentum).

3. Yes. Ring B gains (or "wins") the angular momentum lost by Ring A and there would be a Ring C which would in turn win the angular momentum from Ring B.

4. Quite correct. As stated above the rings would transport their angular momentum to the rings directly above them in the orbit and the outermost rings will probably diffuse into the interstellar medium, carrying all the lost angular momentum.

I would like to add a few more points.

Since the ring which moves inwards moves closer to the central body, a part of its gravitational energy is converted into kinetic energy. Thus, the disk loses angular momentum but gains kinetic energy, which keeps the process going.

Your understanding about the inner ring slowing down and the outer ring speeding up is correct, but, this is not mediated by a process as simple as molecular friction. This effect is attributed to various causes such as presence of a magnetic field which gives rise to something called the 'Magnetorotational Instability' among other models, and is in fact an active field of research.

Answer 3

This answer does not explain the method of angular momentum transfer etc rather it uses simple orbital mechanics to try and explain the angular momentum and energy changes involved when orbits change.

Assuming circular orbits then applying Newton's second law $\dfrac{GMm}{R^2} = m\dfrac{v^2}{R} \Rightarrow v^2 = \dfrac{GM}{R}$
So for a particular value of the radius of the orbit $R$ the speed of the satellite $v$ has to be a value given by the equation above.

This may seem a strange result in that when a satellite is acted on by frictional forces its orbit $R$ is reduced and yet its kinetic energy $\dfrac 12 mv^2$ increases.
However in such a situation the total energy of a satellite is the sum of the kinetic energy and the gravitational potential energy, $E_{\rm total} =\dfrac 12 m v^2 - \dfrac {GMm}{R}=-\dfrac{GMm}{2R}$, and if the orbital radius decreases the total energy of the satellite also decreases - becomes more negative.
The difference being the work done by the frictional forces on the satellite.

After substituting for $v$ the angular momentum $L = mvR = m\sqrt{GMR}$ which decreases as the radius of the orbit decreases.
Your notes use the specific (meaning divided by mass) angular momentum $l = \dfrac{mvR}{m} = \sqrt{GMR}$

---

To give you an idea of what might be happening in an accretion disc assume a mass $2m$ in orbit at a radius $a$ with angular momentum $2m\sqrt{GMa}$ and total energy $-\dfrac{GMm}{a}$.
In some way mass $m$ moves to a lower orbit $b(<a)$ and mass $m$ moves to a higher orbit $c(>a)$ with the angular momentum conserved.

$2m\sqrt{GMa} = m\sqrt{GMb} + m\sqrt{GMc}\Rightarrow 2\sqrt{a} = \sqrt{b} + \sqrt{c}$

The total energy of the system of two masses is now $-\dfrac{GMm}{2b} -\dfrac{GMm}{2c}$ having been $-\dfrac{GMm}{a}$ before the separation of the masses.

In terms of energy changes one has to compare $-\dfrac 1a$ with $-\dfrac {1}{2b} -\dfrac {1}{2c} $ which I will do with a numerical example - think of this as setting $GMm=1$.

Assume $a=1$ and $b=0.99$ then using the conservation of angular momentum gives $c \approx 1.01$

The total energy before separation is $-\dfrac11 = -1 $ and after separation the total energy is $-\dfrac {1}{2\times 0.99}- \dfrac {1}{2\times 1.01}\approx -1.0001$.

So there is a loss of mechanical energy due to the complex mechanism of interaction within an accretion disc which transfers angular momentum between various parts of the disc.

Answer 4

This is an interesting observation. The solar system is assumed to be formed from a primordial nebula. But the outer planets of our Solar system are not only moving the same way but have almost equal angular momenta. This includes the Sun spinning on its axis. Why it is so is not clear.The order of magnitude is e^43. Seems to be an equipartition of angular momentum. Is it due to collisions, sticking, gravity and friction or absence of any of them excluding gravity of course?

The data is from http://www.zipcon.net/~swhite/docs/astronomy/Angular_Momentum.html

Orbital Angular Momentum Body orbital radius (km) orbital period (days) mass (kg) L Mercury 58.e6 87.97 3.30e23 9.1e38

Venus 108.e6 224.70 4.87e24 1.8e40

Earth 150.e6 365.26 5.97e24 2.7e40

Mars 228.e6 686.98 6.42e23 3.5e39

Jupiter 778.e6 4332.71 1.90e27 1.9e43

Saturn 1429.e6 10759.50 5.68e26 7.8e42

Uranus 2871.e6 30685.00 8.68e25 1.7e42

Neptune 4504.e6 60190.00 1.02e26 2.5e42

Pluto 5914.e6 90800 1.27e22 3.6e38 3.1e43

Moon 384e3 27.32 7.35e22 2.9e34

Io 422e3 1.77 8.93e22 6.5e35

Europa 671e3 3.55 4.80e22 4.4e35

Ganymede 1070e3 7.15 1.48e23 1.7e36

Callisto 1883e3 16.69 1.08e23 1.7e36

and Rotational Angular Momentum (spin)

Body radius (km) rotational period (days) mass (kg) L

Sun 695000 24.6 1.99e30 1.1e42

Earth 6378 0.99 5.97e24 7.1e33

Jupiter 71492 0.41 1.90e27 6.9e38