Why isn't it possible to focus a laser beam to an infinitely small point in space? I am familiar with the shape of a gaussian beam, but why can't my $w_0$ be equal to zero?
$\begingroup$
$\endgroup$
4
-
$\begingroup$ Related/possible duplicates: physics.stackexchange.com/q/234996/50583, physics.stackexchange.com/q/421584/50583, physics.stackexchange.com/q/140949/50583 $\endgroup$– ACuriousMind ♦Commented Mar 14, 2019 at 20:07
-
$\begingroup$ I am sorry, but non of these answer my question. $\endgroup$– Timo_BLNCommented Mar 14, 2019 at 20:08
-
$\begingroup$ The answer is diffraction. $\endgroup$– GilbertCommented Mar 14, 2019 at 20:16
-
2$\begingroup$ It's amazing how the two current answers to this question, by Thomas Fritsch and @ACuriousMind, answer the question with the same underlying principle approached from two entirely different sides. Thomas Fritsch explains it from the mathematical model, with the result that you'd need a wavelength of $0$ to have a beam with a width of $0$, and ACuriousMind states that at the most extreme case, you'd have only a single wave, which logically would be bounded in width by wavelength and amplitude, thus requiring the same wavelength of $0$. This is why I love physics. $\endgroup$– TheEnvironmentalistCommented Mar 14, 2019 at 20:42
Add a comment
|
4 Answers
$\begingroup$
$\endgroup$
2
An ideal Gaußian beam is diffraction-limited - its wavefront inevitably spreads out due to Huygens' principle. An infinitesimally small beam would diffract infinitely strongly, i.e. not resemble a beam at all: By Huygens' principle a single point (i.e. a "beam source" with zero radius) as a source simply emits a single, spherical wave, not a beam.
answered Mar 14, 2019 at 20:29
-
$\begingroup$ This does not explain why you cannot focus to a point though. $\endgroup$ Commented Mar 14, 2019 at 22:05
-
$\begingroup$ @PaulChilds I linked three other questions about focusing to a point vs. conservation of etendue in the comments to the question, and the OP said they didn't answer their question, so I didn't see the point of explaining that yet again. $\endgroup$– ACuriousMind ♦Commented Mar 14, 2019 at 22:15
$\begingroup$
$\endgroup$
3
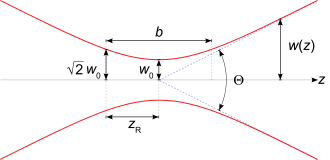
According to Wikipedia:Gaussian beam the beam waist ($w_0$) and the total angular spread of the beam ($\Theta$) are related by
$$ \pi w_0 \tan \frac \Theta 2 = \lambda $$
From this formula you see: The wave-nature of light (via its wavelength $\lambda$) is responsible for the non-zero waist.
That means you would have a beam waist $w_0 = 0$ only for these cases:
- wavelength $\lambda = 0$ (so that there is no diffraction)
- total angular spread $\Theta = 180°$ (meaning we have a spherical wave from a point source)
answered Mar 14, 2019 at 20:26
-
1
-
$\begingroup$ I think we can assume that the wavelength is not zero in which case the only non-trivial solution is $\Theta = 0$ as The Photon has said. $\endgroup$ Commented Mar 14, 2019 at 22:02
-
1$\begingroup$ @ThePhoton correct, I've updated my answer $\endgroup$ Commented Mar 14, 2019 at 22:14
$\begingroup$
$\endgroup$
The Gaussian beam is not a precise solution of the Maxwell equation, it can only derived in the paraxial approximation, and this approximation is not applicable for small $w_0$.
$\begingroup$
$\endgroup$
7
Ok, I'm not happy with the answers so far so here's my 2 cents.
Yes $\omega_0$ can be zero. If you have a perfectly spherical mirror with a single particle lasing source inside, then by classical optics it will focus light to a infinitely narrow point at the centre.
The real reason then why it is not truly infinitely narrow (manufacturing defects aside) is that even if we are to ignore entanglement re: the photon and assume it to not ne governed by the exclusion principle, the emitter will be, and cannot be isolated to a infinitely narrow non-moving position.
-
$\begingroup$ Thank you, that was the answer I was looking for! $\endgroup$– Timo_BLNCommented Mar 14, 2019 at 22:17
-
$\begingroup$ That answer is not correct. It is possible to cause a single atom in a crystal to emit light, and to know that the atom is localized to within a fraction of a nanometer, but it is not possible to focus the emitted light back to a region the same size. $\endgroup$ Commented Mar 15, 2019 at 1:35
-
$\begingroup$ How does that explain why it is incorrect? Uncertainty in location and momentum cause respective spatial and temporal decoherences. These will govern how tightly you can focus light. $\endgroup$ Commented Mar 15, 2019 at 1:54
-
$\begingroup$ This answer is wrong. You can't focus light into a single point, even if you try to describe it as a classical wave. There is no classical solution to the wave equation with an abitrary small focus-size. $\endgroup$ Commented Mar 30, 2021 at 18:53
-
$\begingroup$ @Quantumwhisp please tell me then what is the classical solution for ω_0 in my example. $\endgroup$ Commented Mar 31, 2021 at 0:04