In this diagram of a Gaussian beam, why does the radius vary with z position? Is it assumed that a Gaussian beam is always being focus by a lense? Why can the beam not go any smaller than $w_0$ and how is $w_0$ calculated?
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
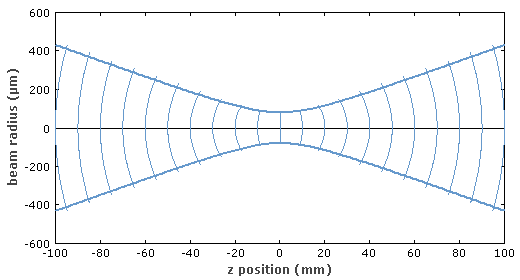
A Gaussian beam has a width that changes with distance because of diffraction, which is an effect that takes place in any wave phenomenon. It has a pretty similar description to the Heisenberg uncertainty principle in QM if you're familiar with that. Namely, as the position in the $x$ and $y$ directions (with the optical axis pointing in the $z$ direction) becomes very well defined, the spatial frequencies (basically the same thing as momentum) in the $x$ and $y$ directions become very ill-defined. This means the tighter you focus a beam, the more it will tend to spread. So if you do anything to try to converge a Gaussian beam to a point, diffraction will compete against you and you're left with some non-zero smallest spot size.
It doesn't have anything to do with the specifics of how you make the beam narrower (e.g. lensing), but is just a consequence of wave optics. If you'd like to see the math behind this, take a look at the paraxial wave equation which describes waves mostly travelling in 1 direction, such as a Gaussian beam in the $z$ direction. You'll find there are no solutions to that equation with zero beam width except for the trivial solution where the amplitude is zero everywhere.
