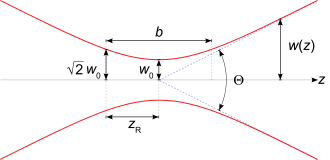
According to Wikipedia:Gaussian beam the beam waist ($w_0$) and the total angular spread of the beam ($\Theta$) are related by
$$ \pi w_0 \tan \frac \Theta 2 = \lambda $$
From this formula you see: The wave-nature of light (via its wavelength $\lambda$) is responsible for the non-zero waist.
That means you would have a beam waist $w_0 = 0$ only for these cases:
- wavelength $\lambda = 0$ (so that there is no diffraction)
- total angular spread $\Theta = 180°$ (meaning we have a spherical wave from a point source)