Let's consider a very tall building (g would be variable) which is rotating with the earth naturally. Now do the centrifugal force and the gravitational force act on the same spot? If not why does the center of gravity change from the center of mass in a system that has non-uniform gravity throughout?
$\begingroup$
$\endgroup$
1
-
1$\begingroup$ Possible duplicates: physics.stackexchange.com/q/50107/2451 , physics.stackexchange.com/q/151402/2451 and links therein. $\endgroup$– Qmechanic ♦Commented Jan 15, 2019 at 11:52
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
Center of Mass as a concept was developed from Center of Gravity, when the disuniformity of the gravity field was unknown. Center of mass (point you can push an object without it starting to rotate (no other forces acting)) is not equal to center of gravity (point where you would have to support an object to counteract gravity) if you have an object that is not symmetrical about the focus of the gravity field.
$\begingroup$
$\endgroup$
Think of the centre-of-mass as a "weighed" geometric centre. It is just the entire object's mass "averaged down" to one point: $$r_\text{CoM}=\frac{\sum rm}{\sum m}$$
The centre-of-gravity on the other hand is the "averaged down" pull from gravity. If gravity pulls equally in each particle, then the total pull "averages out" to the "weighed" geometric centre, so it is the same as the centre-of-mass.
But imagine that gravity of some reason only pulls in the bottom half and not the top half. Then the total pull doesn't "average out" to the centre of this object - instead it "averages out" to some point in the bottom half. So, it does not coincide with the centre-of-mass in this case. Whenever gravity is non-uniform throughout the object, this may be the case (unless the symmetry of this non-uniform gravitational pull happens to make them coincide again).
$\begingroup$
$\endgroup$
To answer your question fully is very difficult so I will make some assumptions to start with.
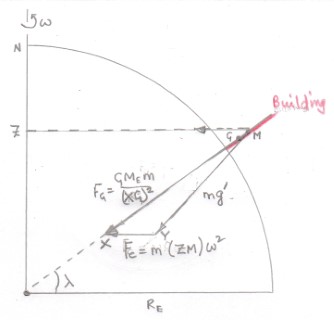
Assume that the Earth is a rotating sphere with angular speed about the $NS$ axis $\omega$, that its mass ii $M_{\rm E}$ and it has a radius $R_{\rm E}$.
Let the tall building be of uniform composition and that its centre of mass is at position $M$ as shown in the diagram below.
The centre of gravity of the tall building is at position $G$ and the building has a mass $m$.
The answers here and the links within explain the reason for the centre of mass and the centre of gravity not being at the same position in a non-uniform gravitational field.
The gravitation force on the tall building is $F_{\rm G} = \dfrac{GM_{\rm E}m}{(XG)^2}$ and the line of action of this force acts through the centre of mass of the tall building.
That gravitational force can be resolved into two components $F_{\rm C}$ which provides the centripetal acceleration of the tall building $ \omega (ZM)^2$ and $mg'$ where the line of action of $g'$ does not go through the centre of the Earth.
I have purposely not introduced pseudo forces and totally ignored the stability of the building ie omitted the force (and torques) on the building due to it being in contact with the Earth.
The analysis becomes much easier if the building is very close to the Earth so that the magnitude of the gravitation field of the Earth in the region of the tall building is constant.
You could then say that the position of its centre of gravity $G$ is, to a very good approximation, the same as its position of the centre of mass.
