1
$\begingroup$

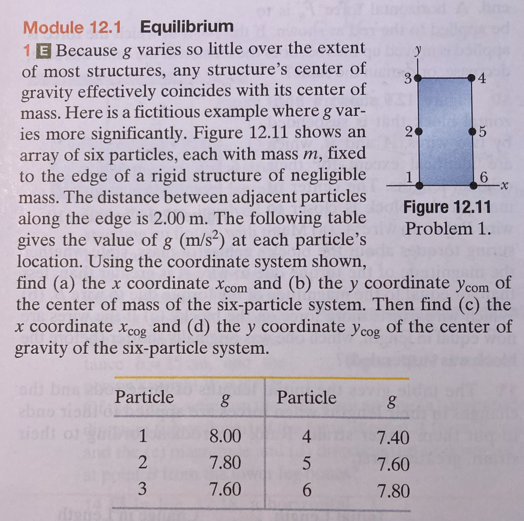
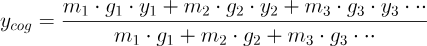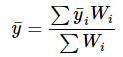The following is a textbook problem where one is asked to find the $x$ and $y$ coordinates of the center of mass AND those of the center of gravity in a non-uniform gravitational field:

enter image description here

The location of the center of mass and the x-coordinate of the center of gravity are trivial, but I am having trouble with the y-coordinate of the center of gravity.

A solution I found online shows this:

enter image description here

Which yields 1.97 meters, which agrees with the answer given in the textbook.

This is intuitive, but at the same time I don't understand where this equation is coming from, or what the exact definition of center of gravity means. If it is the point where the net force can act on an extended object and create the same net torque, then any location with the x-coordinate of the center of gravity should work, since the force points down.

If I rotate the object clockwise by 90 degrees, and find the location where the net force will create the same net torque as all the individual torques on each mass, then, yes, I get the same answer, but, the point is that the gravitational field is not uniform, thus it will depend on the position of the masses, and since those have changed, so most likely have the gravitational fields each mass feels, since they are at a different position.

Improve this question
$\endgroup$
4
  • 2
    $\begingroup$ See Eq 7.2.1 of eng.libretexts.org/Bookshelves/Mechanical_Engineering/… $\endgroup$
    – Andrew
    Commented Aug 6, 2023 at 19:58
  • 2
    $\begingroup$ Just some advice: If your entire question is just, "what's the definition of center of gravity", you don't need to include the information about the specific HW problem that prompted the question. It's generally best to keep questions reasonably concise, only including the information that's actually necessary to explain what it is you want help understanding. It took me some time to realize this myself when I was a new contributor to the site, and I started getting many more answers after I made it a point to cut down on the fluff and unnecessary background info in my questions. $\endgroup$
    – Mikayla Eckel Cifrese
    Commented Aug 6, 2023 at 20:29
  • 1
    $\begingroup$ Thank you @Andrew for the link, it was quite helpful. $\endgroup$
    – raphael
    Commented Aug 7, 2023 at 18:34
  • 1
    $\begingroup$ Thank you @MikaylaEckelCifrese for your advice, which I plan to take for any future questions I post. $\endgroup$
    – raphael
    Commented Aug 7, 2023 at 18:35

2 Answers 2

Reset to default
1
$\begingroup$

The center of mass is the first moment of the density distribution, while the center of gravity is the 1st moment of the weight distribution, that is: the mass density distribution weighted bby $\vec g(\vec r)$.

In your problem, $\vec g(\vec r)$ is factorable into $g(x, y)\hat y$ (maybe), so its solvable.

Though I'm not sure that $\phi$ satisfies Poisson Eqs....

Improve this answer
$\endgroup$
1
$\begingroup$

Although the question has been answered to my satisfaction, I wanted to add some notes that I think would be helpful. Unlike center-of-mass, center-of-gravity is much more complicated.

According to this Wikipedia entry, there are different methods for formulating the center-of-gravity.

Textbooks such as The Feynman Lectures on Physics characterize the center of gravity as a point about which there is no torque. One complication concerning $ r_{cg} $ is that its defining equation is not generally solvable.

In the specific situation of the problem I posted, I was using the only method the textbook provided, which was finding the location of the force that would satisfy the condition that it would produce the same torque as the sum of the torques on all the individual particles. This leads to infinite locations along the vertical axis. From same Wikipedia article:

If the equation is solvable, there is another complication: its solutions are not unique. Instead, there are infinitely many solutions; the set of all solutions is known as the line of action of the force. This line is parallel to the weight F. In general, there is no way to choose a particular point as the unique center of gravity.

However, as I think I see now, in the problem I was working on (same Wikipedia article):

A single point may still be chosen in some special cases, such as if the gravitational field is parallel or spherically symmetric.

The field of the problem is parallel (straight down). Thus the equation given in the link of provided by @Andrew (for the y-axis) works:

enter image description here

Improve this answer
$\endgroup$
4
  • 1
    $\begingroup$ In the general case, the field isn't parallel, so you're finding the 1st (vector) moment of a scalar distribution weighted by a vector. Not sure how that works. $\endgroup$
    – JEB
    Commented Aug 8, 2023 at 14:37
  • $\begingroup$ @JEB thank you for the answer. Poisson equations for now are beyond me, since it's been 30 years since I've done partial differential equations. I also understand that a non-uniform gravitational field cannot be truly parallel. In these respects, the textbook problem is oversimplified in order to get a certain point accross. I don't know. But I'm not sure what you mean by "Not sure how that works". I will do the research, but if you can tell me if I'm interpreting your answer incorrectly, please let me know. Thank you again! $\endgroup$
    – raphael
    Commented Aug 8, 2023 at 20:02
  • 1
    $\begingroup$ In newtonian terms, a parallel g-field only comes from an infinite sheet of mass (it's a standard problem treated all over this site), and in relativity is comes from uniform accelerations (Rindler Coordinates). For the infinite sheet, there's no center of gravity or mass because all points are equivalent, so the field is perpendicular to the sheet (it's also doesn't depend on distance...which makes sense because the only way to get weaker without divergence (Poisson's Eq) is by spreading out, but it can't spread out , b/c it's parallel) $\endgroup$
    – JEB
    Commented Aug 8, 2023 at 23:16
  • $\begingroup$ This clears things up a lot for me. Thanks, @JEB for the time and effort spent! $\endgroup$
    – raphael
    Commented Aug 9, 2023 at 16:16

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.