1
$\begingroup$

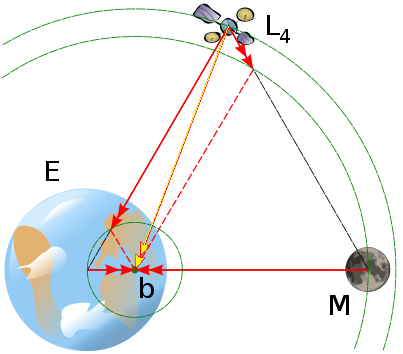
I am aware of the fact that Lagrange points $L_4$ and $L_5$ are stable equilibrium points however I cannot understand why they are equilibrium points in the first place.

Consider a Earth-Moon system with a satellite of negligible mass placed at the $L_4$ point:

In the rotating Earth-Moon reference frame, I wonder what prevents the satellite from falling towards point $b$ in this diagram. What are all the forces involved to put the satellite in equilibrium position? Based on my understanding, I think there is a centrifugal force from point b that has the same magnitude and is in opposite direction of gravitational force (yellow arrow). Is that right? The problem with my explanation is that it doesn't explain why the satellite should be positioned such that it is in equal distance away from both Earth and Moon.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

Yes, OP is right: The Earth-Moon reference frame is rotating, so besides the gravitational forces, there is also a centrifugal force acting on the satellite. (If the satellite is moving, there is also a Coriolis force.) The fact that the Earth-Moon-$L_4$ is an equilateral triangle is shown in my Phys.SE answer here.

Improve this answer
$\endgroup$
1
  • $\begingroup$ I updated the answer. $\endgroup$
    – Qmechanic ♦
    Commented Jun 16 at 6:13

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.