Feynman Lecture 33: Are Fresnel coefficients real?

Question

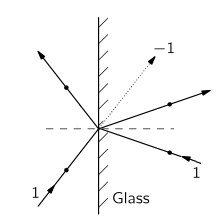
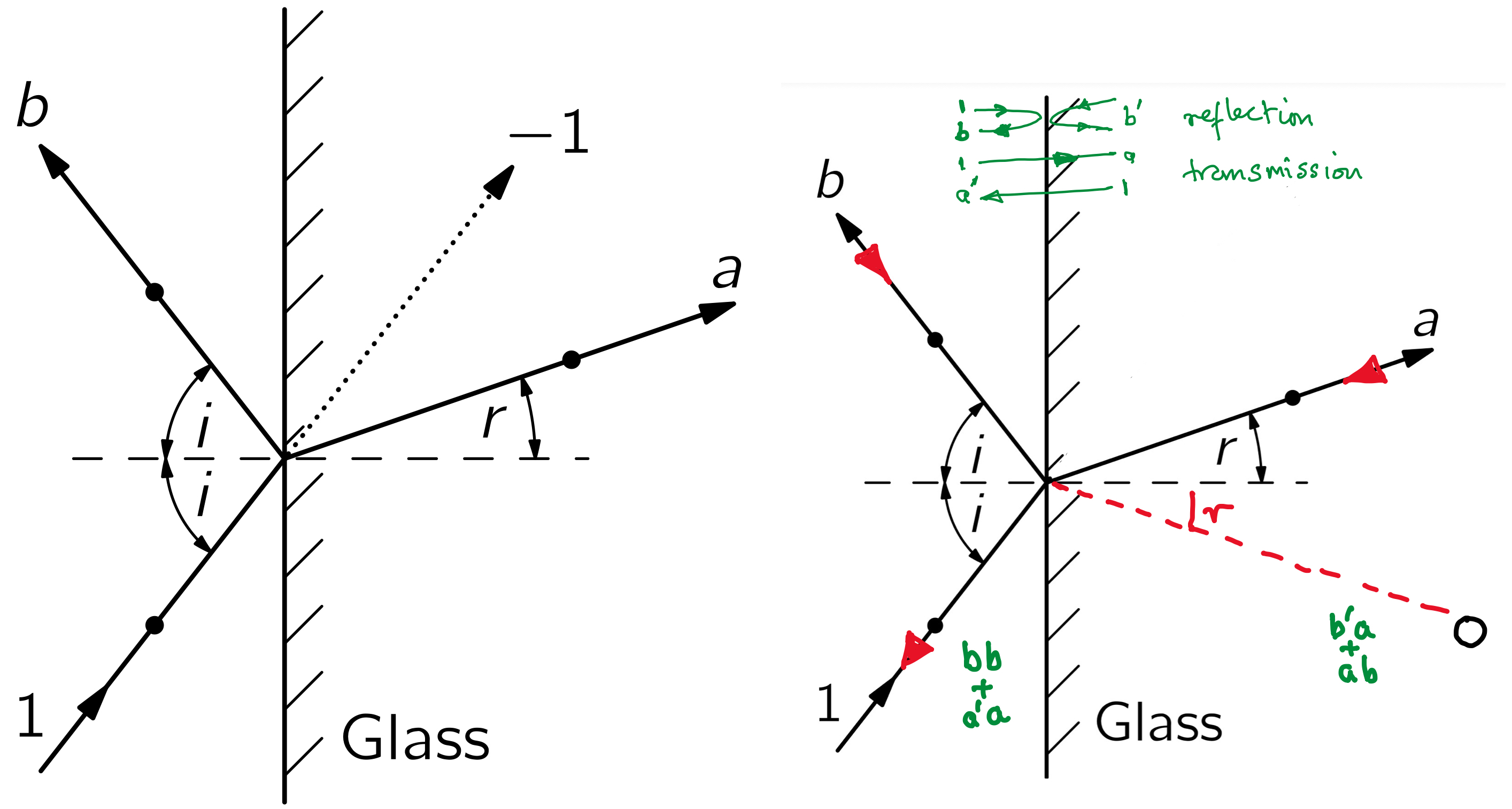
In the Section 33-6 Feynman says:

It is possible to go on with arguments of this nature and deduce that $b$ is real. To prove this, one must consider a case where light is coming from both sides of the glass surface at the same time, a situation not easy to arrange experimentally, but fun to analyze theoretically. If we analyze this general case, we can prove that $b$ must be real...

According to my previous question the case when the light goes from glass to air is not symmetrical. And both outgoing rays are a mix of some reflected and some refracted energy, so a ray will not be a pure $b$ anymore.

So what does Feynman mean by using 2 rays? How to prove that $b$ is real?

Answer 1

So what does Feynman mean by using 2 rays?

Feynman writes:

one must consider a case where light is coming from both sides of the glass surface at the same time, a situation not easy to arrange experimentally, but fun to analyze theoretically

and what he is asking you to do is to consider the reversal of all the light rays thus resulting in two waves incident on the interface.
This is shown in the diagram below.

With light going from glass to air you must replace $a$ and $b$ with $a'$ and $b'$.

Doing this produces the Stokes' relations $1=b^2+a'a$ and $0=ab+b'a \Rightarrow b=-b'$

---

$b=-b'$ shows that there is a phase difference of $\pi$ between waves travelling in air and reflected from the glass and those waves travelling in glass and reflected from the air.

$b$ can be made real by writing $b=|b|$ and $b'=|b|e^{\pm i\pi}$ which satisfies the Stokes' relation $b=-b'$.

What is not known at this point is which of the two reflections undergoes the $\pi$ phase change.

So taking the square root of Feynman's equation (33.6) $|b|^2 = \dfrac {\sin^2(i-r)}{\sin^2(i+r)}$ yields $b =\pm \dfrac {\sin(i-r)}{\sin(i+r)}$