I'm having trouble understanding the physical basis for the UV catastrophe in blackbody radiation graphs.
Is the source of the drop off past UV because the electrons in a blackbody substance can't emit photons with frequencies much greater than those in the UV range?
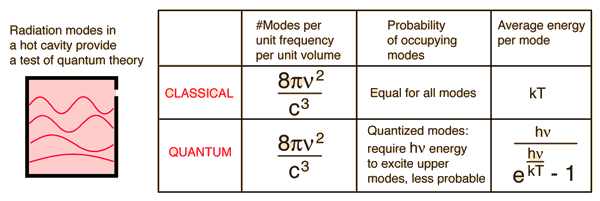
Firstly I want to clarify something, the "UV catastrophe" is an historical concept refering to the fact that previous theoretical developments of electromagnetism (before the rise of quantum mechanics and Planck's law) lead to a problematic divergence of the Rayleigh-Jeans law at high frequencies.
Indeed, the Rayleigh-Jeans law - which was derived from classical physics - states that a black body of temperature $T$ radiates at a frequency $\nu$ with the following radiance:
$B_{\nu} = \dfrac{2 \nu ^ 2 k T}{c ^2}$
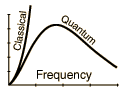
Where $k$ is the Stefan-Boltzmann constant and $c$ the speed of light. Here you should notice that this expression of $B_{\nu}$ diverges when $\nu \rightarrow +\infty$ (in other terms when we approach the "UV side" of the light spectrum). This is the reason why the expression "UV catastrophe" was coined: because classical physics could not correctly describe a black body at high frequency without having the radiance $B_{\nu}$ going to $+\infty$, which is of course not acceptable.
This is where quantum mechanics intervene.
Planck's non-classical law fixed this problem by using a new paradigm in which photons are quantified.
Thus, you should not interpret the "UV catastrophe" as some physical concept that prevents a black body from emitting UV photons. This is a misconception. The "UV catastrophe" is a mathematical artifact from an old theory, which has been fixed by Planck's law. There is no physical basis to understand about the "UV catastrophe", because it does not refer to anything real.
All you have to understand is that photons are described by the non-classical Bose-Einstein statistics, and as a consequence their distribution is characterized by Planck's law.
Hence there is no such thing as a systematic "drop off past UV". Actually, the possibility for a black body to emit UV photons entirely depends on its temperature $T$. Have a look at this figure:
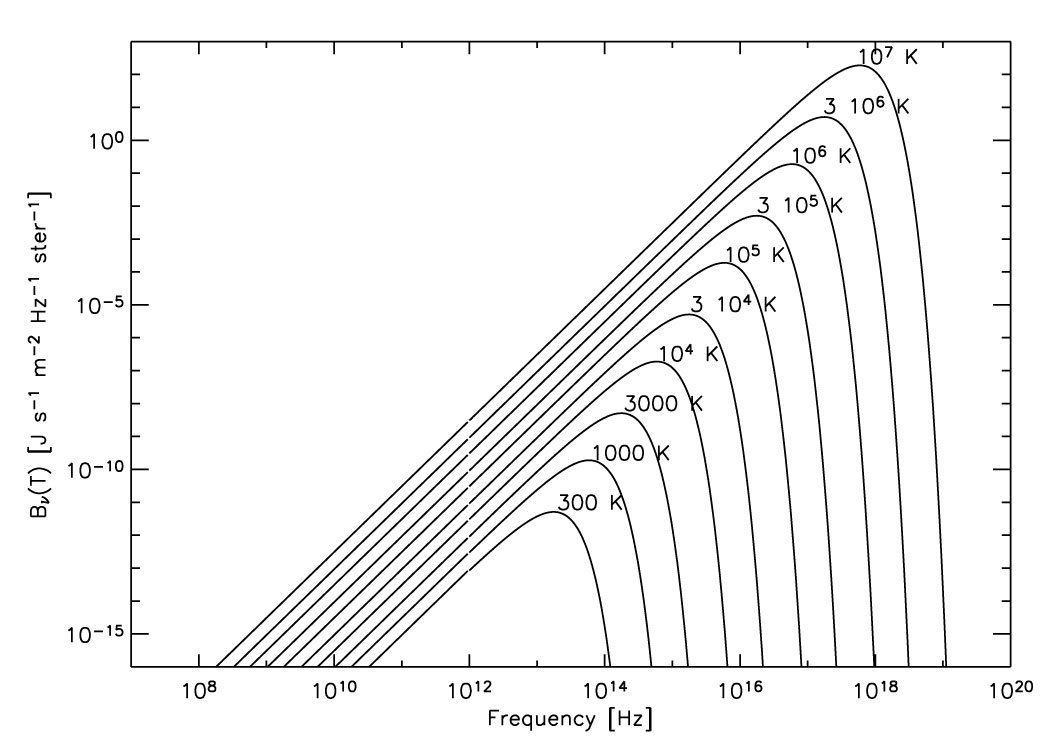
The frequency of a UV photon lies around $10^{15}~\mathrm{Hz}$. You can see that for temperatures $T>3000~\mathrm{K}$, UV photons are actually emitted in large numbers by a black body.
Note: using Wien's displacement law you could even determine the temperature for which UV photons are the main component of the light emitted by a black body.
Here is the difference between the classical and the need for the quantum hypothesis, because data follow the quantum curve
The approximation is in modelling all the radiation sources in matter as small oscillators, that is the analogy. As they are not really that ordered, due to peculiarities of individual atoms and molecules, the black body curve is an approximate one.
Is the source of the drop off past UV because the electrons in a blackbody substance can't emit photons with frequencies much greater than those in the UV range?
Note that the number modes per unit frequency per unit volume are the same in the classical approximation and the quantum. It is the probability of occupying the modes that changes the functional form from classical, which rises with frequency, to the quantized mode which has an exponential fall off with frequency, thus avoiding for a given temperature the infinity at high frequency. This agreed with experiments, the observation was that the higher frequencies were limited according to the formula fit, more or less for real materials.
At the microscopic level there are vibrational and rotational modes in solids, which emit in the infrared region for room temperature, with a very small probability for high energy photons. The emitted photons will scatter elastically and inelastically until reaching the surface and exiting. Some with the correct energies will be absorbed and re-emitted. There are phase transitions to liquid and gas for high temperatures, which allow higher kinetic energies and thus higher energy interactions.
In the plasma of the sun, the approximation of black body radiation is just that, an approximation.
Solar irradiance spectrum above atmosphere and at surface. Extreme UV and X-rays are produced (at left of wavelength range shown) but comprise very small amounts of the Sun's total output power.
The best fit to the black body curve prediction comes from the cosmic microwave background radiation.
Graph of cosmic microwave background spectrum measured by the FIRAS instrument on the COBE, the most precisely measured black body spectrum in nature. The error bars are too small to be seen even in an enlarged image, and it is impossible to distinguish the observed data from the theoretical curve.
