Short answer: They are different.
Long answer: There are a number of subtleties involving horizons in cosmology. I refer anyone who is interested in the details to a paper by Davis and Lineweaver, which I have referenced many times on this site. Here I'll only refer to Figure 1 of that paper, shown below. All three frames are the same, with the lower two being nonlinear but convenient rescalings of the "normal" one on top. (If you are familiar with spacetime diagrams, the bottom frame is conformally related to the top, so light travels on straight lines, which are at $45^\circ$ in the bottom pane. In any case, the rescalings never alter the structure of which regions encompass/border which others.)
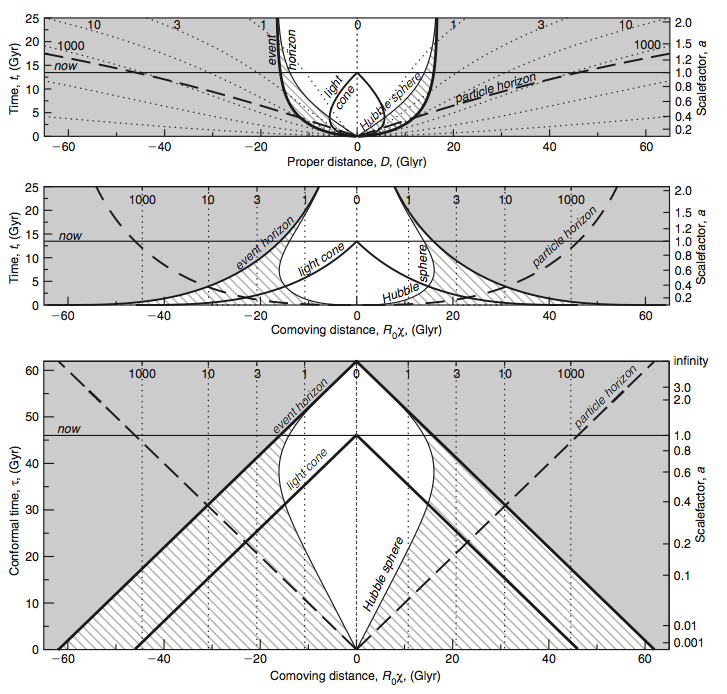
(Diagrams correspond to concordance cosmology of $\Omega_\mathrm{m} = 0.3$, $\Omega_\Lambda = 0.7$, and $H_0 = 70\ (\mathrm{km}/\mathrm{s})/\mathrm{Mpc})$.)
There are four different surfaces of interest. The simplest one to define is the Hubble sphere, which is where the recession velocity given by Hubble's Law is equal to the speed of light. However, the Hubble sphere doesn't mean much at all, despite its enigmatic definition. In general relativity, there's nothing too unusual about proper distances growing faster than $c$.
Our past light cone is also marked. This demarcates the region of the universe capable of influencing us today. That is, events within and on the light cone, and only those events, can originate a photon that we receive right now. Note that you can see beyond the Hubble sphere - there are events outside the Hubble sphere still within our past light cone. At the same time, there are things within the Hubble sphere that have not yet come into view. Thus neither the light cone nor the Hubble sphere is fully contained within the other.
You might also ask what locations in spacetime will we be able to receive information from, given that we wait long enough. This limit of the past light cone as we move its apex toward future infinity is the event horizon. This includes all of the light cone and its interior, since anything seen today can certainly be seen some time between today and future infinity. It also includes all of the Hubble sphere and its interior, since if something is not receding faster than light right now, photons it emits will eventually get here.
Finally, there's the particle horizon. Be careful, as different authors use slightly different definitions. Here, think of it as a function of time. At a particular time, the particle horizon is the distance - at that time - to the furthest object that can be seen. Note that in our universe, with its accelerated expansion, an object can right now be outside our event horizon - thus not capable of ever influencing us any more - while still inside our particle horizon, since we can see an older version of it with light that was emitted back when it was still inside our event horizon.
In summary, "what we can see" and "what is presently receding faster than light" are two different sets of things. Also, I would advise against trying to apply special relativistic Doppler effects to GR. The main purpose of the paper is in displaying all the ways this goes wrong. Have a look at Appendix B to see a list of examples were scientists got confused on this point.

