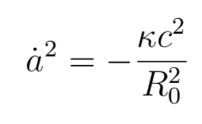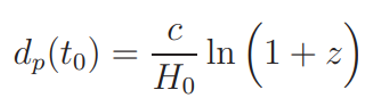How could proper distance today be infinity in a curvature only Universe when the age is finite?

Question

So for a curvature only universe, the Friedmann equation becomes

and we get the solution $a(t) = t/to$, and $to = 1/Ho$.

If we calculate the proper distance today we will get

As $z-> infinity$, the proper distance today also approaches infinity.

Typical textbook would say something like, in this universe we could see things infinitely far away.

I understand that when a galaxy emits a light, as the light travels towards us that galaxy is moving away from us, thus the proper distance to that galaxy today would be larger than the distance to that galaxy when that photon was emitted. But how could that galaxy's proper distance be infinity today? The age of this universe is $1/Ho$, finite, how could that galaxy have travelled to infinitely far from us by today?

Answer 1

When $a(t) = t/t_0$ and $κ=-1/t_0^2$, the FLRW metric

$$ds^2 = dt^2 - a(t)^2 \left( \frac{dr^2}{1-κr^2} + r^2 dΩ^2 \right)$$

with the coordinate substitution

$$\begin{eqnarray} T &=& t\sqrt{1-kr^2} \\ R &=& r\,a(t) \end{eqnarray}$$

becomes

$$ds^2 = dT^2 - dR^2 - R^2 dΩ^2$$

which shows that it's just Minkowski space in odd coordinates. This shouldn't be surprising since the stress-energy tensor is identically zero.

If you fix $t$ and $Ω$, and look at the locus of points at all $r$ in the Minkowski $(T,R)$ coordinates, you'll see that these points form a hyperbola which fits entirely inside the future light cone of the origin ($T=R=0$), even though it's of infinite length. Therefore, it's possible for a test particle starting at the origin (the big bang) to reach any $r$ at a later time without exceeding the speed of light.