2
$\begingroup$

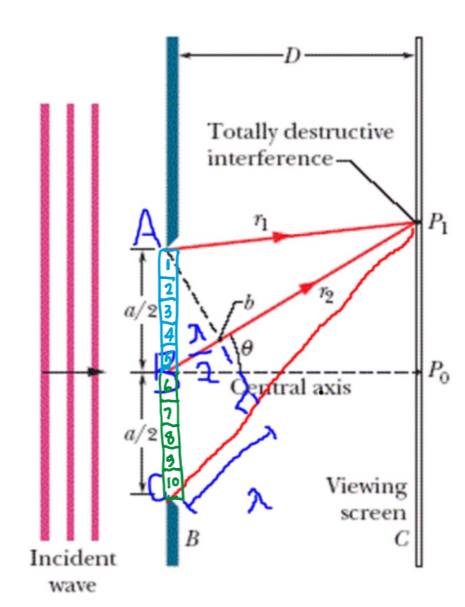
enter image description here

I understand that the Huygens principle states that wavefront can be treated as infinite point sources of circular waves, and that the first destructive interference requires the phase difference between A and C to be λ, so that the half point B can form destructive interference with A. However, if point B interfere destructively with A, then wouldn't that mean the C has no other waves to interfere destructively with? Thus, there is always a single ray of light that will arrive at the screen without being interfered.

Essentially, to which point on the incident wave does the light ray from point C interfere destructively with?

Please explain. Thank you.

Improve this question
$\endgroup$
1
  • $\begingroup$ Interference modifies the amplitude at a given point, say by summing together positive and negative waves at that point. It does not 'destroy' any of the waves. The waves pass through each other unaltered. $\endgroup$
    – user45664
    Commented Jun 2, 2018 at 16:52

3 Answers 3

Reset to default
2
$\begingroup$

Assume that there are ten secondary sources as shown below with the waves from source $1$ overlapping with that from source $6$, $2$ with $7$ etc with the path difference being $\frac \lambda 2$.

enter image description here

Now imagine that instead of ten secondary sources you increase that number to one thousand, one million, one billion etc.

Improve this answer
$\endgroup$
1
$\begingroup$

It's not quite clear what your assumptions are, but it appears that you might be assuming that only two "rays" can interfere at a time at any point. If so, that's not correct. Destructive interference doesn't destroy the interfering waves. Instead, interference involves the superposition of all the "rays" incident at a point. I use the term "rays" here because of the way you made your drawing with waves coming into the aperture and rays leaving the aperture.

If, instead, you draw concentric circles around A having the same radial spacing as your incident wavelength, and do the same for B and C, then you might get a better idea of how Huygens principle works. Anyplace that circles from A, B, and C all overlap, you'll get constructive interference. Destructive interference (and interference in general) is more difficult to illustrate graphically, because it requires taking both phase and amplitude into account. Phase change is proportional to the distance from the point source; and the amplitude contributed by each point source drops in proportion to $1/r$, where $r$ is the distance from the point source. Note that this is different from the way that intensity drops, which is in proportion to $1/r^2$. The only way I know to do the interference calculation is to use calculus: to do an integral adding all the phase and amplitude contributions from the wavefront, across all the points in the aperture.

If this doesn't help, please try to clarify your question.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Thanks for the response! So are you saying that the amplitude of the light ray drops as it travels a greater distance, therefore, if hypothetically there are two light rays travel from A and B to the screen with a phase difference of λ/2, the resultant wave due to the interference of the two wave is actually bigger than 0, as the ray from A will have a greater amplitude than the ray from B? $\endgroup$
    – lww
    Commented Jun 2, 2018 at 18:52
  • $\begingroup$ Yes, exactly. The resultant amplitude is the sum of the complex amplitudes of A and B. $\endgroup$
    – S. McGrew
    Commented Jun 2, 2018 at 21:22
  • $\begingroup$ So does it also implies that for a double slit interference from two point sources, the dark fringe due to the λ/2 phase difference does not have a resultant amplitude of 0? Given that one light ray travels a longer distance, thus, a smaller amplitude. Therefore, it is only 'dark' relatively. $\endgroup$
    – lww
    Commented Jun 2, 2018 at 23:00
  • $\begingroup$ You are right. The fringe can only be "perfectly" dark if all the complex amplitudes there add up to exactly zero. $\endgroup$
    – S. McGrew
    Commented Jun 2, 2018 at 23:51
0
$\begingroup$

The picture is a simplification using only 3 point sources when in fact they are infinite. The amount of light reaching P1 will be the integral of the phases reaching P1 from across the entire line segment AC. It will probably be something like the integral of cosine from -pi to pi, which is 0.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.