I have a question relating to this diagram:
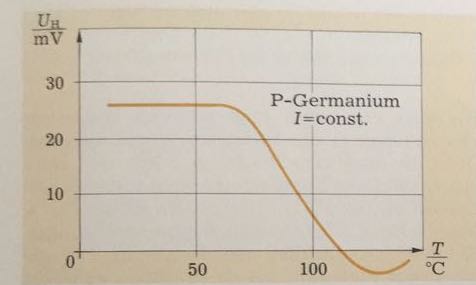
It shows the relationship between Temperature and Hall-Voltage of a P-doped Germanium Plate. The electron density n is constant from 10°C to 50°C, that's why the voltage is constant.
But Why exactly does the Voltage(Hall) decrease with higher temperatures and why is it getting negative (below 0) and increasing again. My understanding of it is somehow vague and would appreciate a detailed response.
