There's roughly three things going on here.
For one, the increasing-$(n+\ell)$ rule is true but it is incomplete: it correctly predicts, say, that the $3d$ shell gets filled after the $4s$ one, but it is completely silent on whether the $3p$ shell gets filled before or after the $4s$ shell (since both have $n+\ell=4$).
Secondly, the assignment of energy levels in hydrogen as $E_n=-\frac{1}{n^2}|E_1|$ is a very good approximation but it is nevertheless an approximation which needs to be refined if you want to describe atomic physics correctly.
Finally, you are correct in your assertion that
we can't use anymore the hydrogen atom as an example
when there is more than one electron in the atom, because the electron-electron electrostatic interaction (most strongly in the form of shielding of the nuclear charge) is a key factor in determining atomic structure.
OK, so let's start from the top. The key principle that your question describes is known as the Aufbau principle (also discussed here), which specifies that shells should be filled in order of increasing $n+\ell$ and that, within each $n+\ell=\mathrm{const}$ level, they should be filled with increasing $n$:
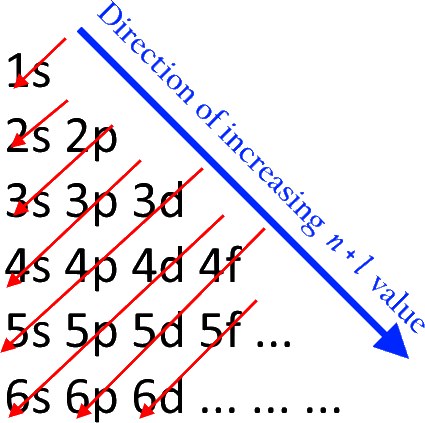
Image source
The electron configurations of atoms are dominantly governed by the electrostatic interaction: between the electrons and the nucleus, as in the hydrogen atom, but also between the electrons themselves. If you want to do this in full it is a crazy-complicated problem (broadly classed as electron-electron correlation) but the greatest contribution, as mentioned above, is shielding:
- A $2p$ electron in boron, for example, is essentially outside the $1s$ cloud, so it experiences an effective nuclear charge much closer to $Z_\mathrm{eff}=3$ than to $Z=5$.
- By contrast, the $2s$ cloud spends some time further away, but it also ventures closer to the nucleus, so it experiences the full brunt of the nuclear charge over more of its domain, which brings its energy down compared to the $2p$ wavefunction, even if a hydrogenic approximation would suggest that they'd be degenerate.
(For more details, see my answer to Why are the higher angular momentum states of a hydrogen atom closer to the nucleus?, particularly the second-to-last paragraph.)
Also, it's important to note that the Aufbau principle is not universally accurate: it is a good guide, but it does fail in cases where the complexities of many-electron atoms overwhelm it. (As one rough example, the ground-state configuration of copper is $[\mathrm{Ar}]3d^{10}4s^1$ rather than $[\mathrm{Ar}]3d^{9}4s^2$ due to the benefits of having a full $d$ shell.) And similarly, if you're going down the Aufbau-principle path, it's important to mention Hund's rules as important principles in determining atomic structure.
Thus far for multi-electron atoms. However, even in hydrogen, the assignment of energies as $E_{n,\ell}=-\frac{1}{n^2}|E_1|$ with complete degeneracy in $\ell$ is only an approximation: it is a good approximation, but the degeneracy is lifted by the fine structure contributions, which are essentially relativistic corrections (both via kinetic-energy modifications and via magnetic effects, including spin-orbit interactions), which are about $\alpha^2\approx 5\times 10^{-5}$ times smaller than the electrostatic dynamics (where $\alpha=⟨v⟩/c$ is the fine-structure constant, for $⟨v⟩$ the mean velocity in hydrogen eigenstates).
Since it's been mentioned in the other answer, there is also an additional hyperfine structure, which results from the interaction of the electron's orbital and spin magnetic dipole moments with the intrinsic magnetic dipole moment of the nucleus. For hydrogen in the ground state, this is responsible for the 21cm line at about 5.9 µeV (as compared to the fine-structure contributions at about 0.5 meV, a hundred times larger). Hyperfine structure, as the name indicates, is essentially irrelevant unless you're actively looking for it; as an example, any temperatures above 60 millikelvins will completely overwhelm this splitting.

