I am unable to understand resonance currently, how could we determine what resonance is in laymen terms?
$\begingroup$
$\endgroup$
3
-
4$\begingroup$ I'm voting to close this question as off-topic because "I am unable to understand resonance currently" doesn't relieve you of the obligation to show some, even a little, effort at research and narrowing the question down to some more or less specific that isn't clear to you. $\endgroup$– Alfred CentauriCommented Nov 24, 2017 at 1:11
-
$\begingroup$ You could read through this hyperphysics.phy-astr.gsu.edu/hbase/Sound/rescon.html#c1 and then follow the advice in the comment above. $\endgroup$– user176049Commented Nov 24, 2017 at 1:35
-
$\begingroup$ I appreciate the honesty and will put more effort into my research $\endgroup$– BrandonCommented Nov 24, 2017 at 2:21
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
I will try to explain using real life situation. Imagine you have a system which has a stable fixed point - a 'situation' when it is not moving (evolving, so to say) and is stable. E.g the neutral position for a pendulum. For small perturbations around this point it will oscillate and after a while the motion will die out and you will have it still again. Now instead of having a 'free' motion - you coerce a force upon the system. An example would be instead of holding the top of the rope on a pendulum fixed, instead you apply some wobbling to it - thus perturbating the poor still pendulum. Assume your force is oscillating (such as moving the rope left and right like on a swing). As mathematics shows - after a while the pendulum will follow your hand and oscillate at the same frequency. Like a pet following your 'lead'. If you shake the pendulum quite slowly - it will oscillate but it will only make small, bearly noticable swings. As you raise the frequency (in a way that you now shake it faster but with a constant, higher frequency), the pendulum will like it more and start swinging wilder. If you pick just the right frequency - it will go wild and have relatively large swings even if your wobbling is small. In such a scenario the system (your hand - the external constant oscillatory wobbling + pendulum) is in resonance - it is when your external force has the largest 'effect' on the pendulum so to say. If you then have stamina left and try to wobble it even faster you will notice a sudden drop in pendulum's vigor. It simply doesn't like being far from resonance.
In physics, a graph speaks a thousand words. On $x$ axis is the ratio of the frequency of your wobbling and the natural pendulum frequency $f_0$ - when it is freely oscillating. The sharp amplitude peak is called resonance - approximately when your frequency $f$ matches the natural frequency $f_0$. That's when things start to go wild.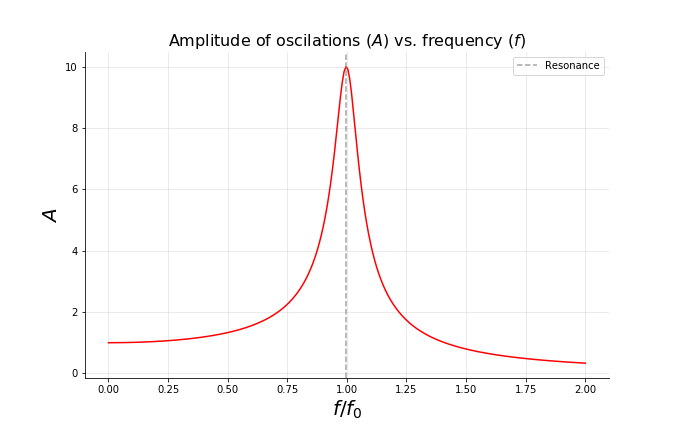 
This topic is far more general and resonance is playing a vital role in mechanics, electrodynamics (RLC circuits, basically every reciever), quantum physics (MRI), projecting bridges etc. :)
